SPATIAL  ENERGY   DISTRIBUTIONS:   TRANSVERSE   MODES

       In an ideal situation, energy would be stored throughout the entire lasing medium in a consistent manner and the entire lasing volume would be utilized. In reality the nature of the cavity gives rise to electromagnetic modes in which standing waves are set up not only in the longitudinal direction (i.e., the length of the cavity; these modes vary slightly in frequency) but also in the transverse direction. These represent alternative solutions to the wave equation which defines the beam (the complex equation that previously yielded the Gaussian solution). With energy stored in various areas of the lasing medium, the patterns formed are manifested in the output beam as well, which can assume shapes such as those shown in Figure 1.1, in which six common modes are photographed. As you peruse these photographs, note that they were shot at the same exposure, so that the relative brightness of the pattern indicates relative power of the output beam. In this case it is evident that some of the high-order modes, such as TEM₁₁, have much higher powers than that of the TEM₀₀ mode. Also, the camera was kept at the same position so that the relative size of the various modes can be compared (indicating the divergence, since the beam expands as it exits the laser).

     TEM₀₀, the Gaussian mode, is shown in the upper left corner. Two modes are labeled TEM₀₁: the first, commonly called the 01 donut mode, appears to have circular symmetry, and the other mode has rectangular (x/y axis) symmetry. The donut mode is often the superposition of TEM₀₁ and TEM₁₀ modes, both with rectangular symmetry, rather than a circularly symmetrical Laguerre–Gaussian mode. Several higher-order modes, all with rectangular symmetry, are shown in the bottom three photographs. All of the modes in Figure 1.1 are Hermite–Gaussian modes. In general, the mode designation (the subscripted numbers) may easily be

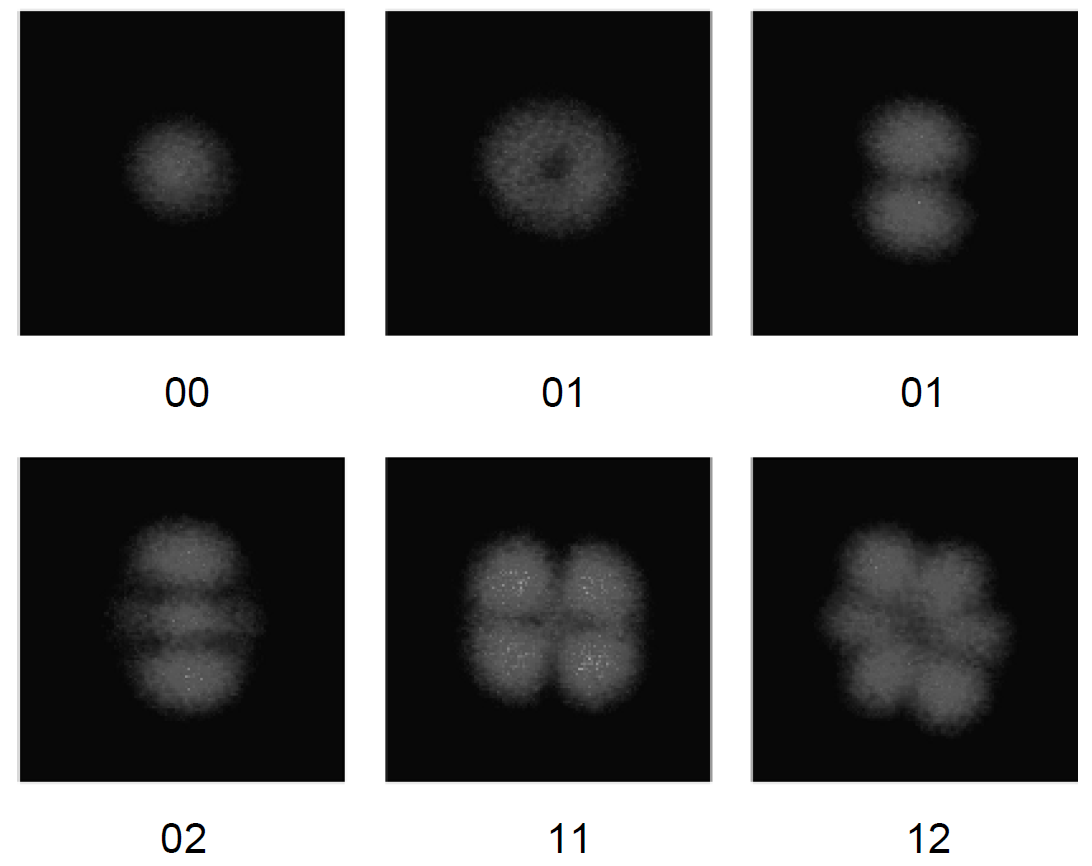
Figure1.1. Transverse electromagnetic modes.

Figure 1.2. Typical Laguerre–Gaussian mode (TEM₀₃).

determined for a Hermite–Gaussian mode by counting the number of dark lines in each direction so the mode designated as TEM₀₂ has no dark lines in the horizontal axis and two in the vertical axis. There is no preference to the order of the subscripted numbers, so TEM₀₂ is equivalent to TEM₂₀.

          Although Hermite–Gaussian modes (with rectangular symmetry) are favored in most operating lasers, other, circularly symmetrical modes are possible. These Laguerre–Gaussian modes are composed of concentric rings which are usually split into pie-shaped wedges like that shown in Figure 1.2. The mode in the figure is designated TEM₀₃. The first subscripted number for modes of this type is determined by counting the number of dark rings around the center of the pattern (in this example there are none), and the second number is determined by counting the number of lines bisecting the pattern (in this case, three).

        In many cases the highest power output from a particular laser can be obtained on a high-order mode in which the volume of the gain medium is utilized more effectively than is possible with the TEM₀₀ mode. This is evident by the fact that the TEM₁₁ mode and some other higher-order modes are considerably brighter than lower-order modes shown in Figure 1.1. This fact can be exploited (in reverse) to limit a laser to operation to TEM₀₀ mode.

مواضيع ذات صلة

Mirror reflections

The sensation of color

Physiochemistry of color vision

The mechanism of color vision

The chromaticity diagram

Measuring the color sensation

Color depends on intensity

Aberration

The ω,k four-vector

Moving sources

Anomalous refraction

The intensity of reflected light