
Laguerre Polynomial
 المؤلف:
Abramowitz, M. and Stegun, I. A.
المؤلف:
Abramowitz, M. and Stegun, I. A.
 المصدر:
"Orthogonal Polynomials." Ch. 22 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
المصدر:
"Orthogonal Polynomials." Ch. 22 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
 الجزء والصفحة:
...
الجزء والصفحة:
...
 4-8-2019
4-8-2019
 4451
4451
Laguerre Polynomial
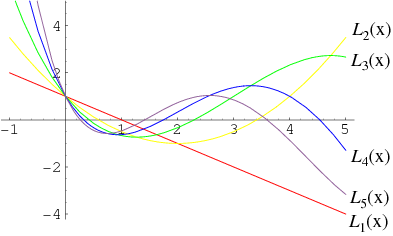
The Laguerre polynomials are solutions 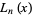 to the Laguerre differential equation with
to the Laguerre differential equation with 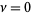 . They are illustrated above for
. They are illustrated above for ![x in [0,1]](http://mathworld.wolfram.com/images/equations/LaguerrePolynomial/Inline3.gif) and
and 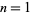 , 2, ..., 5, and implemented in the Wolfram Language as LaguerreL[n, x].
, 2, ..., 5, and implemented in the Wolfram Language as LaguerreL[n, x].
The first few Laguerre polynomials are
When ordered from smallest to largest powers and with the denominators factored out, the triangle of nonzero coefficients is 1; 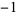 , 1; 2,
, 1; 2, 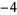 , 1;
, 1; 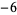 , 18,
, 18,  1; 24,
1; 24, 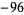 , ... (OEIS A021009). The leading denominators are 1,
, ... (OEIS A021009). The leading denominators are 1, 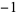 , 2,
, 2, 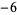 , 24,
, 24, 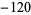 , 720,
, 720, 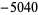 , 40320,
, 40320, 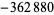 , 3628800, ... (OEIS A000142).
, 3628800, ... (OEIS A000142).
The Laguerre polynomials are given by the sum
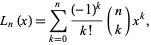 |
(5)
|
where 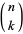 is a binomial coefficient.
is a binomial coefficient.
The Rodrigues representation for the Laguerre polynomials is
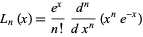 |
(6)
|
and the generating function for Laguerre polynomials is
A contour integral that is commonly taken as the definition of the Laguerre polynomial is given by
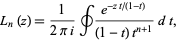 |
(9)
|
where the contour 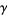 encloses the origin but not the point
encloses the origin but not the point 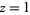 (Arfken 1985, pp. 416 and 722).
(Arfken 1985, pp. 416 and 722).
The Laguerre polynomials satisfy the recurrence relations
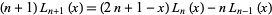 |
(10)
|
(Petkovšek et al. 1996) and
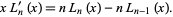 |
(11)
|
Solutions to the associated Laguerre differential equation with 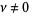 and
and 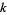 an integer are called associated Laguerre polynomials
an integer are called associated Laguerre polynomials 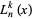 (Arfken 1985, p. 726) or, in older literature, Sonine polynomials (Sonine 1880, p. 41; Whittaker and Watson 1990, p. 352).
(Arfken 1985, p. 726) or, in older literature, Sonine polynomials (Sonine 1880, p. 41; Whittaker and Watson 1990, p. 352).
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Orthogonal Polynomials." Ch. 22 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 771-802, 1972.
Andrews, G. E.; Askey, R.; and Roy, R. "Laguerre Polynomials." §6.2 in Special Functions. Cambridge, England: Cambridge University Press, pp. 282-293, 1999.
Arfken, G. "Laguerre Functions." §13.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 721-731, 1985.
Chebyshev, P. L. "Sur le développement des fonctions à une seule variable." Bull. Ph.-Math., Acad. Imp. Sc. St. Pétersbourg 1, 193-200, 1859.
Chebyshev, P. L. Oeuvres, Vol. 1. New York: Chelsea, pp. 499-508, 1987.
Iyanaga, S. and Kawada, Y. (Eds.). "Laguerre Functions." Appendix A, Table 20.VI in Encyclopedic Dictionary of Mathematics. Cambridge, MA: MIT Press, p. 1481, 1980.
Koekoek, R. and Swarttouw, R. F. "Laguerre." §1.11 in The Askey-Scheme of Hypergeometric Orthogonal Polynomials and its  -Analogue. Delft, Netherlands: Technische Universiteit Delft, Faculty of Technical Mathematics and Informatics Report 98-17, pp. 47-49, 1998.
-Analogue. Delft, Netherlands: Technische Universiteit Delft, Faculty of Technical Mathematics and Informatics Report 98-17, pp. 47-49, 1998.
Laguerre, E. de. "Sur l'intégrale  ." Bull. Soc. math. France 7, 72-81, 1879. Reprinted in Oeuvres, Vol. 1. New York: Chelsea, pp. 428-437, 1971.
." Bull. Soc. math. France 7, 72-81, 1879. Reprinted in Oeuvres, Vol. 1. New York: Chelsea, pp. 428-437, 1971.
Petkovšek, M.; Wilf, H. S.; and Zeilberger, D. A=B. Wellesley, MA: A K Peters, pp. 61-62, 1996. http://www.cis.upenn.edu/~wilf/AeqB.html.
Roman, S. "The Laguerre Polynomials." §3.1 i The Umbral Calculus. New York: Academic Press, pp. 108-113, 1984.
Rota, G.-C.; Kahaner, D.; Odlyzko, A. "Laguerre Polynomials." §11 in "On the Foundations of Combinatorial Theory. VIII: Finite Operator Calculus." J. Math. Anal. Appl. 42, 684-760, 1973.
Sansone, G. "Expansions in Laguerre and Hermite Series." Ch. 4 in Orthogonal Functions, rev. English ed. New York: Dover, pp. 295-385, 1991.
Sloane, N. J. A. Sequences A000142/M1675 and A021009 in "The On-Line Encyclopedia of Integer Sequences."
Sonine, N. J. "Sur les fonctions cylindriques et le développement des fonctions continues en séries." Math. Ann. 16, 1-80, 1880.
Spanier, J. and Oldham, K. B. "The Laguerre Polynomials 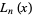 ." Ch. 23 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 209-216, 1987.
." Ch. 23 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 209-216, 1987.
Szegö, G. Orthogonal Polynomials, 4th ed. Providence, RI: Amer. Math. Soc., 1975.
Whittaker, E. T. and Watson, G. N. Ch. 16, Ex. 8 in A Course in Modern Analysis, 4th ed. Cambridge, England: Cambridge University Press, p. 352, 1990.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


