

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Manipulating the Vote
المؤلف:
W.D. Wallis
المصدر:
Mathematics in the Real World
الجزء والصفحة:
191-193
14-2-2016
1697
The term strategic voting means voting in a way that does not represent your actual preferences, in order to change the result of the election. We would call the resulting ballot insincere.
Suppose your favorite is candidate X. (We will call you an X supporter.) Then X would normally appear at the top of your preference list. But sometimes you can achieve X’s election by voting for another candidate in first place! This is most common in runoff situations; you can ensure that your candidate does not have to face a difficult opponent. The following example illustrates this.
Sample Problem 1.1 A runoff election has preference profile

Show that the supporters of C can change the result so that their candidate wins, by the two voters in the second column changing their ballots by demoting their candidate.
Solution. Initially the first-place votes are A–6, B–5,C–9, D–4, so runoff election will be between A and C, and A wins 15–9. The revised profile is

the first-place votes are A–6, B–7, C–7, D–4, so the runoff election is between B and C, and C wins 13–11.
Even when you cannot ensure victory for your favorite candidate, you may still be able to obtain a preferable result. For example, suppose you support candidate X; you think candidate Y is acceptable, but hate candidate Z. Even if insincere voting cannot ensure victory for candidate X, you may be able to swing the election to Y rather than Z.
Sample Problem 1.2 An election with four candidates and seven voters is to be decided by the Hare system. The preference profile is
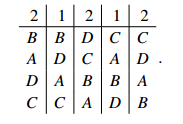
Show that one of the two voters with B,A,D,C can change the outcome to a more favorable one by insincere voting.
Solution. First consider the result of sincere voting. Initially A is eliminated, having no first-place votes:

The winner is C.
Now suppose one voter changes his ballot from B,A,D,C to D,A,B,C. The profile is
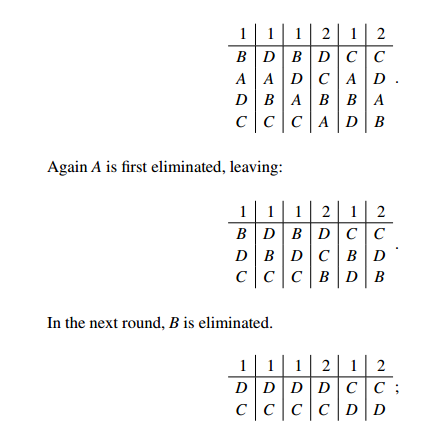
The winner is D. This is a preferable outcome for the voter who switched.
Your Turn. Consider a Hare system election with preference profile
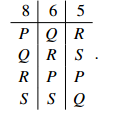
Show that P would win this election. Show that if one of the six supporters of Q changes her vote, she could ensure that R wins, even though a majority of voters still prefer Q to R.
Of course, you do not always know exactly how the votes will go. Strategic voting is usually based on assumptions about the election.
 الاكثر قراءة في الرياضيات التطبيقية
الاكثر قراءة في الرياضيات التطبيقية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















