

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Moritz Abraham Stern
المؤلف:
B Bergmann and M Epple
المصدر:
Jüdische Mathematiker in der deutschsprachigen akademischen Kultur
الجزء والصفحة:
...
3-11-2016
1082
Died: 30 January 1894 in Zurich, Switzerland
Moritz Abra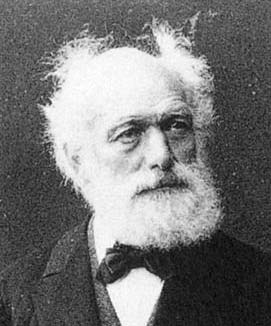 ham Stern's parents both came from old-established, well-off Jewish families in Frankfurt. His father Abraham Süsskind Stern, a merchant and bookseller, was a very scholarly man who [4] "dedicated his life to studying the Talmud". Moritz's mother, Vögele Eva Reiss, owned a silk shop which, as a young woman, she opened in order to support her parents and siblings after the family had lost all of its possessions in 1796, when a fire destroyed most of the Judengasse, the Jewish ghetto in Frankfurt. Moritz had two brothers, Jakob and Emanuel. Incidentally, Emanuel was the grandfather of Alice Frank (née Stern), Anne Frank's grandmother. Moritz's nephew Bernhard Stern recalls in [4] that Moritz's parents were "truly religious", and that they:-
ham Stern's parents both came from old-established, well-off Jewish families in Frankfurt. His father Abraham Süsskind Stern, a merchant and bookseller, was a very scholarly man who [4] "dedicated his life to studying the Talmud". Moritz's mother, Vögele Eva Reiss, owned a silk shop which, as a young woman, she opened in order to support her parents and siblings after the family had lost all of its possessions in 1796, when a fire destroyed most of the Judengasse, the Jewish ghetto in Frankfurt. Moritz had two brothers, Jakob and Emanuel. Incidentally, Emanuel was the grandfather of Alice Frank (née Stern), Anne Frank's grandmother. Moritz's nephew Bernhard Stern recalls in [4] that Moritz's parents were "truly religious", and that they:-
... took the raising of their sons very seriously, especially their son Moritz Abraham, who showed great intelligence from early on.
Moritz did not attend school but was educated at home, first by his father and later by private teachers, among them Michael Creizenach, who taught him mathematics. He had a particular talent for languages, learning Hebrew, Yiddish, Latin and Greek to perfection, as well as ancient oriental languages such as Chaldean and Syriac. At the age of 80 he began to learn Russian, which enabled him to read both Russian literature and Russian mathematical papers in the original.
His mother had hoped that he would become a Rabbi, but when Moritz began his studies at the University of Heidelberg in 1826, he mainly attended lectures on mathematics by Ferdinand Schweins. Following the suggestion of his friend, the mathematician Michel Reiss, he moved to Göttingen half a year later. There he was primarily taught by Carl Friedrich Gauss and Bernhard Friedrich Thibaut, but he also studied chemistry and philology. Gauss in particular had a big influence on Stern's mathematical taste as he introduced him to number theory, which became one of his main areas of interest.
Already in 1829 Stern received a doctorate for his thesis Observationum in fractiones continuas specimen on the theory of continued fractions. He was the first doctoral candidate examined by Gauss, who is said to have joked later on that he was more afraid of the viva than the examinee [1]. He habilitated at the University of Göttingen, becoming a Privatdozent there in 1830. This was an unpaid position to begin with; it was only eight years later that the university paid him an annual salary, initially 150 talers. For comparison purposes, we note that an ordinary professor earned more than ten times as much [2].
In 1848 Stern was finally appointed to an extraordinary professorship. This did not improve his financial situation, but it raised his profile in academia. The appointment was by no means a matter of course: while Jews were admitted to university studies, they were generally denied any academic positions in the German states of the 19th century. Stern was not the first Jew to become an extraordinary professor, but even making a Jew a Privatdozent would have caused outrage at many other universities. At the time, professors at German universities were held in extraordinarily high esteem and enjoyed many privileges. Appointing a Jew to a professorship would have been considered tantamount to sacrilege. As a result, many Jewish academics decided to be baptised into the Christian faith or to take up posts at universities abroad.
Stern however stayed in Germany and was determined to become a professor. His son Alfred later wrote about his father's ambitions [2]:-
But my father had set himself the goal of trying his luck as an academic teacher at the Georgia Augusta. He knew that it would be a thorny path. Up until then there had not been a single ordinary professor in Germany who was Jewish. When his friend, the solicitor Goldschmidt from Frankfurt, heard of his intention, he said: "You are as good as insane." But he felt bold and strong so as to chance what was unheard of.
Interestingly, Stern was by no means a devout Jew, but belonged to Liberal or Reform Judaism. He believed that many traditional Jewish practices could and should be adapted to particular circumstances of everyday life. In a letter to a friend he even said that [4]:-
What binds me to Judaism [...] is purely a filial sense of duty.
Nonetheless he saw himself as a protagonist of the Jewish emancipation, and believed that an appointment to a professorship would bring glory not only to himself but to the entire Jewish population [10].
He reached his goal in 1859, when he was appointed to an ordinary professorship. This made him the first non-baptised Jew ever to become a full professor at a German university. He was inaugurated at the same time as his former student Bernhard Riemann, who succeeded Lejeune Dirichlet. One reason for this appointment was the numerous petitions and recommendations that Stern's friends and colleagues, including the university's rector, had been sending to the government of the Kingdom of Hanover for the past 20 years. Moreover, the university feared that it would lose one of its most experienced lecturers otherwise [10].
During his 55 years at the University of Göttingen, Stern lectured on a wide variety of topics, including algebraic analysis, analytic geometry, differential and integral calculus, variational calculus, mechanics, popular astronomy and, of course, number theory. Stern is said to have been one of the most popular and also most gifted lecturers at the university [10]:-
Unlike Gauss, Stern was a very good teacher, even though he did not establish a mathematical school. He had thoroughly mastered the subjects he taught, and his lectures bore witness to that. [...] both colleagues and students spoke most highly of Stern's pedagogical talent.
However he could not compete with his former teacher Gauss when it came to research, which is why his merits are not always recognised. Ullrich writes in his biography of Riemann that [12]:-
Moritz Abraham Stern, Riemann's second academic teacher at Göttingen, is often termed a second-rate mathematician, sometimes even without noting that one is comparing him to Gauss. In any case, Riemann received a profound knowledge of the state of the art of analysis as taught in Germany at that time from Stern's lectures on calculus.
Stern married Bertha Simon (1823-1850) in 1844. The wedding took place in the village of Bockenheim (now part of Frankfurt) instead of his hometown, as the by-laws regulating the lives of Frankfurt's Jewish population permitted a maximum of 15 Jewish weddings per year. Bertha Stern died only six years later, after having given birth to their daughter Emma. When Emma died in 1884, Stern retired from his teaching post and moved to Bern, where his son Alfred (1846-1936) was a history professor at the university. Stern's chair at Göttingen was filled by Felix Klein.
Three years later Alfred was appointed to a professorship in history at the Polytechnic in Zurich. Upon arrival in Zurich, Moritz Stern was made an honorary member of the local Society of Natural Scientists. He soon became well known and respected among the Zurich mathematicians. He also had very good contacts with German mathematicians, most notably with Gotthold Eisenstein. Among his friends outside of mathematics, we mention the anatomist Jacob Henle, the author Berthold Auerbach, and the politician and judge Gabriel Riesser.
Stern remained extraordinarily healthy and engaged in mathematical and philological research until his death.
Nowadays Stern is primarily remembered for the fact that he was the first non-baptised Jewish university professor in Germany and not for his mathematical work. Even in his lifetime he was recognised more for his teaching than for his research. Nevertheless Stern published numerous papers in various German, French and Belgian journals, as well as in Acta Mathematica. In Crelle's Journal alone, 55 of his papers appeared between 1830 and 1890. His publications reflect his diverse mathematical interests, including work on number theory, the theory of continued fractions, the theory of series, the theory of Bernoulli's and Euler's numbers, and the theory of functions. Further publications considered problems in mechanics and astronomy, such as his books Darstellung der populären Astronomie (1834) and Himmelskunde (1846). Stern also translated Poisson's Lehrbuch der Mechanik (1835-36) and wrote his own textbookLehrbuch der algebraischen Analysis (1860). In addition, he also published biographies of Gauss and the Austrian mathematician Johannes von Gmunden, as well as several philological papers and articles on Reform Judaism.
For his paper Über die Auflösung der transcendenten Gleichungen (Crelle, 1841) he received a prize from the Danish Academy of Sciences. In the same year the Belgian Academy of Sciences awarded him a prize for his paper on quadratic remainders, Recherches sur la théorie des résidus quadratiques (Mémoires cournonnés par l'Academie des sciences de Belgique, 1841). Stern was made an honorary member of the Physics Society of Frankfurt in 1855. Four years later he became a member of the Royal Bavarian Academy of Sciences, and in 1862 he was elected a member of the Royal Göttingen Academy of Sciences.
One of Stern's papers that is of particular interest is Sur une assertion de Goldbach relative aux nombres impairs (Nouvelles annals de mathématiques, 1854). Christian Goldbach had conjectured in 1752 that every odd integer could be expressed in the form p + 2a2, where p is prime and a ≥ 0 is an integer. Stern found two exceptions to this: 5777 and 5993, called Stern numbers (see, for example, [7]). He also showed that the only primes that cannot be written as p + 2a2, where p is a smaller prime and a > 0 is an integer, are 17, 137, 227, 977, 1187, and 1493. These primes are known as Stern primes. Since 1 was considered a prime in Stern's lifetime, the list of Stern primes can be extended to include 2 and 3 as well.
In his paper Über eine zahlentheoretische Funktion (Crelle, 1858) Stern described the mathematics behind what is now called the Stern-Brocot tree. Every entry in the tree is found by taking the mediant (m1 + n1)/(m2 + n2) of two adjacent fractions m1/m2 and n1/n2, starting with the fractions 0/1 and 1/0 as the first parents. By applying the mediant function repeatedly, one obtains an infinite binary tree, in which every positive rational number appears exactly once and in its lowest terms. However Stern used continued fractions to describe the relations between the individual vertices of the tree rather than mediants, and he studied how mediants are related to the Euclidean algorithm.
Taking all entries in the first i levels of the tree together with the two initial parents 0/1 and 1/0 gives the Stern-Brocot sequence of order i. The algorithm used to construct these sequences is similar to that used to create Farey sequences; the differences being that for a Farey sequence of order n the two initial fractions are 0/1 and 1/1, and that for all fractions a/b in the sequence we need b ≤ n.
In 1860 the French clockmaker Achille Brocot (1817-1878) discovered the tree independently of Stern. He had more practical applications in mind than Stern though, as he constructed the tree in order to choose the ratio of gears in watches.
- F Rudio, Biography in Allgemeine Deutsche Biographie 54 (1908), 502-504.
http://www.deutsche-biographie.de/pnd117276936.html?anchor=adb
Books:
- B Bergmann and M Epple, Jüdische Mathematiker in der deutschsprachigen akademischen Kultur, Springer, Berlin, 2009.
- S B Guthery, A Motif of Mathematics. History and Application of the Mediant and the Farey Sequence, Docent Press, Boston, 2011.
- M Pressler, Treasures From the Attic: The Extraordinary Story of Anne Frank's Family (Weidenfeld & Nicolson, London, 2011) (Orion EBook).
- N Schmitz, Alfred Stern (1846-1936). Ein europäischer Historiker gegen den Strom der nationalen Geschichtsschreibung (PhD Thesis, University of Tromso, 2008).
Articles:
- E Berkovich, The Year of Mathematics and History Lessons. Jewish Mathematicians in the German-speaking Academic Culture (in Russian), Notes on Jewish History 10 (2008).
http://berkovich-zametki.com/2008/Zametki/Nomer10/Berkovich0.php - L Hodges, A Lesser-Known Goldbach Conjecture, Mathematics Magazine 66 (1993), 45-47.
- D Roegel, A reconstruction of Merritt's Brocot table (1947).
http://locomat.loria.fr/merritt1947/merritt1947doc2.pdf - F Rudio, Erinnerung an Moriz Abraham Stern, Vierteljahrsschrift der Naturf. Gesellschaft in Zürich 39 (1894), 130-143.
- N Schmitz, Moritz Abraham Stern (1807-1894). Den første jødiske ordinarius ved et tysk universitet og hans populærastronomiske verk.
http://septentrio.uit.no/index.php/nordlit/article/view/1859/1730 - M A Stern, Über eine zahlentheoretische Funktion, Journal für die reine und angewandte Mathematik 55 (1858), 193-220.
- P Ullrich, Riemann, Georg Friedrich Bernhard (Biography of Riemann).
http://www.encyclopedia.com/topic/Berhnard_Riemann.aspx#2
 الاكثر قراءة في 1800to1819
الاكثر قراءة في 1800to1819
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















