

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Pa ul Adrien Maurice Dirac
المؤلف:
Biography in Encyclopaedia Britannica
المصدر:
Biography in Encyclopaedia Britannica
الجزء والصفحة:
...
26-9-2017
889
Died: 20 October 1984 in Tallahassee, Florida, USA
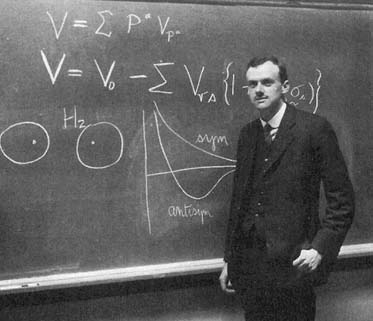
Paul Dirac's father was Charles Adrien Ladislas Dirac and his mother was Florence Hannah Holten. Charles Dirac was a Swiss citizen born in Monthey, in the Valais Canton of Switzerland, while his mother came from Cornwall in England. Charles had been educated at the University of Geneva, then came to England in around 1888 and taught French in Bristol. There he met Florence, whose father had moved to Bristol as Master Mariner on a Bristol ship, when she was working in the library there. Charles and Florence married in 1899 and they moved into a house in Bishopston, Bristol, which they named Monthey after the town of Charles's birth. By this time Charles was teaching French at the secondary school attached to the Merchant Venturers Technical College in Bristol.
Paul was one of three children, his older brother being Reginald Charles Felix Dirac and his younger sister being Beatrice Isabelle Marguerite Walla Dirac. Paul had a very strict family upbringing. His father insisted that only French be spoken at the dinner table and, as a result, Paul was the only one to eat with his father in the dining room. Paul's father was so strict with his sons that both were alienated and Paul was brought up in a somewhat unhappy home.
The first school which Paul attended was Bishop Primary school and already in this school his exceptional ability in mathematics became clear to his teachers. When he was twelve years old he entered secondary school, attending the secondary school where his father taught which was part of the Merchant Venturers Technical College. At about the time Paul entered this school, World War I began and this had a beneficial effect for Paul since the older boys in the school left for military service and the younger boys had more access to the science laboratories and other facilities. Paul himself wrote about his school years in [14]:-
The Merchant Venturers was an excellent school for science and modern languages. There was no Latin or Greek, something of which I was rather glad, because I did not appreciate the value of old cultures. I consider myself very lucky in having been able to attend the school. ... I was rushed through the lower forms, and was introduced at an especially early age to the basis of mathematics, physics and chemistry in the higher forms. In mathematics I was studying from books which mostly were ahead of the rest of the class. This rapid advancement was a great help to me in my latter career.
He completed his school education in 1918 and then studied electrical engineering at the University of Bristol. By this time the University had combined with the Merchant Venturers Technical College so Dirac remained in the same building as he had studied during his four years at secondary school. Although mathematics was his favourite subject he chose to study an engineering course at university since he thought that the only possible career for a mathematician was school teaching and he certainly wanted to avoid that profession. He obtained his degree in engineering in 1921 but following this, after an undistinguished summer job in an engineering works, he did not find a permanent position. By this time he was developing a real passion for mathematics but his attempts to study at Cambridge failed for rather strange reasons.
Taking the Cambridge scholarship examinations in June 1921 he was awarded a scholarship to study mathematics at St John's College, Cambridge, but it did not provide enough to support him. Additional support would have been expected from his local education authority, but he was refused support on the grounds that his father had not been a British citizen for long enough. Dirac was offered the chance to study mathematics at Bristol without paying fees and he did so being awarded first class honours in 1923. Following this he was awarded a grant to undertake research at Cambridge and he began his studies there in 1923.
Dirac had been hoping to have his research supervised by Ebenezer Cunningham, for by this time Dirac had become fascinated in the general theory of relativity and wanted to undertake research on this topic. Cunningham already had as many research students as he was prepared to take on and so Dirac was supervised by Ralph Fowler. The authors of [13] write:-
Fowler was then the leading theoretician in Cambridge, well versed in the quantum theory of atoms; his own research was mostly on statistical mechanics. He recognised in Dirac a student of unusual ability. Under his influence Dirac worked on some problems in statistical mechanics. Within six months of arriving in Cambridge he wrote two papers on these problems. No doubt Fowler aroused his interest in the quantum theory, and in May 1924 Dirac completed his first paper dealing with quantum problems. Four more papers were completed by November 1925.
Despite the obvious academic success Dirac enjoyed as a research student this was no easy time for him. His brother Reginald Dirac committed suicide during this period. No reason for the suicide seems to be known but Dirac's relations with his father, already strained, seemed almost to end completely after this which does suggest that Dirac felt that his father carried at least some responsibility. Already a person who had few friends, this personal tragedy had the effect of making him even more withdrawn.
Although he had already made an excellent start to his research career, even more impressive work was to follow. This was as a result of Dirac being given proofs of a paper by Heisenberg to read in the summer of 1925. The significance of the algebraic properties of Heisenberg's commutators struck Dirac when he was out for a walk in the country. He realised that Heisenberg's uncertainty principle was a statement of the noncommutativity of the quantum mechanical observables. He realised the analogy with Poisson brackets in Hamiltonian mechanics. Higgs writes in [14]:-
This similarity provided the clue which led him to formulate for the first time a mathematically consistent general theory of quantum mechanics in correspondence with Hamiltonian mechanics.
The ideas were laid out in Dirac's doctoral thesis Quantum mechanics for which he was awarded a Ph.D. in 1926. It is remarkable that Dirac had eleven papers in print before submitting his doctoral dissertation. Following the award of the degree he went to Copenhagen to work with Niels Bohr, moving on to Göttingen in February 1927 where he interacted with Robert Oppenheimer, Max Born, James Franck and the Russian Igor Tamm. Accepting an invitation from Ehrenfest, he spent a few weeks in Leiden on his way back to Cambridge. He was elected a Fellow of St John's College, Cambridge in 1927.
Dirac visited the Soviet Union in 1928. It was the first of many visits for he went again in 1929, 1930, 1932, 1933, 1935, 1936, 1937, 1957, 1965, and 1973. Also in 1928 he found a connection between relativity and quantum mechanics, his famous spin-1/2 Dirac equation. In 1929 he made his first visit to the United States, lecturing at the Universities of Wisconsin and Michigan. After the visit, along with Heisenberg, he crossed the Pacific and lectured in Japan. He returned via the trans-Siberian railway.
In 1930 Dirac published The principles of Quantum Mechanics and for this work he was awarded the Nobel Prize for Physics in 1933. De Facio, reviewing [4], says of this book:-
Dirac was not influenced by the feeding frenzy in experimental phenomenology of the time. This has given Dirac's book ... a lasting quality that few works can match.
The authors of [13] comment that the book:-
... reflects Dirac's very characteristic approach: abstract but simple, always selecting the important points and arguing with unbeatable logic.
The obituary [2] notes:-
His lectures at Cambridge were closely modelled on [The principles of Quantum Mechanics], and they conveyed to generations of students a powerful impression of the coherence and elegance of quantum theory. They constituted his principal contribution to education, for he took very few research students.
Also in 1930 Dirac was elected a Fellow of the Royal Society. This honour came on the first occasion that his name was put forward, in itself quite an unusual event which says much about the extremely high opinion that Dirac's fellow scientists had of him.
Dirac was appointed Lucasian professor of mathematics at the University of Cambridge in 1932, a post he held for 37 years. In 1933 he published a pioneering paper on Lagrangian quantum mechanics which became the foundation on which Feynman later built his ideas of the path integral. In the same year Dirac received the Nobel prize for physics which he shared with Schrödinger. It is an interesting comment on Dirac's nature that his first thought was to turn down the prize on the grounds that he hated publicity. However when it was pointed out to him that he would receive far more publicity if he turned down the prize, he accepted it. Another comment about this event is that Dirac was told that he could invite his parents to the award ceremony in Stockholm, but he chose to invite only his mother and not his father.
The academic year 1934-35 was important for Dirac both for personal and professional reasons. He visited the Institute for Advanced Study at Princeton and there he became friendly with Wigner. While Dirac was there Wigner's sister Margit, who lived in Budapest, visited her brother. This chance meeting led, in January 1937, to Dirac marrying Margit in London. Margit had been married before and had two children Judith and Gabriel Andrew from her first marriage. Both children adopted the name Dirac and Gabriel Andrew Dirac went on the became a famous pure mathematician, particularly contributing to graph theory, becoming professor of pure mathematics at the University of Aarhus in Denmark.
In 1937, the same year that he married, Dirac published his first paper on large numbers and cosmological matters. We comment further on his ideas on cosmology below. He published his famous paper on classical electron theory, which included mass renormalisation and radiative reaction in 1938. Dirac worked during World War II on uranium separation and nuclear weapons. In particular he acted as a consultant to a group in Birmingham working on atomic energy. This association led to Dirac being prevented by the British government from visiting the Soviet Union after the end of the war; he was not able to visit again until 1957.
We noted above that Dirac was elected a fellow of the Royal Society in 1930. He was awarded the Royal Society's Royal Medal in 1939 and the Society awarded him their Copley Medal in 1952:-
... in recognition of his remarkable contributions to relativistic dynamics of a particle in quantum mechanics.
In 1969 Dirac retired from the Lucasian chair of mathematics at Cambridge and went with his family to Florida in the United States. He held visiting appointments at the University of Miami and at Florida State University. Then, in 1971, Dirac was appointed professor of physics at Florida State University where he continued his research.
In 1973 and 1975 Dirac lectured in the Physical Engineering Institute in Leningrad. In these lectures he spoke about the problems of cosmology or, to be more precise, to the problems of non-dimensional combinations of world constants.
Although Dirac made vastly important contributions to physics, it is important to realise that he was always motivated by principles of mathematical beauty. Dirac unified the theories of quantum mechanics and relativity theory, but he also is remembered for his outstanding work on the magnetic monopole, fundamental length, antimatter, the d-function, bra-kets, etc.
There is a standard folklore of Dirac stories, mostly revolving around Dirac saying exactly what he meant and no more. Once when someone, making polite conversation at dinner, commented that it was windy, Dirac left the table and went to the door, looked out, returned to the table and replied that indeed it was windy. It has been said in jest that his spoken vocabulary consisted of "Yes", "No", and "I don't know". Certainly when Chandrasekhar was explaining his ideas to Dirac he continually interjected "yes" then explained to Chandrasekhar that "yes" did not mean that he agreed with what he was saying, only that he wished him to continue. He once said:-
I was taught at school never to start a sentence without knowing the end of it.
This may explain much about his conversation, and also about his beautifully written sentences in his books and papers.
Dirac received many honours for his work, some of which we have mentioned above. He refused to accept honorary degrees but he did accept honorary membership of academies and learned societies. The list of these is long but among them are USSR Academy of Sciences (1931), Indian Academy of Sciences (1939), Chinese Physical Society (1943), Royal Irish Academy (1944), Royal Society of Edinburgh (1946), Institut de France (1946), National Institute of Sciences of India (1947), American Physical Society (1948), National Academy of Sciences (1949), National Academy of Arts and Sciences (1950), Accademia delle Scienze di Torino (1951), Academia das Ciencias de Lisboa (1953), Pontifical Academy of Sciences, Vatican City (1958), Accademia Nazionale dei Lincei, Rome (1960), Royal Danish Academy of Sciences (1962), and Académie des Sciences Paris (1963). He was appointed to the Order of Merit in 1973.
A memorial meeting was held at the University of Cambridge on 19 April 1985 and the papers presented at this meeting were published in Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987). The papers [12], [15], [25], [30], [37], [39] and [40] come from this volume. Achuthan, reviewing the volume, writes:-
... we vividly see everywhere the brilliant imprints of Dirac, unifier of quantum mechanics and relativity theory. Each of the pieces not only is in praise of an exceptionally gifted intellect but also places on record how deeply and abidingly the human mind can delve into the realms of mathematical insight and modelling, keeping intact the spirit of beauty and clarity of a creative genius. Only a few Nobel laureates ever can compare as well with this giant of mathematical sciences in whose demise the world of original thinking certainly has lost one of the most precious souls retaining fortunately still the glory for others to sing and emulate for a long time to come.
In November 1995 of a plaque was unveiled in Westminster Abbey commemorating Paul Dirac. The volume [9] consists of lectures presented to the Royal Society on this occasion. The memorial address was presented by Stephen Hawking who was Dirac's successor in the Lucasian chair of mathematics at Cambridge which was also Newton's chair.
- Biography in Encyclopaedia Britannica.
http://www.britannica.com/EBchecked/topic/164795/PAM-Dirac
Books:
- B L Cline, The Questioners: Physicists and the Quantum Theory (1965).
- R H Dalitz (ed.), The collected works of P A M Dirac : 1924-1948 (Cambridge, 1995).
- H Kragh, Dirac : A Scientific Biography (Cambridge, 1991).
- B N Kursunoglu and E P Wigner (eds.), Reminiscences about a great physicist: Paul Adrien Maurice Dirac (Cambridge, 1987).
- B V Medvedev (ed.), Paul Dirac and 20th century physics (Russian) (Moscow, 1990).
- N Mukunda, The life and work of P A M Dirac, Recent developments in theoretical physics (Singapore, 1987).
- A Pais, M Jacob, D I Olive, and M F Atiyah, Paul Dirac : The man and his work (Cambridge, 1998).
Articles:
- A O Barut and A van der Merwe, Paul Dirac on his eightieth birthday, Found. Phys. 13 (2) (1983), 187-188.
- S D'Agostino, Mathematics and physics in the ideas of P A M Dirac, Rend. Accad. Naz. Sci. XL Mem. Sci. Fis. Natur. 9 (5) (1985), 287-290.
- R H Dalitz, A biographical sketch of the life of Professor P A M Dirac, OM, FRS, in J G Taylor (ed.), Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 3-28.
- R H Dalitz and R Peierls, Paul Adrien Maurice Dirac, 8 August 1902 - 20 October 1984, Biographical Memoirs of Fellows of the Royal Society of London 32 (1986), 139-180.
- P A M Dirac, A little 'prehistory', The Old Cothamian (1980), 9.
- C J Eliezer, Some reminiscences of Professor P A M Dirac, in J G Taylor (ed.), Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 58-60.
- V Ya Frenkel, Dirac in the USSR (Russian), Voprosy Istor. Estestvoznan. i Tekhn. (3) (1988), 91-100.
- V Ya Frenkel, Professor Dirac and Soviet physicists, Soviet Phys. Uspekhi 30 (9) (1987), 816-822.
- L Halpern, Paul Adrien Maurice Dirac (1902-1984), Found. Phys. 15 (3) (1985), 257-259.
- Harish-Chandra, My association with Professor Dirac, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 34-36.
- P Higgs, Paul Adrien Maurice Dirac, Yearbook of the Royal Society of Edinburgh Session 1985-86 (1987), 49-51.
- N Kemmer, What Paul Dirac meant in my life, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 37-42.
- A D Krisch, An experimenter's view of P A M Dirac, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 46-52.
- H Kragh, The genesis of Dirac's relativistic theory of electrons, Arch. Hist. Exact Sci. 24 (1) (1981), 31-67.
- S A Kursunoglu, Dirac in Coral Gables, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 9-28.
- J E Lannutti, Eulogy for Paul A M Dirac, 19 November 1984: 'Who was this guy?', in J G Taylor (ed.), Tributes to Paul Dirac (Bristol, 1987), 43-47.
- J E Lannutti, Recollections of Paul Dirac at Florida State University, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 29-33.
- P T Matthews, Dirac and the foundation of quantum mechanics, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 199-224.
- F A Medvedev, The delta-function of G L Giorgi and P A M Dirac (Russian), Studies in the history of physics and mechanics (Moscow, 1988), 78-88.
- B V Medvedev and D V Shirkov, P A M Dirac and the formation of the basic ideas of quantum field theory, Soviet Phys. Uspekhi 30 (9) (1987), 791-815.
- J Mehra, Dirac's contribution to the early development of quantum mechanics, in Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 63-75.
- L Michel, La vie et l'oeuvre de Paul Dirac, C. R. Acad. Sci. Sér. Gén. Vie Sci. 2 (6) (1985), 619-622.
- N Mott, Reminiscences of Paul Dirac, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 230-233.
- N Mukunda, Dirac, Harish-Chandra and the unitary representations of the Lorentz group, Current Sci. 65 (12) (1993), 936-940.
- N Mukunda, The life and work of P A M Dirac, in Recent developments in theoretical physics, Kottayam, 1986 (Singapore, 1987), 260-282.
- A Pais, Paul Dirac : aspects of his life and work, in Paul Dirac (Cambridge, 1998), 1-45.
- R Peierls, Dirac's way, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 43-45.
- R Peierls, Address to Dirac memorial meeting, Cambridge, in Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 35-37.
- J C Polkinghorne, At the feet of Dirac, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 227-229.
- J C Polkinghorne, A brief reminiscence of Dirac, in Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 33-34.
- S Shanmugadhasan, Dirac as research supervisor and other remembrances, in Tributes to Paul Dirac, Cambridge, 1985 (Bristol, 1987), 48-57.
- Ya A Smorodinskii, P A M Dirac (8.VII.1902 - 20.X.1984) (Russian), Paul Dirac and 20th century physics (Moscow, 1990), 5-14.
- H K Stanford, Dirac at the University of Miami, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 53-56.
- S Stenholm, P A M Dirac, 1902-1984 (Finnish), Arkhimedes 36 (4) (1984), 230-233.
- Vl P Vizgin, P A M Dirac and the problem of the interrelation between physics and mathematics (Russian), Studies in the history of physics and mechanics (Moscow, 1988), 88-106.
- G C Wick, Paul Adrien Maurice Dirac, Atti della Reale Accademia dei Lincei 78 (1985), 181-193.
- E P Wigner, Remembering Paul Dirac, in Reminiscences about a great physicist : Paul Adrien Maurice Dirac (Cambridge, 1987), 57-65.
 الاكثر قراءة في 1900to1904
الاكثر قراءة في 1900to1904
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















