

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Lebesgue Minimal Problem
المؤلف:
Ball, W. W. R. and Coxeter, H. S. M.
المصدر:
athematical Recreations and Essays, 13th ed. New York: Dover
الجزء والصفحة:
...
10-2-2020
1383

Find the plane lamina of least area 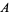 which is capable of covering any plane figure of unit generalized diameter. A unit circle is too small, but a hexagon circumscribed on the unit circle is larger than necessary. Pál (1920) showed that the hexagon can be reduced by cutting off two isosceles triangles on the corners of the hexagon which are tangent to the hexagon's incircle (Wells 1991; left figure above). Sprague subsequently demonstrated that an additional small curvilinear region could be removed (Wells 1991; right figure above). These constructions give upper bounds.
which is capable of covering any plane figure of unit generalized diameter. A unit circle is too small, but a hexagon circumscribed on the unit circle is larger than necessary. Pál (1920) showed that the hexagon can be reduced by cutting off two isosceles triangles on the corners of the hexagon which are tangent to the hexagon's incircle (Wells 1991; left figure above). Sprague subsequently demonstrated that an additional small curvilinear region could be removed (Wells 1991; right figure above). These constructions give upper bounds.
The hexagon having inradius 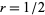 (giving a diameter of 1) has side length
(giving a diameter of 1) has side length
 |
(1) |
and the area of this hexagon is
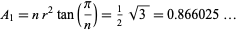 |
(2) |
(OEIS A010527).
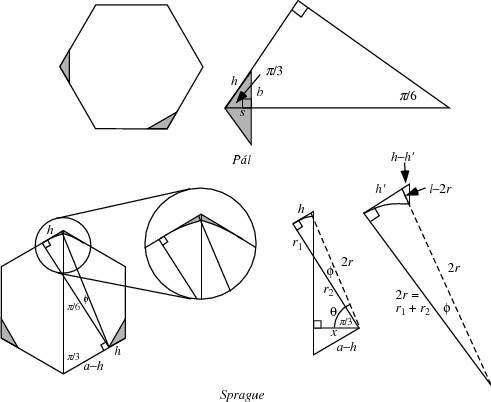
In the above figure, the sagitta is given by
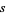 |
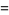 |
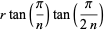 |
(3) |
 |
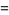 |
 |
(4) |
and the other distances by
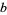 |
 |
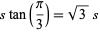 |
(5) |
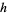 |
 |
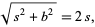 |
(6) |
so the area of one of the equilateral triangles removed in Pál's reduction is
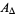 |
 |
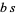 |
(7) |
 |
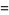 |
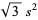 |
(8) |
 |
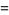 |
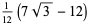 |
(9) |
 |
 |
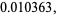 |
(10) |
so the area left after removing two of these triangles is
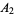 |
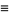 |
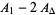 |
(11) |
 |
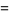 |
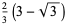 |
(12) |
 |
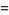 |
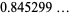 |
(13) |
(OEIS A093821).
Computing the area of the region removed in Sprague's construction is more involved. First, use similar triangles
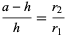 |
(14) |
together with 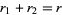 to obtain
to obtain
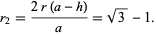 |
(15) |
Then
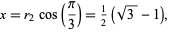 |
(16) |
and the angle  is given by
is given by
![theta=cos^(-1)(x/(2r))=cos^(-1)[1/2(sqrt(3)-1)],](http://mathworld.wolfram.com/images/equations/LebesgueMinimalProblem/NumberedEquation6.gif) |
(17) |
and the angle 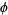 is just
is just
 |
(18) |
The distance 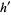 is
is
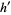 |
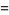 |
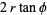 |
(19) |
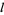 |
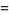 |
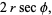 |
(20) |
and the area between the triangle and sector is
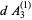 |
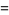 |
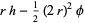 |
(21) |
 |
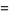 |
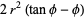 |
(22) |
 |
 |
 |
(23) |
 |
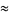 |
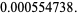 |
(24) |
The area of the small triangle is
 |
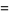 |
 |
(25) |
 |
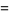 |
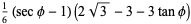 |
(26) |
 |
 |
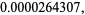 |
(27) |
so the total area remaining is
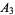 |
 |
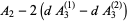 |
(28) |
 |
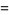 |
![-(109)/(121)-(82)/(121sqrt(3))+2/(121)sqrt(28634sqrt(3)-35139)-1/3pi+cos^(-1)[1/2(sqrt(3)-1)]](http://mathworld.wolfram.com/images/equations/LebesgueMinimalProblem/Inline72.gif) |
(29) |
 |
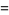 |
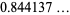 |
(30) |
(OEIS A093822).
It is also known that a lower bound for the area is given by
 |
(31) |
(Ogilvy 1990).
REFERENCES:
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 99, 1987.
Coxeter, H. S. M. "Lebesgue's Minimal Problem." Eureka 21, 13, 1958.
Grünbaum, B. "Borsuk's Problem and Related Questions." Proc. Sympos. Pure Math, Vol. 7. Providence, RI: Amer. Math. Soc., pp. 271-284, 1963.
Kakeya, S. "Some Problems on Maxima and Minima Regarding Ovals." Sci. Reports Tôhoku Imperial Univ., Ser. 1 (Math., Phys., Chem.) 6, 71-88, 1917.
Ogilvy, C. S. Tomorrow's Math: Unsolved Problems for the Amateur, 2nd ed. New York: Oxford University Press, 1972.
Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 142-144, 1990.
Pál, J. "Ueber ein elementares Variationsproblem." Det Kgl. Danske videnkabernes selskab, Math.-fys. meddelelser 3, Nr. 2, 1-35, 1920.
Sloane, N. J. A. Sequences A010527, A093821, and A093822 in "The On-Line Encyclopedia of Integer Sequences."
Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, p. 138, 1991.
Yaglom, I. M. and Boltyanskii, V. G. Convex Figures. New York: Holt, Rinehart, & Winston, pp. 18 and 100, 1961.