POINTS IN THE RL QUARTER-PLANE

In a circuit containing both resistance and inductance, the characteristics become two-dimensional. You can orient the resistance and reactance halflines perpendicular to each other to make a quarter-plane coordinate system, as shown in Fig. 1. Resistance is shown on the horizontal axis, and inductive reactance is plotted vertically, going upward. Each point on the R_L quarter-plane corresponds to a unique complexnumber impedance. Conversely, each complex-number impedance value corresponds to a unique point on the quarter-plane. Impedances on the R_L quarter-plane are written in the form R + jX_L, where R is the resistance in ohms, X_L is the inductive reactance in ohms, and j is the unit imaginary number, that is, the positive square root of -1. The value j in this application is known as the j operator. 
Suppose that you have a pure resistance, say, R = 5 ohms. Then the complex-number impedance is 5+ j0 and is at the point (5, 0) on the R_L quarter-plane. If you have a pure inductive reactance, such as X_L = 3 ohms, then the complex-number impedance is 0+ j3 and is at the point (0, 3) on the R_L quarter-plane. These points, and a few others, are shown in Fig. 1.

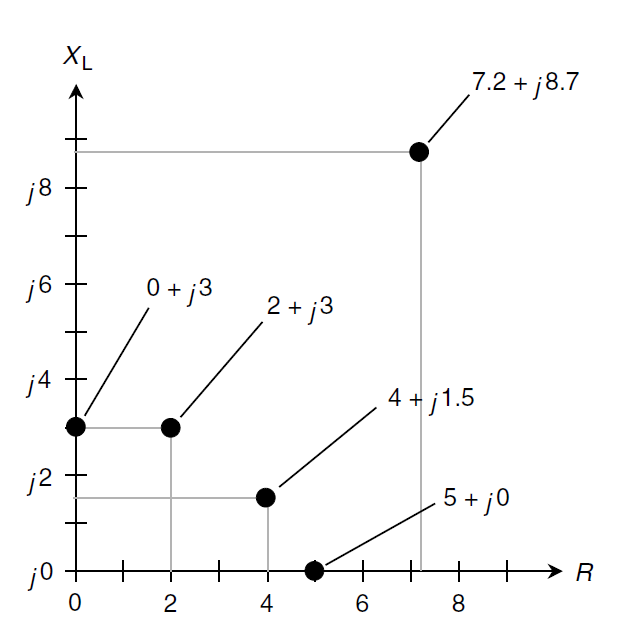
Fig. 1. The RL impedance quarter-plane showing five points for specific complex-number impedances.

In the real world, all coil inductors have some resistance because no wire is a perfect conductor. All resistors have at least a tiny bit of inductive reactance because they have wire leads at each end and they have a measurable physical length. There is no such thing as a mathematically perfect pure resistance like 5+ j0 or a mathematically perfect pure reactance like 0 +j3. Sometimes you can get close to such ideals, but absolutely pure resistances or reactances never exist (except occasionally in quiz and test problems, of course!).
In electronic circuits, resistance and inductive reactance are sometimes both incorporated deliberately. Then you get impedances values such as 2+ j3 or 4+ j1.5.
Remember that the values for X_L are reactances (expressed in ohms) and not inductances (which are expressed in henrys). Reactances vary with the frequency in an R_L circuit. Changing the frequency has the effect of making the points move in the R_L quarter-plane. They move vertically, going upward as the ac frequency increases and downward as the ac frequency decreases. If the ac frequency goes down to zero, thereby resulting in dc, the inductive reactance vanishes. Then X_L = 0, and the point is along the resistance axis of the R_L quarter-plane.

مواضيع ذات صلة

The dipole radiator

Radiation

Electromagnetism

ثنائيات الأشعة السينية آلية عملها

انفجار الأشعة السينية المنبعثة من 1–Sco X

أستاذ المناطيد (مناطيد استكشاف الاشعة السينية)

استعادة التلسكوب الخاص باستكشاف الاشعة السينية من وسط الصحراء: جاك صائد حيوانات الكنغر

التحليق عاليا المناطيد.. وكواشف الأشعة السينية.. وعملية الإطلاق

مطاردة الأشعة السينية بالمناطيد.. البداية

ما هي الأشعة السينية؟

أشعة سينية من الفضاء الخارجي

إنجاز ماكسويل العظيم