

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Lucas-Lehmer Test
المؤلف:
Knuth, D. E.
المصدر:
§4.5.4 in The Art of Computer Programming, Vol. 2: Seminumerical Algorithms. Reading, MA: Addison-Wesley, 1969.
الجزء والصفحة:
...
17-1-2021
1287
The Lucas-Lehmer test is an efficient deterministic primality test for determining if a Mersenne number 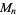 is prime. Since it is known that Mersenne numbers can only be prime for prime subscripts, attention can be restricted to Mersenne numbers of the form
is prime. Since it is known that Mersenne numbers can only be prime for prime subscripts, attention can be restricted to Mersenne numbers of the form  , where
, where 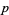 is an odd prime.
is an odd prime.
Consider the recurrence equation
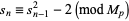 |
(1) |
with 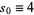 . For example, ignoring the congruence, the first few terms of this iteration are 4, 14, 194, 37634, 1416317954, ... (OEIS A003010).
. For example, ignoring the congruence, the first few terms of this iteration are 4, 14, 194, 37634, 1416317954, ... (OEIS A003010).
It turns out that 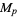 is prime iff
is prime iff 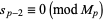 , and the value
, and the value 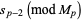 is called the Lucas-Lehmer residue for
is called the Lucas-Lehmer residue for  .
.
For example, the sequence obtained for 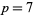 is given by 4, 14, 67, 42, 111, 0, so
is given by 4, 14, 67, 42, 111, 0, so 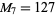 is prime.
is prime.
For prime  , the first few Lucas-Lehmer residues are 1, 0, 0, 0, 1736, 0, 0, 0, 6107895, 458738443, 0, 117093979072, ... (OEIS A095847).
, the first few Lucas-Lehmer residues are 1, 0, 0, 0, 1736, 0, 0, 0, 6107895, 458738443, 0, 117093979072, ... (OEIS A095847).
This test can also be extended to arbitrary integers. Prior to the work of Pratt (1975), the Lucas-Lehmer test had been regarded purely as a heuristic that worked a lot of the time (Knuth 1969). Pratt (1975) showed that Lehmer's primality heuristic could be made a nondeterministic procedure by applying it recursively to the factors of 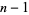 , resulting in a certification of primality that has come to be known as the Pratt certificate.
, resulting in a certification of primality that has come to be known as the Pratt certificate.
A generalized version of the Lucas-Lehmer test lets
 |
(2) |
with 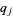 the distinct prime factors, and
the distinct prime factors, and 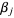 their respective powers. If there exists a Lucas sequence
their respective powers. If there exists a Lucas sequence 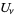 such that
such that
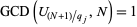 |
(3) |
for 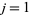 , ...,
, ..., 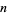 and
and
 |
(4) |
then 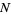 is a prime. This reduces to the conventional Lucas-Lehmer test for Mersenne numbers.
is a prime. This reduces to the conventional Lucas-Lehmer test for Mersenne numbers.
REFERENCES:
Knuth, D. E. §4.5.4 in The Art of Computer Programming, Vol. 2: Seminumerical Algorithms. Reading, MA: Addison-Wesley, 1969.
Pratt, V. "Every Prime Has a Succinct Certificate." SIAM J. Comput. 4, 214-220, 1975.
Ribenboim, P. "Primality Tests Based on Lucas Sequences." §2.V in The Little Book of Bigger Primes, 2nd ed. New York: Springer-Verlag, p. 63, 2004.
Sloane, N. J. A. Sequences A003010/M3494 and A095847 in "The On-Line Encyclopedia of Integer Sequences."