A pseudoprime is a composite number that passes a test or sequence of tests that fail for most composite numbers. Unfortunately, some authors drop the "composite" requirement, calling any number that passes the specified tests a pseudoprime even if it is prime. Pomerance, Selfridge, and Wagstaff (1980) restrict their use of "pseudoprime" to odd composite numbers.

"Pseudoprime" used without qualification means Fermat pseudoprime, i.e., a composite number that nonetheless satisfies Fermat's little theorem to some base or set of bases.

Carmichael numbers are odd composite numbers that are Fermat pseudoprimes to every base; they are sometimes called absolute pseudoprimes.

The following table gives the number of Poulet numbers psp(2), Euler-Jacobi pseudoprimes ejpsp(2), and strong pseudoprimes spsp(2) to the base 2, and Carmichael numbers CN that are smaller than the first few powers of 10 (Guy 1994). The table below extend Guy's table with the results of Pinch, who calculated the number of pseudoprimes up to .

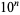	psp(2)	ejpsp(2)	spsp(2)	CN
Sloane	A055550	A055551	A055552	A055553
Sloane counts	A001567	A047713	A001262	A002997
	0	0	0	0
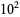	0	0	0	0
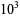	3	1	0	1
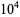	22	12	5	7
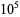	78	36	16	16
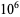	245	114	46	43
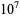	750	375	162	105
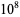	2057	1071	488	255
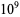	5597	2939	1282	646
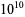	14884	7706	3291	1547
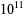	38975	20417	8607	3605
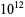	101629	53332	22407	8241
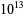	264239	124882	58892	19279

REFERENCES:

Caldwell, C. K. "Prime Links++." http://primes.utm.edu/links/theory/finding_and_proving/probable_primality/.

Grantham, J. "Frobenius Pseudoprimes." http://www.clark.net/pub/grantham/pseudo/pseudo1.ps.

Grantham, J. "Pseudoprimes/Probable Primes." http://www.clark.net/pub/grantham/pseudo/.

Guy, R. K. "Pseudoprimes. Euler Pseudoprimes. Strong Pseudoprimes." §A12 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 27-30, 1994.

Pinch, R. G. E. "The Pseudoprimes Up to 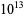." ftp://ftp.dpmms.cam.ac.uk/pub/PSP/.

Pomerance, C.; Selfridge, J. L.; and Wagstaff, S. S. Jr. "The Pseudoprimes to 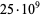." Math. Comput. 35, 1003-1026, 1980. http://mpqs.free.fr/ThePseudoprimesTo25e9.pdf.

Sloane, N. J. A. Sequences A001262, A001567/M5441, A002997/M5462, A047713, A055550, A055551, A055552, and A055553 in "The On-Line Encyclopedia of Integer Sequences."