Intrinsic Particle Properties and Conservation laws

As we have discussed elementary particles so far they have been characterized by essentially three quantities their masses, electric charges and spins. These quantities are important since, as we have seen, they are all quantities which feature in conservation laws, The three relevant laws refer to the conservation of mass energy, the conservation of charge and the conservation of angular momentum. As is to be expected, all elementary particle processes obey these conservation laws as well as the law of conservation of momentum, but it is found in studying the way in which elementary particles interact that other simple conservation laws must be in operation. For example, in any process it is found that the number of baryons less the number of antibaryons at the outset of a process is the same as at the end. This can be

Table 1.1: A few elementary particles.

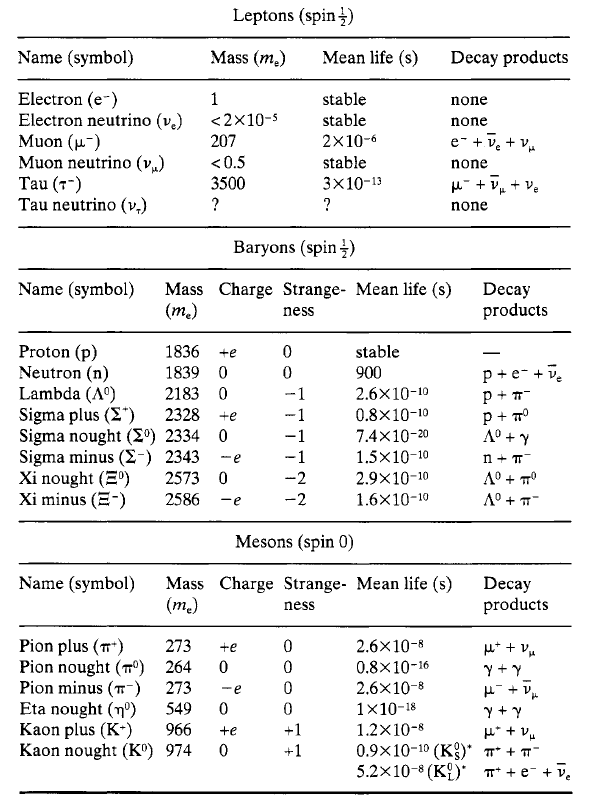
formulated quantitatively by giving every baryon a baryon number (denoted by B) equal to +1, every antibaryon B = -1 and all other particles B = 0 and then requiring that the total baryon number in any process is conserved the law of baryon conservation. This is exemplified in the following processes only one of which can take place. The other, although otherwise allowed, cannot happen because baryon number is not conserved.

(allowed since the total value of B is 2 initially and finally)

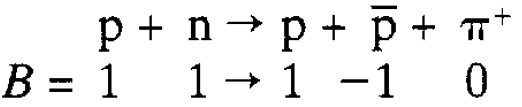
(disallowed since B = 2 initially and B = 0 finally) where the symbol 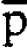 denotes an antiproton with, of course, a negative electric charge. There is a similar conservation law for leptons and their antiparticles. In table 1.1 it will be seen that besides the electron and the neutrino that we have met in nuclear beta decay, there are two further pairs of leptons the muon together with its neutrino and the tau together with its neutrino. It is found that only those processes take place in which the three different brands of lepton are separately conserved. For example, if a muon is emitted or absorbed then its associated neutrino (or antineutrino) will also be involved. Another conservation law of this kind and of great importance is strangeness conservation which only applies, however, to processes resulting from the strong and electromagnetic interactions. The introduction of the concept of ‘strangeness’ arose in the early 1950s to account for the fact that some of the new particles being produced at that time in accelerator experiments could only be created in association with each other. For example the K⁰ could be produced in association with a Λ⁰ and not in association with a neutron. The first of the following processes happens, but the second does not even though none of the conservation laws discussed so far is violated:

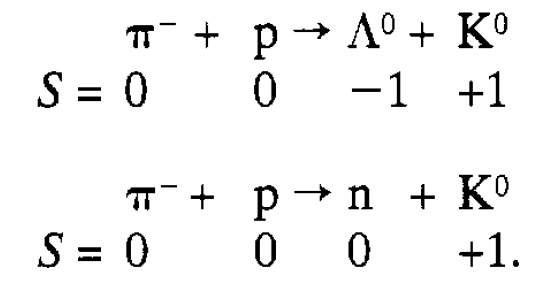
The solution to this strange behaviour was to introduce a conservation law that was violated by the second process. To this end a number called strangeness (denoted by S ) was allocated to all strongly interacting particles (with the opposite sign for the corresponding antiparticle) as in table 1.1. Since these ‘strange’ particles were produced prolifically the production process must involve the strong interaction and it was therefore proposed that it conserved strangeness as in the first of the above processes, but not the second. It will be noted in the table that the Ξ-particle has S = - 2 and, therefore, can only be produced in association with, for example, two K⁰-particles (each having S = +1). However, it will be seen from the table that strangeness is not conserved when strange particles decay it changes by 1. Such decays result from the weak interaction, which therefore does not conserve strangeness. Conservation laws of the type that we have been discussing baryon, lepton and strangeness conservation are, like charge conservation, simple additive laws. The sum of the initial quantities (e.g. B or S) must be equal to the sum of the final quantities. It should be stressed here that, although quantities such as charge, baryon number and strangeness were initially introduced to help codify what can and cannot happen, when detailed theories of these different processes are formulated, the conservation laws arise naturally by requiring the theories to be invariant under certain mathematical transformations. A simple example of invariance in classical physics is that the laws of conservation of momentum and of energy follow from the requirement that the theory of classical mechanics remains the same (i.e. is invariant) whatever position in space and time it is referred to. If it were not then the nature of motion would change from place to place and from time to time. It clearly does not. Invariance under more complicated and less intuitively obvious transformations lead on to the other conservation laws.

مواضيع ذات صلة

انعطاف صغير عند النجوم

ما الحرارة في الاندماج النووي

التسخين بالحقن بذرات متعادلة

الاندماج النووي في المختبر

Broken symmetries

Antimatter

Nuclear forces

د. سميرة موسى علي، الإبداع في الفيزياء النووية

داخل النواة

أجهزة الإنذار الشخصية: عدادات الشارة

أجهزة الإنذار الشخصية: العدادات الجيبية

أجهزة الإنذار الشخصية Personnel Monitoring