
Electric Potential and Electric Field
 المؤلف:
المؤسسة العامة للتعليم الفني والتدريب المهني
المؤلف:
المؤسسة العامة للتعليم الفني والتدريب المهني
 المصدر:
مقدمة عن علم الكهربية الساكنة
المصدر:
مقدمة عن علم الكهربية الساكنة
 الجزء والصفحة:
...
الجزء والصفحة:
...
 16-2-2017
16-2-2017
 2848
2848
Electric Potential and Electric Field
Simple Case (Uniform electric field):
The potential difference between two points A and B in a Uniform electric field E can be found as follow, Assume that a positive test charge qo is moved by an external agent from A to B in uniform electric field as shown in figure 1.1.
The test charge qo is affected by electric force of qoE in the downward direction. To move the charge from A to B an external force F of the same magnitude to the electric force but in the opposite direction. The work W done by the external agent is:

WAB = Fd = qoEd
The potential difference VB-VA is
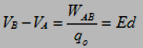
This equation shows the relation between the potential difference and the electric field for a special case (uniform electric field). Note that E has a new unit (V/m). hence,
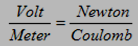
The relation in general case (not uniform electric field):
If the test charge qo is moved along a curved path from A to B as shown in figure 1.2. The electric field exerts a force qoE on the charge. To keep the charge moving without accelerating, an external agent must apply a force F equal to -qoE.
If the test charge moves distance dl along the path from A to B, the work done is F.dl. The total work is given by,
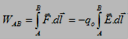
The potential difference VB-VA is,
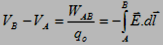
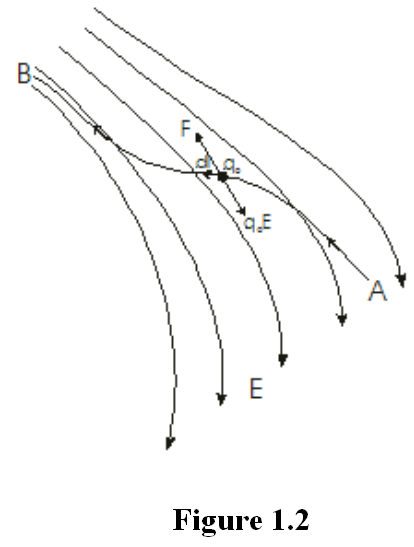
وتكون الزاوية dl هي التي تحدد المسار ومنه اتجاه متجه الإزاحة B الى A لاحظ هنا أن حدود التكامل من ومتجه المجال الكهربي هي الزاوية المحصورة بين منتجه الإزاحة θ.
If the point A is taken to infinity then VA=0 the potential V at point B is,
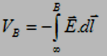
This equation gives the general relation between the potential and the electric field.
Example 1.1
Derive the potential difference between points A and B in uniform electric field using the general case.
Solution
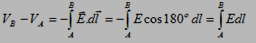
E is uniform (constant) and the integration over the path A to B is d, therefore
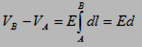
The Electron Volt Unit
A widely used unit of energy in atomic physics is the electron volt (eV).
ELECTRON VOLT, unit of energy, used by physicists to express the energy of ions and subatomic particles that have been accelerated in particle accelerators. One electron volt is equal to the amount of energy gained by an electron traveling through an electrical potential difference of 1 V; this is equivalent to 1.60207 x 10–19J. Electron volts are commonly expressed as million electron volts (MeV) and billion electron volts (BeV or GeV).
Assume two points A and B near to a positive charge q as shown in figure 1.3. To calculate the potential difference VB-VA we assume a test charge qo is moved without acceleration from A to B.
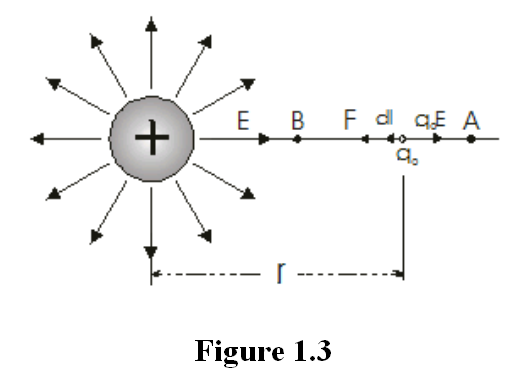
In the figure above the electric field E is directed to the right and dl to the left.

However when we move a distance dl to the left, we are moving in a direction of decreasing r. Thus

Therefore
-Edl = Edr
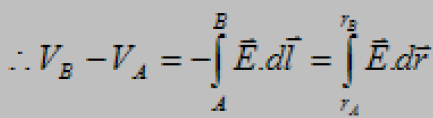
Substitute for E
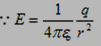
We get
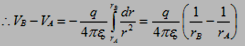
 الاكثر قراءة في الكهربائية
الاكثر قراءة في الكهربائية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


