


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 30-3-2021
Date: 5-4-2021
Date: 12-4-2021
|
The mean deviation (also called the mean absolute deviation) is the mean of the absolute deviations of a set of data about the data's mean. For a sample size 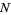 , the mean deviation is defined by
, the mean deviation is defined by
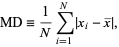 |
(1) |
where  is the mean of the distribution. The mean deviation of a list of numbers is implemented in the Wolfram Language as MeanDeviation[data].
is the mean of the distribution. The mean deviation of a list of numbers is implemented in the Wolfram Language as MeanDeviation[data].
The mean deviation for a discrete distribution 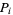 defined for
defined for 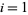 , 2, ...,
, 2, ..., 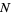 is given by
is given by
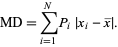 |
(2) |
Mean deviation is an important descriptive statistic that is not frequently encountered in mathematical statistics. This is essentially because while mean deviation has a natural intuitive definition as the "mean deviation from the mean," the introduction of the absolute value makes analytical calculations using this statistic much more complicated than the standard deviation
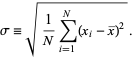 |
(3) |
As a result, least squares fitting and other standard statistical techniques rely on minimizing the sum of square residuals instead of the sum of absolute residuals.
For example, consider the discrete uniform distribution consisting of 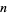 possible outcomes with
possible outcomes with 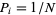 for
for  , 2, ...,
, 2, ..., 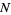 . The mean is given by
. The mean is given by
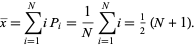 |
(4) |
The variance (and therefore its square root, namely the standard deviation) is also straightforward to obtain as
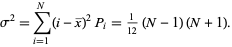 |
(5) |
On the other hand, the mean deviation is given by
 |
(6) |
This can be obtained in closed form, but is much more unwieldy since it requires breaking up the summand into two pieces and treating the cases of  even and odd separately.
even and odd separately.
The following table summarizes the mean absolute deviations for some named continuous distributions, where 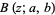 is an incomplete beta function,
is an incomplete beta function, 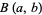 is a beta function,
is a beta function, 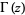 is a gamma function,
is a gamma function, 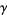 is the Euler-Mascheroni constant,
is the Euler-Mascheroni constant,  is a Meijer G-function,
is a Meijer G-function, 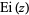 is the exponential integral function,
is the exponential integral function, 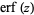 is erf, and
is erf, and 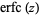 is erfc.
is erfc.
| distribution | M.D. |
| beta distribution | 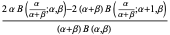 |
| chi-squared distribution | 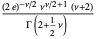 |
| exponential distribution |  |
| gamma distribution | 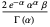 |
| Gumbel distribution | ![beta[G_(2,1)^(2,0)(e,gamma^(-1)|1,1; 0)-Ei(sinhgamma-coshgamma)]](https://mathworld.wolfram.com/images/equations/MeanDeviation/Inline23.gif) |
| half-normal distribution | ![2/theta[e^(-1/pi)+erf(1/(sqrt(pi)))]](https://mathworld.wolfram.com/images/equations/MeanDeviation/Inline24.gif) |
| Laplace distribution |  |
| logistic distribution | 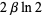 |
| log normal distribution | 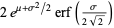 |
| Maxwell distribution | ![4e^(-4/pi)sqrt(2/pi)sigma[1+e^(4/pi)erf(2/(sqrt(pi)))]](https://mathworld.wolfram.com/images/equations/MeanDeviation/Inline28.gif) |
| normal distribution |  |
| Pareto distribution | 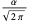 |
| Rayleigh distribution | 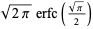 |
| Student's t-distribution | 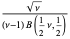 |
| Student's t-distribution | 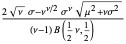 |
| triangular distribution | 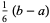 |
| triangular distribution | 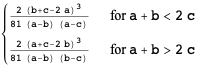 |
| uniform distribution | 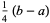 |
The following table summarizes the mean absolute deviations for some named discrete distributions, where 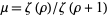 .
.
| distribution | M.D. |
| Bernoulli distribution |  |
| binomial distribution |  |
| discrete uniform distribution | 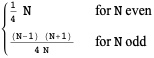 |
| geometric distribution | 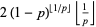 |
| Poisson distribution |  |
| Zipf distribution | ![(2[zeta(rho+1)zeta(rho,|_mu_|+1)-zeta(rho)zeta(rho+1,|_mu_|+1)])/(zeta^2(rho+1))](https://mathworld.wolfram.com/images/equations/MeanDeviation/Inline43.gif) |
REFERENCES:
Havil, J. "Ways of Means." §13.1 in Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 119-121, 2003.
Kenney, J. F. and Keeping, E. S. "Mean Absolute Deviation." §6.4 in Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, pp. 76-77 1962.



|
|
|
|
دراسة تحدد أفضل 4 وجبات صحية.. وأخطرها
|
|
|
|
|
|
|
جامعة الكفيل تحتفي بذكرى ولادة الإمام محمد الجواد (عليه السلام)
|
|
|