


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 2-5-2021
Date: 26-4-2021
Date: 9-3-2021
|
Probability is the branch of mathematics that studies the possible outcomes of given events together with the outcomes' relative likelihoods and distributions. In common usage, the word "probability" is used to mean the chance that a particular event (or set of events) will occur expressed on a linear scale from 0 (impossibility) to 1 (certainty), also expressed as a percentage between 0 and 100%. The analysis of events governed by probability is called statistics.
There are several competing interpretations of the actual "meaning" of probabilities. Frequentists view probability simply as a measure of the frequency of outcomes (the more conventional interpretation), while Bayesians treat probability more subjectively as a statistical procedure that endeavors to estimate parameters of an underlying distribution based on the observed distribution.
A properly normalized function that assigns a probability "density" to each possible outcome within some interval is called a probability density function (or probability distribution function), and its cumulative value (integral for a continuous distribution or sum for a discrete distribution) is called a distribution function (or cumulative distribution function).
A variate is defined as the set of all random variables that obey a given probabilistic law. It is common practice to denote a variate with a capital letter (most commonly  ). The set of all values that
). The set of all values that  can take is then called the range, denoted
can take is then called the range, denoted  (Evans et al. 2000, p. 5). Specific elements in the range of
(Evans et al. 2000, p. 5). Specific elements in the range of  are called quantiles and denoted
are called quantiles and denoted  , and the probability that a variate
, and the probability that a variate 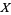 assumes the element
assumes the element  is denoted
is denoted 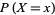 .
.
Probabilities are defined to obey certain assumptions, called the probability axioms. Let a sample space contain the union (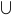 ) of all possible events
) of all possible events 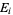 , so
, so
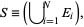 |
(1) |
and let 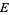 and
and  denote subsets of
denote subsets of 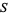 . Further, let
. Further, let 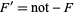 be the complement of
be the complement of  , so that
, so that
 |
(2) |
Then the set 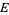 can be written as
can be written as
 |
(3) |
where 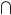 denotes the intersection. Then
denotes the intersection. Then
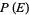 |
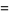 |
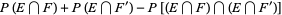 |
(4) |
 |
 |
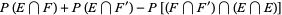 |
(5) |
 |
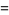 |
 |
(6) |
 |
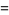 |
 |
(7) |
 |
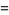 |
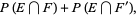 |
(8) |
where 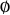 is the empty set.
is the empty set.
Let 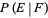 denote the conditional probability of
denote the conditional probability of  given that
given that 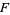 has already occurred, then
has already occurred, then
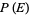 |
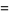 |
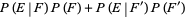 |
(9) |
 |
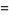 |
 |
(10) |
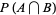 |
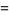 |
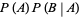 |
(11) |
 |
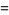 |
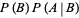 |
(12) |
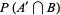 |
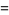 |
 |
(13) |
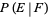 |
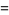 |
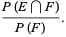 |
(14) |
The relationship
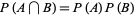 |
(15) |
holds if 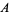 and
and 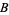 are independent events. A very important result states that
are independent events. A very important result states that
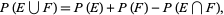 |
(16) |
which can be generalized to
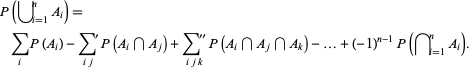 |
(17) |
REFERENCES:
Evans, M.; Hastings, N.; and Peacock, B. Statistical Distributions, 3rd ed. New York: Wiley, 2000.
Everitt, B. Chance Rules: An Informal Guide to Probability, Risk, and Statistics. Copernicus, 1999.
Goldberg, S. Probability: An Introduction. New York: Dover, 1986.
Keynes, J. M. A Treatise on Probability. London: Macmillan, 1921.
Mises, R. von Mathematical Theory of Probability and Statistics. New York: Academic Press, 1964.
Mises, R. von Probability, Statistics, and Truth, 2nd rev. English ed. New York: Dover, 1981.
Mosteller, F. Fifty Challenging Problems in Probability with Solutions. New York: Dover, 1987.
Mosteller, F.; Rourke, R. E. K.; and Thomas, G. B. Probability: A First Course, 2nd ed. Reading, MA: Addison-Wesley, 1970.
Nahin, P. J. Duelling Idiots and Other Probability Puzzlers. Princeton, NJ: Princeton University Press, 2000.
Neyman, J. First Course in Probability and Statistics. New York: Holt, 1950.
Rényi, A. Foundations of Probability. San Francisco, CA: Holden-Day, 1970.
Ross, S. M. A First Course in Probability, 5th ed. Englewood Cliffs, NJ: Prentice-Hall, 1997.
Ross, S. M. Introduction to Probability and Statistics for Engineers and Scientists. New York: Wiley, 1987.
Ross, S. M. Applied Probability Models with Optimization Applications. New York: Dover, 1992.
Ross, S. M. Introduction to Probability Models, 6th ed. New York: Academic Press, 1997.
Székely, G. J. Paradoxes in Probability Theory and Mathematical Statistics, rev. ed. Dordrecht, Netherlands: Reidel, 1986.
Todhunter, I. A History of the Mathematical Theory of Probability from the Time of Pascal to that of Laplace. New York: Chelsea, 1949.
Weaver, W. Lady Luck: The Theory of Probability. New York: Dover, 1963.



|
|
|
|
للعاملين في الليل.. حيلة صحية تجنبكم خطر هذا النوع من العمل
|
|
|
|
|
|
|
"ناسا" تحتفي برائد الفضاء السوفياتي يوري غاغارين
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|