
Probability Models
 المؤلف:
W.D. Wallis
المؤلف:
W.D. Wallis
 المصدر:
Mathematics in the Real World
المصدر:
Mathematics in the Real World
 الجزء والصفحة:
39-40
الجزء والصفحة:
39-40
 14-2-2016
14-2-2016
 2177
2177
A probability model consists of a sample space together with the list of all probabilities of the different outcomes.
One example is a fair die, whose outcomes 1, 2, 3, 4, 5, 6 have probabilities

This is a perfectly good probability model.
Another is a biased die, whose outcomes 1, 2, 3, 4, 5, 6 have probabilities

This is still a good probability model, because the probabilities still add to 1.
In some cases there is no numerical value associated with the outcomes— for example, predicting the weather. However, in many probability models, each outcome is a number, or has a numerical value. For example, this is true of rolling dice.
For example, suppose you are playing a game with three outcomes, call them A,B,C. If the outcome is A, you win $10. If it is B, you win $2. And if it is C, you lose $15. The game is completely random, and observations show that A and C each occur 40% of the time, while B is the result of 20% of plays.
If the game were played 100 times, your best guess would be that A came up 40 times, B 20 and C 40. So someone who played 100 times might expect to win $(10 × 40 + 2 × 20), or $440, and lose $15 × 40 = $600. The net loss is $160, or $1.60 per play. You would say this is the expected cost of a play.
In general, suppose an occurrence has a numerical value associated with outcome, and a probability also. The expected value of the occurrence is found by multiplying each value by the associated probability, and adding. This is also called the mean value, or mean of the occurrence. The mean is most commonly denoted m.
Sample Problem 1.1 You are playing a game where you draw cards from a standard pack. If you draw a 2, 3, . . . , or 10, you score 1 point; a Jack, Queen or King is worth 3; and an Ace is 5 points. How many points would you get for a typical draw?
Solution. The probability of a draw worth 1 point is 36/52, or 9/13, since 36 of the 52 cards are worth 1 point, Similarly, the probabilities of 3 and 5 are 3/13 and 1/13 respectively. So the expected value is
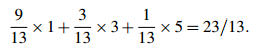
Similar calculations can be applied to any probability model where the outcomes have values associated with them.
 الاكثر قراءة في الاحتمالات و الاحصاء
الاكثر قراءة في الاحتمالات و الاحصاء
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


