
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
ANGULAR MOMENTUM IN QUANTUM STATES
المؤلف:
Mark Csele
المصدر:
FUNDAMENTALS OF LIGHT SOURCES AND LASERS
الجزء والصفحة:
p56
9-3-2016
3373
ANGULAR MOMENTUM IN QUANTUM STATES
Number n, which in the Bohr model represented an orbit. If we are to continue using the analogy of an electron as an orbiting satellite around a planet, we must also account for the angular momentum of the satellite. By knowing the total kinetic energy and the total angular momentum of a planet, we may predict its orbit and energy. Refining the analogy even further, planets have two types of angular momentum: orbital and spin. The spin is an intrinsic angular momentum; in the case of the Earth, it is the spin of the planet that brings about day and night. Furthermore, the orbiting satellite in our case, a charged electron generates a magnetic field by virtue of the fact that we have a moving charge. Both effects, magnetic and spin, contribute to the complete description of an energy state, but the largest influence is that of the orbital angular momentum of the electron, so we shall deal with it first. One major shortcoming of the Bohr model was the failure to account for the hyperfine structure of hydrogen lines. Each line emitted from hydrogen is, in fact, a series of very closely spaced lines. In 1916, the physicist Arnold Sommerfeld suggested that orbiting electrons could follow not only a circular orbit (as in the Bohr model) but also elliptical orbits, and each orbit would have a slightly different energy than that of the circular orbit. His theory was that only certain elliptical orbits would be allowed in the atom fitting the requirements for quantization in the atom. A set of allowed orbits, then, would exist for each principal quantum number n. In the case of the hydrogen emission spectrum, transitions between these closely spaced energy levels give rise to the many closely spaced lines seen. Every particle in orbit has a constant angular momentum in that orbit equal to its mass times its velocity (where velocity is a vector quantity having the property of direction). The same is true whether an orbit is circular (in which case l = mv) or noncircular (in which case L = mvr sin θ). The later case is depicted in Figure 1.1, where angular momentum is the same at every point in the orbit of the particle. When the particle approaches the center (at radius r2 in the figure), speed increases; when
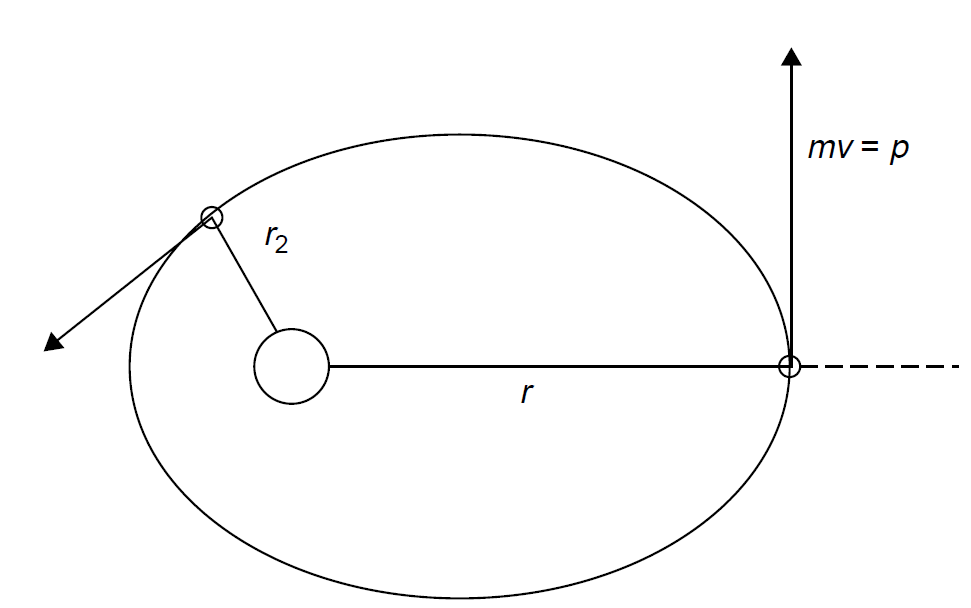
Figure 1.1. Angular momentum of a particle.
the radius is larger, the speed is lower. This is simply conservation of momentum, a concept from basic kinematics, which holds true here as well. By analogy, electrons orbiting around a nucleus will also exhibit angular momentum. Having angular momentum implies that the electron has kinetic energy whose amount is limited by the total electron energy (in the case of an atom, the total energy is described by the principal quantum number n). Like other quantities in an atom, angular momentum is quantized into allowed values; this is one result of the solution of wave equations (Schrodinger wave equations), which describe the behavior of the electron. Angular momentum consists of two key components: orbital angular momentum and spin angular momentum. Spin is an intrinsic property of electrons and is manifested by the magnetic moment created by spinning electrons. Orbital angular momentum is somewhat more straightforward from a classical perspective and is examined first. We now introduce a quantum number, l, to describe orbital angular momentum. This number, the orbital quantum number, describes the magnitude of the orbital angular momentum. Values for l are constrained by the principal quantum number n and can have integer values of zero to n -1. For a principal quantum number of n = 1, l always has a value of zero. This indeed states that the lowest orbit has an angular momentum of zero—at odds with Bohr’s original model! For a principal quantum number of n = 2, l can be 0 or 1, meaning that an electron in the n = 2 state can have zero angular momentum, corresponding to a circular orbit, or some discrete value of angular momentum, corresponding to an elliptical orbit. In either case (=0 or 1), the electrons have almost the same energy (i.e., they are still both in the n =2 principal state). Both quantum numbers together, n and l, are required to fully describe the energy of a given state.
 الاكثر قراءة في ميكانيكا الكم
الاكثر قراءة في ميكانيكا الكم
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















