
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
Helmholtzs theorem: A mathematical digression
المؤلف:
Richard Fitzpatrick
المصدر:
Classical Electromagnetism
الجزء والصفحة:
p 83
2-1-2017
1993
Helmholtz's theorem: A mathematical digression
Let us now embark on a slight mathematical digression. Up to now we have only studied the electric and magnetic fields generated by stationary charges and steady currents. We have found that these fields are describable in terms of four field equations:
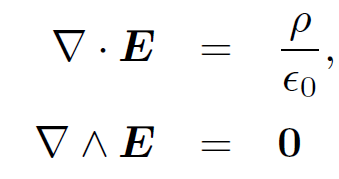 (1.1)
(1.1)
for electric fields, and
 (1.2)
(1.2)
for magnetic fields. There are no other field equations. This strongly suggests that if we know the divergence and the curl of a vector field then we know everything there is to know about the field. In fact, this is the case. There is a mathematical theorem which sums this up. It is called Helmholtz's theorem after the German polymath Hermann Ludwig Ferdinand von Helmholtz. Let us start with scalar fields. Field equations are a type of differential equation; i.e., they deal with the infinitesimal differences in quantities between neigh bouring points. The question is, what differential equation completely specifies a scalar field? This is easy. Suppose that we have a scalar field ϕ and a field equation which tells us the gradient of this field at all points: something like
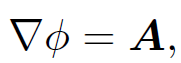 (1.3)
(1.3)
where A(r) is a vector field. Note that we need ∇ ˄ A = 0 for self consistency, since the curl of a gradient is automatically zero. The above equation completely specifies ϕ once we are given the value of the field at a single point, P say. Thus,
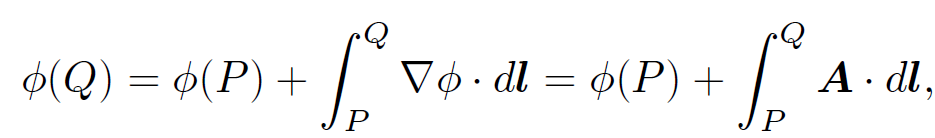 (1.4)
(1.4)
where Q is a general point. The fact that ∇ ˄ A = 0 means that A is a conservative field which guarantees that the above equation gives a unique value for ϕ at a general point in space. Suppose that we have a vector field F. How many differential equations do we need to completely specify this field? Hopefully, we only need two: one giving the divergence of the field and one giving its curl. Let us test this hypothesis. Suppose that we have two field equations:
 (1.5a)
(1.5a)
 (1.5b)
(1.5b)
where D is a scalar field and C is a vector field. For self-consistency we need
 (1.6)
(1.6)
since the divergence of a curl is automatically zero. The question is, do these two field equations plus some suitable boundary conditions completely specify F? Suppose that we write
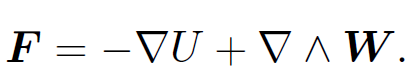 (1.7)
(1.7)
In other words, we are saying that a general field F is the sum of a conservative field, ∇U, and a solenoidal field, ∇˄W. This sounds plausible, but it remains to be proved. Let us start by taking the divergence of the above equation and making use of Eq. (1.5a). We get
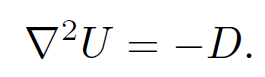 (1.8)
(1.8)
Note that the vector field W does not figure in this equation because the divergence of a curl is automatically zero. Let us now take the curl of Eq. (1.7):
 (1.9)
(1.9)
Here, we assume that the divergence of W is zero. This is another thing which remains to be proved. Note that the scalar field U does not figure in this equation because the curl of a divergence is automatically zero. Using Eq. (1.5b) we get
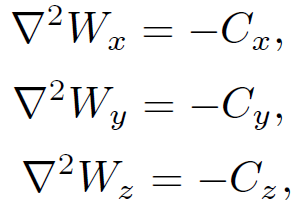 (1.10)
(1.10)
So, we have transformed our problem into four differential equations, Eq. (1.8) and Eqs. (1.10), which we need to solve. Let us look at these equations. We immediately notice that they all have exactly the same form. In fact, they are all versions of Poisson's equation. We can now make use of a principle made famous by Richard P. Feynman: ''the same equations have the same solutions." Recall that earlier on we came across the following equation:
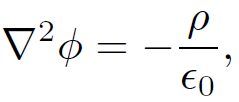 (1.11)
(1.11)
where ϕ is the electrostatic potential and ρ is the charge density. We proved that the solution to this equation, with the boundary condition that ϕ goes to zero at infinity, is
 (1.12)
(1.12)
Well, if the same equations have the same solutions, and Eq. (1.12) is the solution to Eq. (1.11), then we can immediately write down the solutions to Eq. (1.8) and Eqs. (1.10). We get
 (1.13)
(1.13)
and
 (1.14)
(1.14)

The last three equations can be combined to form a single vector equation:
 (1.15)
(1.15)
We assumed earlier that ∇ .W = 0. Let us check to see if this is true. Note that
 (1.16)
(1.16)
which implies that
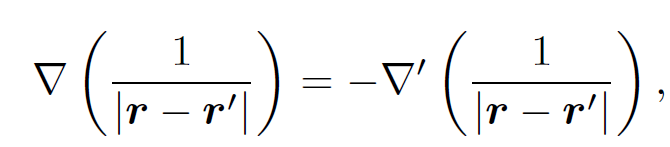 (1.17)
(1.17)
where ∇ʹ is the operator (∂/∂xʹ, ∂/∂yʹ, ∂/∂zʹ). Taking the divergence of Eq. (1.15) and making use of the above relation, we obtain
 (1.18)
(1.18)
Now
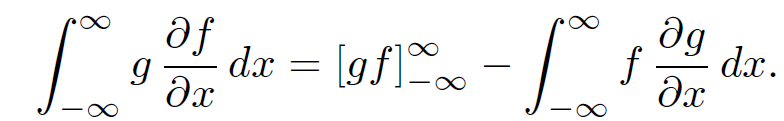 (1.19)
(1.19)
However, if gf → 0 as x → ±∞ then we can neglect the first term on the right-hand side of the above equation and write
 (1.20)
(1.20)
A simple generalization of this result yields
 (1.21)
(1.21)
provided that gx f → 0 as |r| → ∞, etc. Thus, we can deduce that
 (1.22)
(1.22)
from Eq. (1.18), provided |C(r)| is bounded as |r| → ∞. However, we have already shown that ∇ . C = 0 from self-consistency arguments, so the above equation implies that ∇ .W = 0, which is the desired result. We have constructed a vector field F which satisfies Eqs. (1.5) and behaves sensibly at infinity; i.e., |F| → 0 as |r| → ∞. But, is our solution the only possible solution of Eqs. (1.5) with sensible boundary conditions at infinity? Another way of posing this question is to ask whether there are any solutions of
 (1.23)
(1.23)
where i denotes x, y, or z, which are bounded at infinity. If there are then we are in trouble, because we can take our solution and add to it an arbitrary amount of a vector field with zero divergence and zero curl and thereby obtain another solution which also satisfies physical boundary conditions. This would imply that our solution is not unique. In other words, it is not possible to unambiguously reconstruct a vector field given its divergence, its curl, and physical boundary conditions. Fortunately, the equation
 (1.24)
(1.24)
which is called Laplace's equation, has a very nice property: its solutions are unique. That is, if we can find a solution to Laplace's equation which satisfies the boundary conditions then we are guaranteed that this is the only solution. We shall prove this later on in the course. Well, let us invent some solutions to Eqs. (1.23) which are bounded at infinity. How about
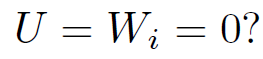 (1.25)
(1.25)
These solutions certainly satisfy Laplace's equation and are well-behaved at infinity. Because the solutions to Laplace's equations are unique, we know that Eqs. (1.25) are the only solutions to Eqs. (1.23). This means that there is no vector field which satisfies physical boundary equations at infinity and has zero divergence and zero curl. In other words, our solution to Eqs. (1.5) is the only solution. Thus, we have unambiguously reconstructed the vector field F given its divergence, its curl, and sensible boundary conditions at infinity. This is Helmholtz's theorem. We have just proved a number of very useful, and also very important, points. First, according to Eq. (1.7), a general vector field can be written as the sum of a conservative field and a solenoidal field. Thus, we ought to be able to write electric and magnetic fields in this form. Second, a general vector field which is zero at infinity is completely specified once its divergence and its curl are given. Thus, we can guess that the laws of electromagnetism can be written as four field equations,
 (1.26)
(1.26)
without knowing the first thing about electromagnetism (other than the fact that it deals with two vector fields). Of course, Eq. (1.1) and (1.2) are of exactly this form. We also know that there are only four field equations, since the above equations are sufficient to completely reconstruct both E and B. Furthermore, we know that we can solve the field equations without even knowing what the right-hand sides look like. After all, we solved Eqs. (1.5) for completely general right-hand sides. (Actually, the right-hand sides have to go to zero at infinity otherwise integrals like Eq. (1.13) blow up.) We also know that any solutions we find are unique. In other words, there is only one possible steady electric and magnetic field which can be generated by a given set of stationary charges and steady currents. The third thing which we proved was that if the right-hand sides of the above field equations are all zero then the only physical solution is E = B = 0. This implies that steady electric and magnetic fields cannot generate themselves, instead they have to be generated by stationary charges and steady currents. So, if we come across a steady electric field we know that if we trace the field lines back we shall eventually find a charge. Likewise, a steady magnetic field implies that there is a steady current flowing somewhere. All of these results follow from vector field theory, i.e., from the general properties of fields in three dimensional space, prior to any investigation of electromagnetism.
 الاكثر قراءة في الكهرومغناطيسية
الاكثر قراءة في الكهرومغناطيسية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















