


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 13-2-2019
Date: 12-3-2017
Date: 23-2-2019
|
What is the quadratic formula?
The quadratic formula is used to solve a very specific type of equation, called a quadratic equation. These equations are usually written in the following form:
Ax2 + Bx + C = 0
Quadratic Equations
We may use the quadratic formula to solve quadratic equations, which are second order polynomials (the highest exponent is two). In simple terms, that means that there's an x2 term, but no x3 or x4terms etc.
They only have one variable, which is often named x. In the equation shown above, the letters a,b,c represent numbers (constants). For example, this is a typical quadratic equation:
ax2 + bx + c = 0
2x2 - 8x + 7 = 0
Notice that I've replaced "a" with 2, "b" with -8, and "c" with 7. Now, how would you solve that equation for x? There's no way to easily manipulate that equation into an x= form. This is where the quadratic formula comes in handy.
The Quadratic Formula

It looks really complicated, I know. Once you use it a lot you'll learn the formula by heart, but until then keep trying. Actually using it isn't that hard -- just plug in your numbers step-by-step. Just insert the values for a,b,c and you'll be left with two possible answers for x.
Confused? Try to follow this example:
Example:
Solve this equation for x: x2 - 4x + 4 = 0
Solution:
What are the three coefficients (a,b,c)? Remember that "a" is the coefficient in front of the x2 term, b is the coefficient in front of x, and c is that constant at the end.
Therefore, for this equation, a=1, b= -4, and c= 4.
Plug those values into the quadratic formula and solve for x:
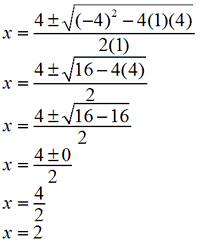
Notice what happened to the +/- sign in that example. Because we were adding or subtracting 0, BOTH answers are the same. There are actually TWO answers for any quadratic formula, and in this case they are both 2.
Check out another example using the quadratic formula where there are two different answers:
Example:
Solve for x: x2 + 2x = 3
Solution:
This equation isn't in the proper form -- we first need to subtract 3 from each side so there's a 0 on the right:
x2 + 2x - 3 = 0
Now we can just use the quadratic formula to get our answers, given that a=1, b=2, c= -3:
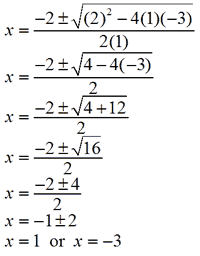
As you can see, we got two answers for this equation. It might seem weird that x could be two different answers, but if you look at the graph for this type of equation, it makes sense. Quadratic equations look like parabolas when graphed, and a parabola can certainly cross the y axis twice (meaning y=0 in two places).
What if you get a negative number inside the square root? That means there are NO possible real answers. This is also possible, and something to look out for. If b2 is not larger than 4ac then the term will be negative and you have no real answers for x.
The quadratic formula is complicated, but if you just follow the steps you'll have no trouble. Memorizing it might take a while, but it's something that will come in very handy.



|
|
|
|
دراسة: حفنة من الجوز يوميا تحميك من سرطان القولون
|
|
|
|
|
|
|
تنشيط أول مفاعل ملح منصهر يستعمل الثوريوم في العالم.. سباق "الأرنب والسلحفاة"
|
|
|
|
|
|
|
الطلبة المشاركون: مسابقة فنِّ الخطابة تمثل فرصة للتنافس الإبداعي وتنمية المهارات
|
|
|