

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Wave Equation-1-Dimensional
المؤلف:
Zwillinger, D.
المصدر:
CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press
الجزء والصفحة:
...
25-7-2018
2268
Wave Equation-1-Dimensional
The one-dimensional wave equation is given by
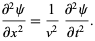 |
(1) |
In order to specify a wave, the equation is subject to boundary conditions
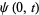 |
 |
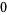 |
(2) |
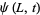 |
 |
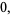 |
(3) |
and initial conditions
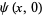 |
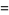 |
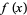 |
(4) |
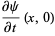 |
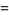 |
 |
(5) |
The one-dimensional wave equation can be solved exactly by d'Alembert's solution, using a Fourier transform method, or via separation of variables.
d'Alembert devised his solution in 1746, and Euler subsequently expanded the method in 1748. Let
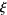 |
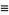 |
 |
(6) |
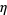 |
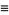 |
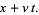 |
(7) |
By the chain rule,
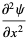 |
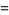 |
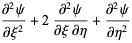 |
(8) |
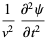 |
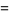 |
 |
(9) |
The wave equation then becomes
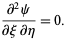 |
(10) |
Any solution of this equation is of the form
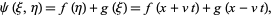 |
(11) |
where 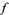 and
and 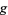 are any functions. They represent two waveforms traveling in opposite directions,
are any functions. They represent two waveforms traveling in opposite directions, 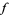 in the negative
in the negative  direction and
direction and 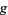 in the positive
in the positive  direction.
direction.
The one-dimensional wave equation can also be solved by applying a Fourier transform to each side,
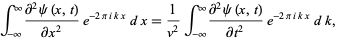 |
(12) |
which is given, with the help of the Fourier transform derivative identity, by
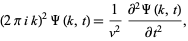 |
(13) |
where
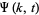 |
 |
](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline33.gif) |
(14) |
 |
 |
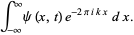 |
(15) |
This has solution
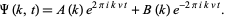 |
(16) |
Taking the inverse Fourier transform gives
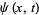 |
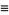 |
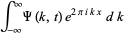 |
(17) |
 |
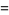 |
![int_(-infty)^infty[A(k)e^(2piikvt)+B(k)e^(-2piikvt)]e^(-2piikx)dk](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline42.gif) |
(18) |
 |
 |
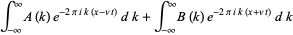 |
(19) |
 |
 |
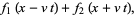 |
(20) |
where
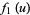 |
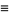 |
=int_(-infty)^inftyA(k)e^(-2piiku)dk](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline51.gif) |
(21) |
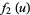 |
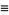 |
=int_(-infty)^inftyB(k)e^(-2piiku)dk.](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline54.gif) |
(22) |
This solution is still subject to all other initial and boundary conditions.
The one-dimensional wave equation can be solved by separation of variables using a trial solution
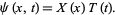 |
(23) |
This gives
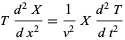 |
(24) |
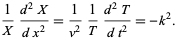 |
(25) |
So the solution for  is
is
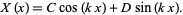 |
(26) |
Rewriting (25) gives
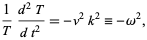 |
(27) |
so the solution for 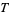 is
is
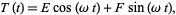 |
(28) |
where 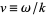 . Applying the boundary conditions
. Applying the boundary conditions 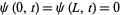 to (◇) gives
to (◇) gives
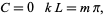 |
(29) |
where 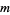 is an integer. Plugging (◇), (◇) and (29) back in for
is an integer. Plugging (◇), (◇) and (29) back in for  in (◇) gives, for a particular value of
in (◇) gives, for a particular value of 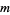 ,
,
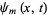 |
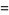 |
![[E_msin(omega_mt)+F_mcos(omega_mt)]D_msin((mpix)/L)](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline64.gif) |
(30) |
 |
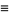 |
![[A_mcos(omega_mt)+B_msin(omega_mt)]sin((mpix)/L).](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/Inline67.gif) |
(31) |
The initial condition 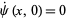 then gives
then gives 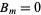 , so (31) becomes
, so (31) becomes
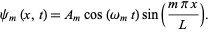 |
(32) |
The general solution is a sum over all possible values of  , so
, so
 |
(33) |
Using orthogonality of sines again,
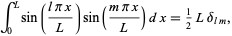 |
(34) |
where 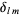 is the Kronecker delta defined by
is the Kronecker delta defined by
|
(35) |
gives
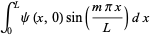 |
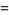 |
 |
(36) |
 |
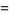 |
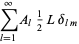 |
(37) |
 |
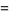 |
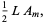 |
(38) |
so we have
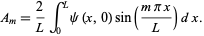 |
(39) |
The computation of 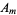 s for specific initial distortions is derived in the Fourier sine series section. We already have found that
s for specific initial distortions is derived in the Fourier sine series section. We already have found that 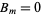 , so the equation of motion for the string (◇), with
, so the equation of motion for the string (◇), with
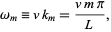 |
(40) |
is
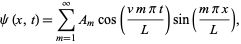 |
(41) |
where the 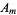 coefficients are given by (◇).
coefficients are given by (◇).
A damped one-dimensional wave
 |
(42) |
given boundary conditions
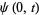 |
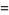 |
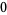 |
(43) |
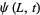 |
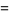 |
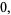 |
(44) |
initial conditions
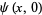 |
 |
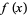 |
(45) |
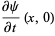 |
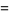 |
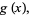 |
(46) |
and the additional constraint
 |
(47) |
can also be solved as a Fourier series.
![psi(x,t)=sum_(n=1)^inftysin((npix)/L)e^(-v^2bt/2)[a_nsin(mu_nt)+b_ncos(mu_nt)],](http://mathworld.wolfram.com/images/equations/WaveEquation1-Dimensional/NumberedEquation23.gif) |
(48) |
where
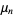 |
 |
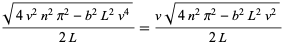 |
(49) |
 |
 |
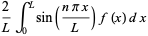 |
(50) |
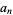 |
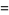 |
(51) |
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Wave Equation in Prolate and Oblate Spheroidal Coordinates." §21.5 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 752-753, 1972.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 124-125 and 271, 1953.
Zwillinger, D. (Ed.). CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, p. 417, 1995.
Zwillinger, D. Handbook of Differential Equations, 3rd ed. Boston, MA: Academic Press, p. 130, 1997.
 الاكثر قراءة في المعادلات التفاضلية الجزئية
الاكثر قراءة في المعادلات التفاضلية الجزئية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















