
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
KEPLERS LAWS
المؤلف:
E. R. Huggins
المصدر:
Physics 2000
الجزء والصفحة:
248
23-11-2020
1754
KEPLER'S LAWS
A more detailed test of Newton's laws and the Orbit 1 program is provided by Kepler's laws of planetary motion.
To get a feeling for the problems involved in studying planetary motion, imagine that you were given the job of going outside, looking at the sky, and figuring out how celestial objects moved. The easiest to start with is the moon, which becomes full again every four weeks. On closer observation you would notice that the moon moved past the background of the apparently fixed stars, returning to its original position in the sky every 27.3 days. Since, as we mentioned, the diameter of the moon does not change much, you might then conclude that the moon is in a circular orbit about the earth, with a period of 27.3 days.
The time it takes the moon to return to the same point in the sky is not precisely equal to the time between full moons. A full moon occurs when the sun, earth, and moon are in alignment. If the sun itself appears to move relative to the fixed stars, the full moons will not occur at precisely the same point, and the time between full moons will not be exactly the time it takes the moon to go around once.
To study the motion of the sun past the background of the fixed stars is more difficult because the stars are not visible when the sun is up. One way to locate the position of the sun is to observe what stars are overhead at "true" midnight, half way between dusk and dawn. The sun should then be located on the opposite side of the sky. (You also have to correct for the north/south position of the sun.) After a fair amount of observation and calculations, you would find that the sun itself moves past the background of the fixed stars, returning to its starting point once a year.
From the fact that the sun takes one year to go around the sky, and the fact that its apparent diameter remains essentially constant, you might well conclude that the sun, like the moon, is traveling in a circular orbit about the earth. This was the accepted conclusion by most astronomers up to the time of Nicolaus Copernicus in the early 1500s AD.
If you start looking at the motion of the planets like Mercury, Venus, Mars, Jupiter, and Saturn, all easily visible without a telescope, the situation is more complicated. Mars, for example, moves in one direction against the background of the fixed stars, then reverses and goes backward for a while, then forward again as shown in Figure (1). None of the planets has the simple uniform motion seen in the case of the moon and the sun.
After a lot of observation and the construction of many plots, you might make a rather significant discovery. You might find what the early Greek astronomers learned, namely that if you assume that the planets Mercury, Venus, Mars, Jupiter, and Saturn travel in circular orbits about the sun, while the sun is traveling in a circular orbit about the earth, then you can explain all the peculiar motion of the planets. This is a remarkable simplification and compelling evidence that there is a simple order underlying the motion of celestial objects.
One of the features of astronomical observations is that they become more accurate as time passes. If you observe the moon for 100 orbits, you can determine the average period of the moon nearly 100 times more accurately than from the observation of a single period. You can also detect any gradual shift of the orbit 100 times more accurately.

Figure 1: Retrograde motion of the planet Mars. Modern view of why Mars appears to reverse its direction of motion for a while.
Even by the time of the famous Greek astronomer Ptolemy in the second century AD, observations of the positions of the planets had been made for a sufficiently long time that it had become clear that the planets did not travel in precisely circular orbits about the sun. Some way was needed to explain the non circularity of the orbits.
The simplicity of a circular orbit was such a compelling idea that it was not abandoned. Recall that the apparently peculiar motion of Mars could be explained by assuming that Mars traveled in a circular orbit about the sun which in turn traveled in a circular orbit about the earth. By having circular orbits centered on points that are themselves in circular orbits, you can construct complex orbits. By choosing enough circles with the correct radii and periods, you can construct any kind of orbit you wish. Ptolemy explained the slight variations in the planetary orbits by assuming that the planets traveled in circles around points which traveled in circles about the sun, which in turn traveled in a circle about the earth. The extra cycle in this scheme was called an epicycle. With just a few epicycles, Ptolemy was able to accurately explain all observations of planetary motion made by the second century AD.
With 1500 more years of planetary observations, Ptolemy's scheme was no longer working well. With far more accurate observations over this long span of time, it was necessary to introduce many more epicycles into Ptolemy's scheme in order to explain the positions of the planets. Even before problems with Ptolemy's scheme became apparent, there were those who argued that the scheme would be simpler if the sun were at the center of the solar system and all the planets, including the earth, moved in circles about the sun. This view was not taken seriously in ancient times, because such a scheme would predict that the earth was moving at a tremendous speed, a motion that surely would be felt. (The principle of relativity was not understood at that time.)
For similar reasons, one did not use the rotation of the earth to explain the daily motion of sun, moon, and stars. That would imply that the surface of the earth at the equator would be moving at a speed of around a thousand miles per hour, an unimaginable speed!
In 1543, Nicolaus Copernicus put forth a detailed plan for the motion of the planets from the point of view that the sun was the center of the solar system and that all the
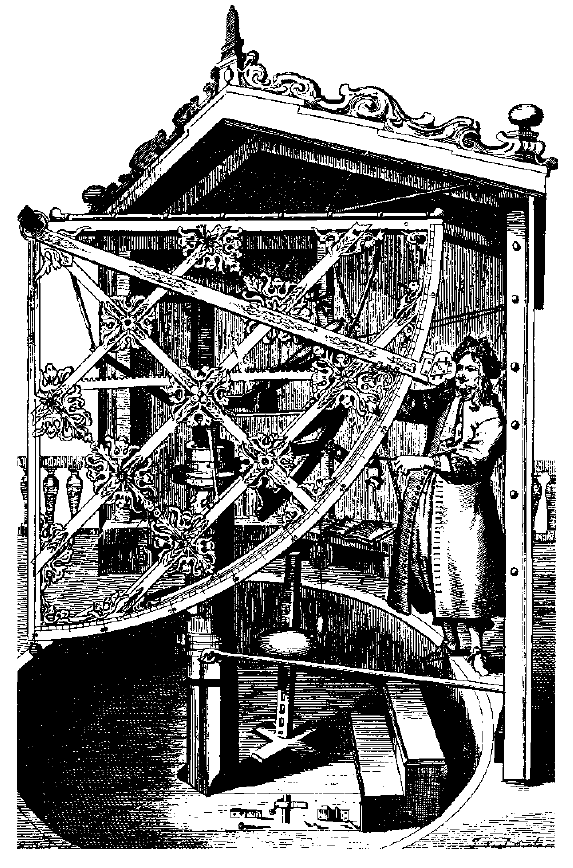
Figure 2:Tycho Brahe’s apparatus.
planets moved in circular orbits about the sun. Such a theory not only conflicted with common sense about feeling the motion of the earth, but also displaced the earth and mankind from the center of the universe, two results quite unacceptable to many scholars and theologians. Copernicus' theory was not quite as simple as it first sounds. Because of the accuracy with which planetary motion was know by 1543, it was necessary to include epicycles in the planetary orbits in Copernicus' model. Starting around 1576, the Dutch astronomer Tycho Brahe made a series of observations of the planetary positions that were a significant improvement over previous measurements.
This work was done before the invention of the telescope, using apparatus like that shown in Figure (2). Tycho Brahe did not happen to believe in the Copernican sun-centered theory, but that had little effect on the reason for making the more accurate observations. Both the Ptolemaic and Copernican systems relied on epicycles, and more accurate data was needed to improve the predictive power of these theories.
Johannes Kepler, a student of Tycho Brahe, started from the simplicity inherent in the Copernican system, but went one step farther than Copernicus. Abandoning the idea that planetary motion had to be described in terms of circular orbits and epicycles, Kepler used Tycho Brahe's accurate data to look for a better way to describe the planet's motion. Kepler found that the planetary orbits were accurately and simply described by ellipses, where the sun was at one of the focuses of the ellipse. (We will soon discuss the properties of ellipses.) Kepler also found a simple rule relating the speed of the planet to the area swept out by a line drawn from the planet to the sun. And thirdly, he discovered that the ratio of the cube of the orbital radius to the square of the period was the same for all planets. These three results are known as Kepler's three laws of planetary motion.
Kepler's three simple rules for planetary motion, which we will discuss in more detail shortly, replaced and improved upon the complex system of epicycles needed by all previous theories. After Kepler's discovery, it was obvious that the sun-centered system and elliptical orbits provided by far the simplest description of the motion of the heavenly objects. For Isaac Newton, half a century later, Kepler's laws served as a fundamental test of his theories of motion and gravitation. We will now use Kepler's laws in a similar way, as a test of the validity of the Orbit 1 program and our techniques for predicting motion.