Band
المؤلف:
Brylinski, J
المصدر:
Loop Spaces, Characteristic Classes, and Geometric Quantization. Boston, MA: Birkhäuser, 1993.
الجزء والصفحة:
...
6-5-2021
1984
Band
A band over a fixed topological space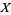 
is represented by a cover 
, 
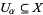, and for each 
, a sheaf of groups 
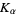on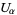 
along with outer automorphisms 
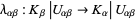satisfying the cocycle conditions 
and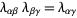 
. Here, restrictions of the cover ![<span style=]()
{U_alpha}" src="https://mathworld.wolfram.com/images/equations/Band/Inline10.gif" style="height:15px; width:27px" /> to a finer cover ![<span style=]()
{V_alpha}" src="https://mathworld.wolfram.com/images/equations/Band/Inline11.gif" style="height:15px; width:25px" /> should be viewed as defining the exact same band.
The collection of all bands over the space 
with respect to a single cover ![<span style=]()
{U_alpha}" src="https://mathworld.wolfram.com/images/equations/Band/Inline13.gif" style="height:15px; width:27px" /> has a natural category structure. Indeed, if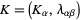 
and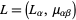 
are two bands over 
with respect to ![<span style=]()
{U_alpha}" src="https://mathworld.wolfram.com/images/equations/Band/Inline17.gif" style="height:15px; width:27px" />, then an isomorphism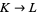 
consists of outer automorphisms 
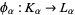compatible on overlaps so that 
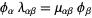. The collection of all such bands and isomorphisms thereof forms a category.
The notion of band is essential to the study of gerbes (Moerdijk). In particular, for a gerbe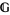 
over a topological space 
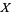, one can choose an open cover 
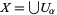of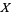 
by open subsets 
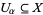, and for each 
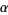, an object 
which together form a sheaf of groups 
on 
. One can then consider a collection of sheaf isomorphisms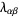 
between any two groups 
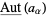and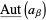 
which forms a collection of well-defined outer automorphisms.
In some literature, an alternative definition of gerbe is used, thereby resulting in an even more specific definition of band. For example, the associated band of some gerbe 
is sometimes assumed to be a sheaf of Lie groups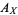 
(Brylinski 1993), though such assumptions appear to be somewhat rare.
REFERENCES:
Brylinski, J. Loop Spaces, Characteristic Classes, and Geometric Quantization. Boston, MA: Birkhäuser, 1993.
Moerdijk, I. "Introduction to the Language of Stacks and Gerbes." 2002. http://arxiv.org/abs/math/0212266.
الاكثر قراءة في التبلوجيا
اخر الاخبار
اخبار العتبة العباسية المقدسة
