

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Jacobi Theta Functions
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
الجزء والصفحة:
...
23-11-2018
3281
Jacobi Theta Functions
The Jacobi theta functions are the elliptic analogs of the exponential function, and may be used to express the Jacobi elliptic functions. The theta functions are quasi-doubly periodic, and are most commonly denoted 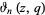 in modern texts, although the notations
in modern texts, although the notations  and
and 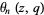 (Borwein and Borwein 1987) are sometimes also used. Whittaker and Watson (1990, p. 487) gives a table summarizing notations used by various earlier writers.
(Borwein and Borwein 1987) are sometimes also used. Whittaker and Watson (1990, p. 487) gives a table summarizing notations used by various earlier writers.
The theta functions are given in the Wolfram Language by EllipticTheta[n, z, q], and their derivatives are given by EllipticThetaPrime[n, z, q].
The translational partition function for an ideal gas can be derived using elliptic theta functions (Golden 1961, pp. 119 and 133; Melzak 1973, p. 122; Levine 2002, p. 838).
The theta functions may be expressed in terms of the nome 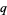 , denoted
, denoted 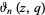 , or the half-period ratio
, or the half-period ratio  , denoted
, denoted 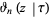 , where
, where 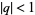 and
and 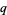 and
and  are related by
are related by
 |
(1) |
Let the multivalued function 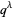 be interpreted to stand for
be interpreted to stand for 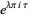 . Then for a complex number
. Then for a complex number  , the Jacobi theta functions are defined as
, the Jacobi theta functions are defined as
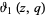 |
 |
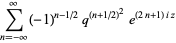 |
(2) |
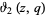 |
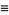 |
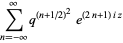 |
(3) |
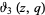 |
 |
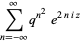 |
(4) |
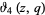 |
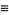 |
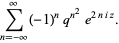 |
(5) |
Writing the doubly infinite sums as singly infinite sums gives the slightly less symmetrical forms
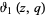 |
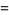 |
![2sum_(n=0)^(infty)(-1)^nq^((n+1/2)^2)sin[(2n+1)z]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline28.gif) |
(6) |
 |
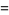 |
![2q^(1/4)sum_(n=0)^(infty)(-1)^nq^(n(n+1))sin[(2n+1)z]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline31.gif) |
(7) |
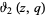 |
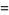 |
![2sum_(n=0)^(infty)q^((n+1/2)^2)cos[(2n+1)z]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline34.gif) |
(8) |
 |
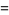 |
![2q^(1/4)sum_(n=0)^(infty)q^(n(n+1))cos[(2n+1)z]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline37.gif) |
(9) |
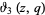 |
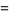 |
 |
(10) |
 |
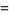 |
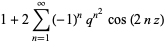 |
(11) |
(Whittaker and Watson 1990, pp. 463-464). Explicitly writing out the series gives
 |
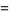 |
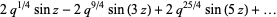 |
(12) |
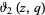 |
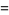 |
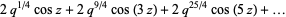 |
(13) |
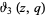 |
 |
 |
(14) |
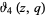 |
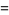 |
 |
(15) |
(Borwein and Borwein 1987, p. 52; Whittaker and Watson 1990, p. 464). 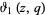 is an odd function of
is an odd function of 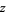 , while the other three are even functions of
, while the other three are even functions of 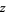 .
.
The following table illustrates the quasi-double periodicity of the Jacobi theta functions.
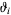 |
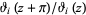 |
 |
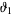 |
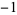 |
 |
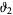 |
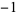 |
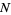 |
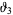 |
1 | 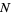 |
 |
1 | 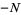 |
Here,
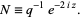 |
(16) |
The quasi-periodicity can be established as follows for the specific case of 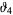 ,
,
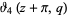 |
 |
 |
(17) |
 |
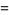 |
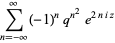 |
(18) |
 |
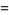 |
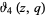 |
(19) |
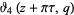 |
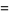 |
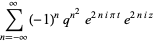 |
(20) |
 |
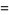 |
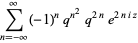 |
(21) |
 |
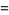 |
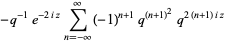 |
(22) |
 |
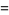 |
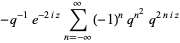 |
(23) |
 |
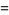 |
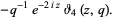 |
(24) |
The Jacobi theta functions can be written in terms of each other:
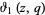 |
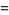 |
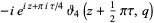 |
(25) |
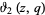 |
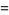 |
 |
(26) |
 |
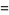 |
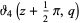 |
(27) |
(Whittaker and Watson 1990, p. 464). Any Jacobi theta function of given arguments can be expressed in terms of any other two Jacobi theta functions with the same arguments.
The functions  and
and 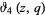 satisfy the identity
satisfy the identity
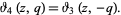 |
(28) |

Define
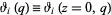 |
(29) |
to be the Jacobi theta functions with argument 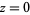 , plotted above. Then the doubly infinite sums (◇) to (◇) take on the particularly simple forms
, plotted above. Then the doubly infinite sums (◇) to (◇) take on the particularly simple forms
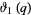 |
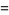 |
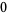 |
(30) |
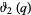 |
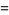 |
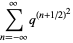 |
(31) |
 |
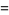 |
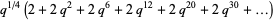 |
(32) |
 |
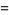 |
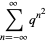 |
(33) |
 |
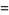 |
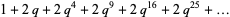 |
(34) |
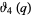 |
 |
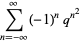 |
(35) |
 |
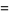 |
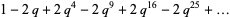 |
(36) |
(OEIS A089800, A000122, and A002448; Borwein and Borwein 1987, p. 33).
The function 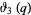 is also given by
is also given by
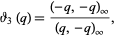 |
(37) |
where 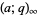 is a q-Pochhammer symbol.
is a q-Pochhammer symbol.
The function
 |
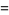 |
 |
(38) |
 |
 |
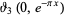 |
(39) |
 |
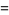 |
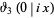 |
(40) |
is sometimes defined in number theoretic contexts (Davenport 1980, p. 62). Similarly, the function
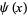 |
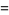 |
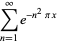 |
(41) |
 |
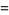 |
![1/2[theta_3(0,e^(-pix))-1]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline146.gif) |
(42) |
is sometimes also defined (Edwards 2001, p. 15). This function satisfies
 |
(43) |
(Jacobi 1828; Riemann 1859; Edwards 2001, p. 15), which Jacobi attributes to Poisson and follows from the Poisson sum formula. Is also satisfies the identity
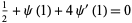 |
(44) |
(Edwards 2001, p. 17).
Special values include
![theta_3(e^(-pi))=(pi^(1/4))/(Gamma(3/4))
theta_3(e^(-pisqrt(2)))=(Gamma(9/8))/(Gamma(5/4))sqrt((Gamma(1/4))/(2^(1/4)pi))
theta_3(e^(-pisqrt(6)))
=[-(Gamma(1/(24))Gamma(5/(24))Gamma(7/(24))Gamma((11)/(24)))/(16sqrt(6)(-18-12sqrt(2)+10sqrt(3)+7sqrt(6))pi^3)]^(1/4)
theta_4(-e^(-pi))=(pi^(1/4))/(Gamma(3/4))
theta_4(e^(-pi))=(pi^(1/4))/(2^(1/4)Gamma(3/4))](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/NumberedEquation8.gif) |
(45) |
and
 |
(46) |
where  is the gamma function, most which are all special cases of the Ramanujan theta functions.
is the gamma function, most which are all special cases of the Ramanujan theta functions.
A special derivative value due to O. Marichev (pers. comm., Jul. 2008) is given by
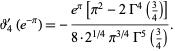 |
(47) |
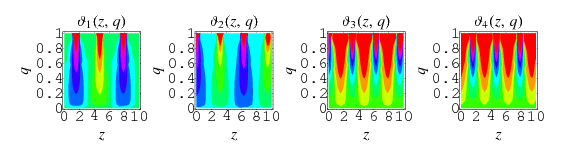
The plots above show the Jacobi theta functions plotted as a function of argument  and nome
and nome  restricted to real values.
restricted to real values.
 |
 |
 |
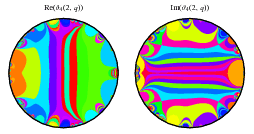 |
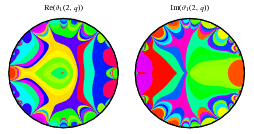
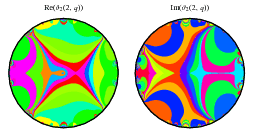
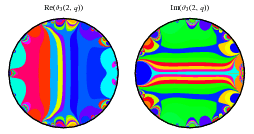
Particularly beautiful plots are obtained by examining the real and imaginary parts of 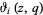 for fixed
for fixed 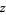 in the complex plane for
in the complex plane for 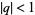 , illustrated above.
, illustrated above.
The Jacobi theta functions satisfy an almost bewilderingly large number of identities involving the four functions, their derivatives, multiples of their arguments, and sums of their arguments. Among the unusual identities given by Whittaker and Watson (1990) are
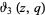 |
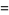 |
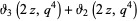 |
(48) |
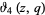 |
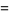 |
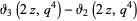 |
(49) |
(Whittaker and Watson 1990, p. 464) and
 |
 |
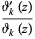 |
(50) |
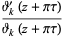 |
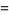 |
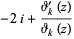 |
(51) |
(Whittaker and Watson 1990, p. 465), for 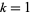 , ..., 4, where
, ..., 4, where 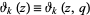 and
and 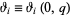 . A class of identities involving the squares of Jacobi theta functions are
. A class of identities involving the squares of Jacobi theta functions are
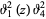 |
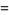 |
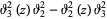 |
(52) |
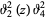 |
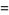 |
 |
(53) |
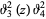 |
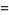 |
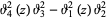 |
(54) |
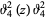 |
 |
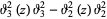 |
(55) |
(Whittaker and Watson 1990, p. 466). Taking  in (55) gives the special case
in (55) gives the special case
 |
(56) |
which is the only identity of this type.
In addition,
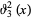 |
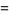 |
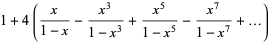 |
(57) |
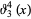 |
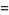 |
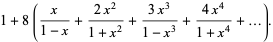 |
(58) |
The Jacobi theta functions obey addition rules such as
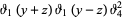 |
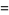 |
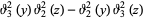 |
(59) |
 |
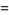 |
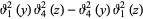 |
(60) |
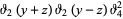 |
 |
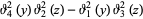 |
(61) |
 |
 |
 |
(62) |
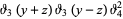 |
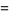 |
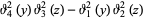 |
(63) |
 |
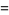 |
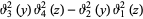 |
(64) |
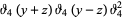 |
 |
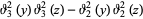 |
(65) |
 |
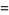 |
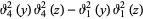 |
(66) |
(Whittaker and Watson 1990, p. 487),
 |
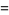 |
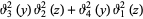 |
(67) |
 |
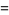 |
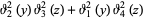 |
(68) |
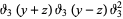 |
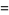 |
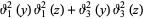 |
(69) |
 |
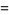 |
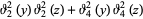 |
(70) |
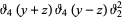 |
 |
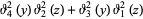 |
(71) |
 |
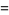 |
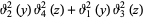 |
(72) |
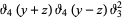 |
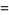 |
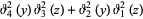 |
(73) |
 |
 |
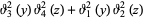 |
(74) |
(Whittaker and Watson 1990, p. 488), and
 |
(75) |
(Whittaker and Watson 1990, p. 488).
There are also a series of duplication formulas:
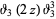 |
 |
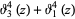 |
(76) |
 |
 |
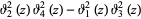 |
(77) |
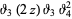 |
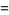 |
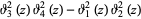 |
(78) |
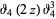 |
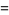 |
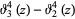 |
(79) |
 |
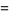 |
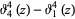 |
(80) |
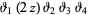 |
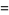 |
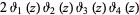 |
(81) |
(Whittaker and Watson 1990, p. 488).
Ratios of Jacobi theta function derivatives to the functions themselves have the simple forms
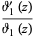 |
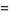 |
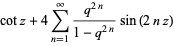 |
(82) |
 |
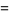 |
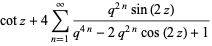 |
(83) |
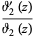 |
 |
 |
(84) |
 |
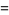 |
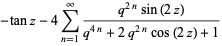 |
(85) |
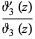 |
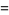 |
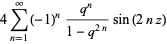 |
(86) |
 |
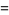 |
 |
(87) |
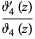 |
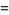 |
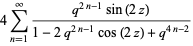 |
(88) |
 |
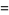 |
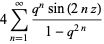 |
(89) |
(Whittaker and Watson 1990, p. 489).
The Jacobi theta functions can be expressed as products instead of sums by
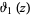 |
 |
![2Gq^(1/4)sinzproduct_(n=1)^(infty)[1-2q^(2n)cos(2z)+q^(4n)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline279.gif) |
(90) |
 |
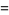 |
![2Gq^(1/4)coszproduct_(n=1)^(infty)[1+2q^(2n)cos(2z)+q^(4n)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline282.gif) |
(91) |
 |
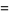 |
![Gproduct_(n=1)^(infty)[1+2q^(2n-1)cos(2z)+q^(4n-2)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline285.gif) |
(92) |
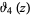 |
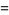 |
![Gproduct_(n=1)^(infty)[1-2q^(2n-1)cos(2z)+q^(4n-2)],](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline288.gif) |
(93) |
where
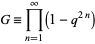 |
(94) |
(Whittaker and Watson 1990, pp. 469-470).
Additional beautiful product ("Eulerian") forms are given by Zucker (1990), partially summarized in the following table, where
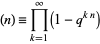 |
(95) |
and the q-products are written 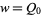 ,
, 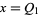 ,
, 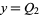 , and
, and 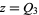 .
.
| theta function | Sloane | Eulerian | Jacobian |
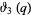 |
A000122 | 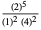 |
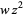 |
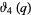 |
A002448 | 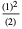 |
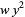 |
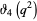 |
A089798 | 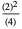 |
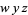 |
 |
A089799 | 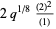 |
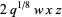 |
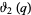 |
A089800 | 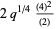 |
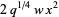 |
![1/2[theta_3(q^(1/3))-theta_3(q^3)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline308.gif) |
A089801 | 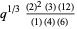 |
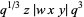 |
![1/2[theta_4(q^3)-theta_4(q^(1/3))]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline311.gif) |
A089802 | 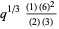 |
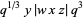 |
![1/2[theta_4(q^6)-theta_4(q^(2/3))]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline314.gif) |
A089805 | 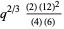 |
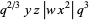 |
![1/2[theta_2(q^(1/6))-theta_2(q^(3/2))]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline317.gif) |
A080995 | 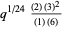 |
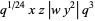 |
![1/2[theta_2(q^(1/3))-theta_2(q^3)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline320.gif) |
A089806 | 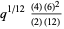 |
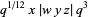 |
![1/2[3theta_3(q^9)-theta_3(q)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline323.gif) |
A089807 | 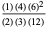 |
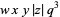 |
![1/2[3theta_4(q^9)-theta_4(q)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline326.gif) |
A089810 | 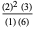 |
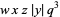 |
![1/2[3theta_4(q^(18))-theta_4(q^2)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline329.gif) |
A089811 | 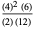 |
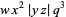 |
![1/2[theta_2(q^(1/2))-3theta_2(q^(9/2))]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline332.gif) |
A089812 |  |
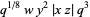 |
![1/2[theta_2(q)-3theta_2(q^9)]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/Inline335.gif) |
A089813 |  |
 |
Additional identities include
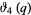 |
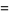 |
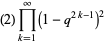 |
(96) |
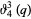 |
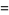 |
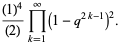 |
(97) |
Here,
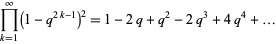 |
(98) |
(OEIS A022597).
The Jacobi theta functions satisfy the partial differential equation
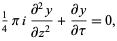 |
(99) |
where 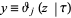 . Ratios of the Jacobi theta functions with
. Ratios of the Jacobi theta functions with 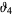 in the denominator also satisfy differential equations
in the denominator also satisfy differential equations
![d/(dz)[(theta_1(z))/(theta_4(z))]=theta_4^2(theta_2(z)theta_3(z))/(theta_4^2(z))](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/NumberedEquation17.gif) |
(100) |
![d/(dz)[(theta_2(z))/(theta_4(z))]=-theta_3^2(theta_1(z)theta_3(z))/(theta_4^2(z))](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/NumberedEquation18.gif) |
(101) |
![d/(dz)[(theta_3(z))/(theta_4(z))]=-theta_2^2(theta_1(z)theta_2(z))/(theta_4^2(z)).](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/NumberedEquation19.gif) |
(102) |
Jacobi's imaginary transformation expresses  in terms of
in terms of 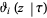 . There are a large number of beautiful identities involving Jacobi theta functions of arguments
. There are a large number of beautiful identities involving Jacobi theta functions of arguments 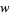 ,
,  ,
,  , and
, and 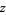 and
and  ,
,  ,
, 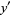 , and
, and 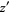 , related by
, related by
 |
 |
 |
(103) |
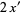 |
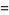 |
 |
(104) |
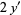 |
 |
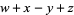 |
(105) |
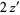 |
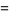 |
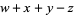 |
(106) |
(Whittaker and Watson 1990, pp. 467-469, 488, and 490). Using the notation
![theta_i(w+pi/2,q)theta_j(x+pi/2,q)theta_k(y,q)theta_l(z,q)=[ijkl]](http://mathworld.wolfram.com/images/equations/JacobiThetaFunctions/NumberedEquation20.gif) |
(107) |
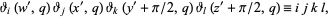 |
(108) |
gives a whopping 288 identities of the form
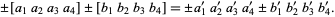 |
(109) |
The complete elliptic integrals of the first and second kinds can be expressed using Jacobi theta functions. Let
 |
(110) |
and plug into (◇)
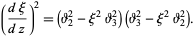 |
(111) |
Now write
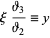 |
(112) |
and
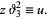 |
(113) |
Then
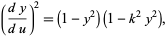 |
(114) |
where the elliptic modulus is defined by
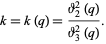 |
(115) |
Define also the complementary elliptic modulus
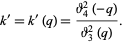 |
(116) |
Now, since
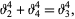 |
(117) |
we have shown
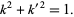 |
(118) |
The solution to the equation is
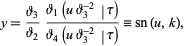 |
(119) |
which is a Jacobi elliptic function with periods
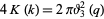 |
(120) |
and
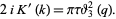 |
(121) |
Letting 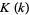 be the complete elliptic integral of the first kind with modulus
be the complete elliptic integral of the first kind with modulus 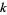 , then
, then
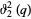 |
 |
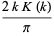 |
(122) |
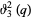 |
 |
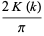 |
(123) |
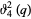 |
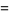 |
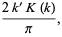 |
(124) |
where 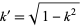 is the complementary modulus.
is the complementary modulus.
The Jacobi theta functions provide analytic solutions to many tricky problems in mathematics and mathematical physics. For example, the Jacobi theta functions are related to the sum of squares function  giving the number of representations of
giving the number of representations of  by two squares via
by two squares via
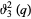 |
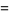 |
 |
(125) |
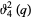 |
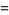 |
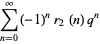 |
(126) |
(Borwein and Borwein 1987, p. 34). The general quintic equation is solvable in terms of Jacobi theta functions, and these functions also provide a uniformly convergent form of the Green's function for a rectangular region (Oberhettinger and Magnus 1949).
Finally, Jacobi theta functions can be used to uniformize all elliptic curves. Jacobi elliptic functions may also be used to uniformize some hyperelliptic curves, although only two such examples are known. The classical example is the Burnside curve, and the second was found by Farkas and Kra around 1995.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 576-579, 1972.
Bellman, R. E. A Brief Introduction to Theta Functions. New York: Holt, Rinehart and Winston, 1961.
Berndt, B. C. "Theta-Functions and Modular Equations." Ch. 25 in Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 138-244, 1994.
Borwein, J. M. and Borwein, P. B. "Theta Functions and the Arithmetic-Geometric Mean Iteration." Ch. 2 in Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, pp. 33-61, 1987.
Davenport, H. Multiplicative Number Theory, 2nd ed. New York: Springer-Verlag, 1980.
Edwards, H. M. Riemann's Zeta Function. New York: Dover, 2001.
Euler, L. Opera Omnia, Series Prima, Vol. 20. Leipzig, Germany, 1912.
Golden, S. Introduction to Theoretical Physical Chemistry. New York: Addison-Wesley, 1961.
Hermite, C. Oeuvres Mathématiques. Paris, 1905-1917.
Jacobi, C. G. J. "Suite des notices sur les fonctions elliptiques." J. reine angew. Math. 3, 303-310, 1828. Reprinted in Gesammelte Mathematische Werke, Vol. 1, pp. 255-263.
Jacobi, C. G. J. Fundamenta Nova Theoriae Functionum Ellipticarum. Königsberg, Germany: Regiomonti, Sumtibus fratrum Borntraeger, 1829. Reprinted in Gesammelte Mathematische Werke, Band. 1. Providence, RI: Amer. Math. Soc., pp. 97-239, 1969.
Klein, F. Vorlesungen über die Theorie der elliptischen Modulfunctionen, 2 vols. Leipzig, Germany: Teubner, 1890-92.
Kronecker, L. "Bemerkungen über die Jacobi'schen Thetaformeln." J. reine angew. Math. 102, 260-272, 1887.
Levine, I. A. Physical Chemistry, 5th ed. New York: McGraw-Hill, 2002.
Melzak, Z. A. Companion to Concrete Mathematics, Vol. 1. New York: Wiley, 1973.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 430-432, 1953.
Oberhettinger, F. and Magnus, W. Anwendung der Elliptischen Funktionen in Physik und Technik. Berlin: Springer-Verlag, 1949.
Riemann, G. F. B. "Über die Anzahl der Primzahlen unter einer gegebenen Grösse." Monatsber. Königl. Preuss. Akad. Wiss. Berlin, 671-680, Nov. 1859.
Reprinted in Das Kontinuum und Andere Monographen (Ed. H. Weyl). New York: Chelsea, 1972. Also reprinted in English translation in Edwards, H. M. Appendix. Riemann's Zeta Function. New York: Dover, pp. 299-305, 2001.
Scheibner, W. "Über die Producte von drei und vier Thetafunctionen." J. reine angew. Math. 102, 255-259, 1887.
Sloane, N. J. A. Sequences A000122, A002448, A022597, A089798, A089799, A089800, A089801, A089802, A089803,A089804, A089805, A080995, A089806, A089807, A089810, A089811, A089812, and A089813 in "The On-Line Encyclopedia of Integer Sequences."
Tannery, J. and Molk, J. Elements de la Theorie des Fonctions Elliptiques, 4 vols. Paris: Gauthier-Villars, 1893-1902.
Tölke, F. "Theta-Funktionen" and "Logarithmen der Theta-Funktionen." Chs. 1-2 in Praktische Funktionenlehre, zweiter Band: Theta-Funktionen und spezielle Weierstraßsche Funktionen. Berlin: Springer-Verlag, pp. 1-83, 1966.
Tölke, F. Praktische Funktionenlehre, fünfter Band: Allgemeine Weierstraßsche Funktionen und Ableitungen nach dem Parameter. Integrale der Theta-Funktionen und Bilinear-Entwicklungen. Berlin: Springer-Verlag, 1968.
Trott, M. "The Mathematica Guidebooks Additional Material: Theta Function Identities." http://www.mathematicaguidebooks.org/additions.shtml#S_3_08.
Weber, H. Elliptische Funktionen und algebraische Zahlen. Brunswick, Germany, 1891.
Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England: Cambridge University Press, 1990.
Zucker, J. "Further Relations Amongst Infinite Series and Products. II. The Evaluation of Three-Dimensional Lattice Sums." J. Phys. A: Math. Gen. 23, 117-132, 1990.
 الاكثر قراءة في التحليل العقدي
الاكثر قراءة في التحليل العقدي
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















