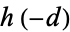 |
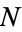 |
Sloane |
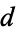 |
| 1 |
9 |
A014602 |
3, 4, 7, 8, 11, 19, 43, 67, 163 |
| 2 |
18 |
A014603 |
15, 20, 24, 35, 40, 51, 52, 88, 91, 115, 123, 148, 187, 232, 235, 267, 403, 427 |
| 3 |
16 |
A006203 |
23, 31, 59, 83, 107, 139, 211, 283, 307, 331, 379, 499, 547, 643, 883, 907 |
| 4 |
54 |
A013658 |
39, 55, 56, 68, 84, 120, 132, 136, 155, 168, 184, 195, 203, 219, 228, 259, 280, 291, 292, 312, 323, 328, 340, 355, 372, 388, 408, 435, 483, 520, 532, 555, 568, 595, 627, 667, 708, 715, 723, 760, 763, 772, 795, 955, 1003, 1012, 1027, 1227, 1243, 1387, 1411, 1435, 1507, 1555 |
| 5 |
25 |
A046002 |
47, 79, 103, 127, 131, 179, 227, 347, 443, 523, 571, 619, 683, 691, 739, 787, 947, 1051, 1123, 1723, 1747, 1867, 2203, 2347, 2683 |
| 6 |
51 |
A046003 |
87, 104, 116, 152, 212, 244, 247, 339, 411, 424, 436, 451, 472, 515, 628, 707, 771, 808, 835, 843, 856, 1048, 1059, 1099, 1108, 1147, 1192, 1203, 1219, 1267, 1315, 1347, 1363, 1432, 1563, 1588, 1603, 1843, 1915, 1963, 2227, 2283, 2443, 2515, 2563, 2787, 2923, 3235, 3427, 3523, 3763 |
| 7 |
31 |
A046004 |
71, 151, 223, 251, 463, 467, 487, 587, 811, 827, 859, 1163, 1171, 1483, 1523, 1627, 1787, 1987, 2011, 2083, 2179, 2251, 2467, 2707, 3019, 3067, 3187, 3907, 4603, 5107, 5923 |
| 8 |
131 |
A046005 |
95, 111, 164, 183, 248, 260, 264, 276, 295, 299, 308, 371, 376, 395, 420, 452, 456, 548, 552, 564, 579, 580, 583, 616, 632, 651, 660, 712, 820, 840, 852, 868, 904, 915, 939, 952, 979, 987, 995, 1032, 1043, 1060, 1092, 1128, 1131, 1155, 1195, 1204, 1240, 1252, 1288, 1299, 1320, 1339, 1348, 1380, 1428, 1443, 1528, 1540, 1635, 1651, 1659, 1672, 1731, 1752, 1768, 1771, 1780, 1795, 1803, 1828, 1848, 1864, 1912, 1939, 1947, 1992, 1995, 2020, 2035, 2059, 2067, 2139, 2163, 2212, 2248, 2307, 2308, 2323, 2392, 2395, 2419, 2451, 2587, 2611, 2632, 2667, 2715, 2755, 2788, 2827, 2947, 2968, 2995, 3003, 3172, 3243, 3315, 3355, 3403, 3448, 3507, 3595, 3787, 3883, 3963, 4123, 4195, 4267, 4323, 4387, 4747, 4843, 4867, 5083, 5467, 5587, 5707, 5947, 6307 |
| 9 |
34 |
A046006 |
199, 367, 419, 491, 563, 823, 1087, 1187, 1291, 1423, 1579, 2003, 2803, 3163, 3259, 3307, 3547, 3643, 4027, 4243, 4363, 4483, 4723, 4987, 5443, 6043, 6427, 6763, 6883, 7723, 8563, 8803, 9067, 10627 |
| 10 |
87 |
A046007 |
119, 143, 159, 296, 303, 319, 344, 415, 488, 611, 635, 664, 699, 724, 779, 788, 803, 851, 872, 916, 923, 1115, 1268, 1384, 1492, 1576, 1643, 1684, 1688, 1707, 1779, 1819, 1835, 1891, 1923, 2152, 2164, 2363, 2452, 2643, 2776, 2836, 2899, 3028, 3091, 3139, 3147, 3291, 3412, 3508, 3635, 3667, 3683, 3811, 3859, 3928, 4083, 4227, 4372, 4435, 4579, 4627, 4852, 4915, 5131, 5163, 5272, 5515, 5611, 5667, 5803, 6115, 6259, 6403, 6667, 7123, 7363, 7387, 7435, 7483, 7627, 8227, 8947, 9307, 10147, 10483, 13843 |
| 11 |
41 |
A046008 |
167, 271, 659, 967, 1283, 1303, 1307, 1459, 1531, 1699, 2027, 2267, 2539, 2731, 2851, 2971, 3203, 3347, 3499, 3739, 3931, 4051, 5179, 5683, 6163, 6547, 7027, 7507, 7603, 7867, 8443, 9283, 9403, 9643, 9787, 10987, 13003, 13267, 14107, 14683, 15667 |
| 12 |
206 |
A046009 |
231, 255, 327, 356, 440, 516, 543, 655, 680, 687, 696, 728, 731, 744, 755, 804, 888, 932, 948, 964, 984, 996, 1011, 1067, 1096, 1144, 1208, 1235, 1236, 1255, 1272, 1336, 1355, 1371, 1419, 1464, 1480, 1491, 1515, 1547, 1572, 1668, 1720, 1732, 1763, 1807, 1812, 1892, 1955, 1972, 2068, 2091, 2104, 2132, 2148, 2155, 2235, 2260, 2355, 2387, 2388, 2424, 2440, 2468, 2472, 2488, 2491, 2555, 2595, 2627, 2635, 2676, 2680, 2692, 2723, 2728, 2740, 2795, 2867, 2872, 2920, 2955, 3012, 3027, 3043, 3048, 3115, 3208, 3252, 3256, 3268, 3304, 3387, 3451, 3459, 3592, 3619, 3652, 3723, 3747, 3768, 3796, 3835, 3880, 3892, 3955, 3972, 4035, 4120, 4132, 4147, 4152, 4155, 4168, 4291, 4360, 4411, 4467, 4531, 4552, 4555, 4587, 4648, 4699, 4708, 4755, 4771, 4792, 4795, 4827, 4888, 4907, 4947, 4963, 5032, 5035, 5128, 5140, 5155, 5188, 5259, 5299, 5307, 5371, 5395, 5523, 5595, 5755, 5763, 5811, 5835, 6187, 6232, 6235, 6267, 6283, 6472, 6483, 6603, 6643, 6715, 6787, 6843, 6931, 6955, 6963, 6987, 7107, 7291, 7492, 7555, 7683, 7891, 7912, 8068, 8131, 8155, 8248, 8323, 8347, 8395, 8787, 8827, 9003, 9139, 9355, 9523, 9667, 9843, 10003, 10603, 10707, 10747, 10795, 10915, 11155, 11347, 11707, 11803, 12307, 12643, 14443, 15163, 15283, 16003, 17803 |
| 13 |
37 |
A046010 |
191, 263, 607, 631, 727, 1019, 1451, 1499, 1667, 1907, 2131, 2143, 2371, 2659, 2963, 3083, 3691, 4003, 4507, 4643, 5347, 5419, 5779, 6619, 7243, 7963, 9547, 9739, 11467, 11587, 11827, 11923, 12043, 14347, 15787, 16963, 20563 |
| 14 |
95 |
A046011 |
215, 287, 391, 404, 447, 511, 535, 536, 596, 692, 703, 807, 899, 1112, 1211, 1396, 1403, 1527, 1816, 1851, 1883, 2008, 2123, 2147, 2171, 2335, 2427, 2507, 2536, 2571, 2612, 2779, 2931, 2932, 3112, 3227, 3352, 3579, 3707, 3715, 3867, 3988, 4187, 4315, 4443, 4468, 4659, 4803, 4948, 5027, 5091, 5251, 5267, 5608, 5723, 5812, 5971, 6388, 6499, 6523, 6568, 6979, 7067, 7099, 7147, 7915, 8035, 8187, 8611, 8899, 9115, 9172, 9235, 9427, 10123, 10315, 10363, 10411, 11227, 12147, 12667, 12787, 13027, 13435, 13483, 13603, 14203, 16867, 18187, 18547, 18643, 20227, 21547, 23083, 30067 |
| 15 |
68 |
A046012 |
239, 439, 751, 971, 1259, 1327, 1427, 1567, 1619, 2243, 2647, 2699, 2843, 3331, 3571, 3803, 4099, 4219, 5003, 5227, 5323, 5563, 5827, 5987, 6067, 6091, 6211, 6571, 7219, 7459, 7547, 8467, 8707, 8779, 9043, 9907, 10243, 10267, 10459, 10651, 10723, 11083, 11971, 12163, 12763, 13147, 13963, 14323, 14827, 14851, 15187, 15643, 15907, 16603, 16843, 17467, 17923, 18043, 18523, 19387, 19867, 20707, 22003, 26203, 27883, 29947, 32323, 34483 |
| 16 |
322 |
A046013 |
399, 407, 471, 559, 584, 644, 663, 740, 799, 884, 895, 903, 943, 1015, 1016, 1023, 1028, 1047, 1139, 1140, 1159, 1220, 1379, 1412, 1416, 1508, 1560, 1595, 1608, 1624, 1636, 1640, 1716, 1860, 1876, 1924, 1983, 2004, 2019, 2040, 2056, 2072, 2095, 2195, 2211, 2244, 2280, 2292, 2296, 2328, 2356, 2379, 2436, 2568, 2580, 2584, 2739, 2760, 2811, 2868, 2884, 2980, 3063, 3108, 3140, 3144, 3160, 3171, 3192, 3220, 3336, 3363, 3379, 3432, 3435, 3443, 3460, 3480, 3531, 3556, 3588, 3603, 3640, 3732, 3752, 3784, 3795, 3819, 3828, 3832, 3939, 3976, 4008, 4020, 4043, 4171, 4179, 4180, 4216, 4228, 4251, 4260, 4324, 4379, 4420, 4427, 4440, 4452, 4488, 4515, 4516, 4596, 4612, 4683, 4687, 4712, 4740, 4804, 4899, 4939, 4971, 4984, 5115, 5160, 5187, 5195, 5208, 5363, 5380, 5403, 5412, 5428, 5460, 5572, 5668, 5752, 5848, 5860, 5883, 5896, 5907, 5908, 5992, 5995, 6040, 6052, 6099, 6123, 6148, 6195, 6312, 6315, 6328, 6355, 6395, 6420, 6532, 6580, 6595, 6612, 6628, 6708, 6747, 6771, 6792, 6820, 6868, 6923, 6952, 7003, 7035, 7051, 7195, 7288, 7315, 7347, 7368, 7395, 7480, 7491, 7540, 7579, 7588, 7672, 7707, 7747, 7755, 7780, 7795, 7819, 7828, 7843, 7923, 7995, 8008, 8043, 8052, 8083, 8283, 8299, 8308, 8452, 8515, 8547, 8548, 8635, 8643, 8680, 8683, 8715, 8835, 8859, 8932, 8968, 9208, 9219, 9412, 9483, 9507, 9508, 9595, 9640, 9763, 9835, 9867, 9955, 10132, 10168, 10195, 10203, 10227, 10312, 10387, 10420, 10563, 10587, 10635, 10803, 10843, 10948, 10963, 11067, 11092, 11107, 11179, 11203, 11512, 11523, 11563, 11572, 11635, 11715, 11848, 11995, 12027, 12259, 12387, 12523, 12595, 12747, 12772, 12835, 12859, 12868, 13123, 13192, 13195, 13288, 13323, 13363, 13507, 13795, 13819, 13827, 14008, 14155, 14371, 14403, 14547, 14707, 14763, 14995, 15067, 15387, 15403, 15547, 15715, 16027, 16195, 16347, 16531, 16555, 16723, 17227, 17323, 17347, 17427, 17515, 18403, 18715, 18883, 18907, 19147, 19195, 19947, 19987, 20155, 20395, 21403, 21715, 21835, 22243, 22843, 23395, 23587, 24403, 25027, 25267, 27307, 27787, 28963, 31243 |
| 17 |
45 |
A046014 |
383, 991, 1091, 1571, 1663, 1783, 2531, 3323, 3947, 4339, 4447, 4547, 4651, 5483, 6203, 6379, 6451, 6827, 6907, 7883, 8539, 8731, 9883, 11251, 11443, 12907, 13627, 14083, 14779, 14947, 16699, 17827, 18307, 19963, 21067, 23563, 24907, 25243, 26083, 26107, 27763, 31627, 33427, 36523, 37123 |
| 18 |
150 |
A046015 |
335, 519, 527, 679, 1135, 1172, 1207, 1383, 1448, 1687, 1691, 1927, 2047, 2051, 2167, 2228, 2291, 2315, 2344, 2644, 2747, 2859, 3035, 3107, 3543, 3544, 3651, 3688, 4072, 4299, 4307, 4568, 4819, 4883, 5224, 5315, 5464, 5492, 5539, 5899, 6196, 6227, 6331, 6387, 6484, 6739, 6835, 7323, 7339, 7528, 7571, 7715, 7732, 7771, 7827, 8152, 8203, 8212, 8331, 8403, 8488, 8507, 8587, 8884, 9123, 9211, 9563, 9627, 9683, 9748, 9832, 10228, 10264, 10347, 10523, 11188, 11419, 11608, 11643, 11683, 11851, 11992, 12067, 12148, 12187, 12235, 12283, 12651, 12723, 12811, 12952, 13227, 13315, 13387, 13747, 13947, 13987, 14163, 14227, 14515, 14667, 14932, 15115, 15243, 16123, 16171, 16387, 16627, 17035, 17131, 17403, 17635, 18283, 18712, 19027, 19123, 19651, 20035, 20827, 21043, 21652, 21667, 21907, 22267, 22443, 22507, 22947, 23347, 23467, 23683, 23923, 24067, 24523, 24667, 24787, 25435, 26587, 26707, 28147, 29467, 32827, 33763, 34027, 34507, 36667, 39307, 40987, 41827, 43387, 48427 |
| 19 |
47 |
A046016 |
311, 359, 919, 1063, 1543, 1831, 2099, 2339, 2459, 3343, 3463, 3467, 3607, 4019, 4139, 4327, 5059, 5147, 5527, 5659, 6803, 8419, 8923, 8971, 9619, 10891, 11299, 15091, 15331, 16363, 16747, 17011, 17299, 17539, 17683, 19507, 21187, 21211, 21283, 23203, 24763, 26227, 27043, 29803, 31123, 37507, 38707 |
| 20 |
350 |
A046017 |
455, 615, 776, 824, 836, 920, 1064, 1124, 1160, 1263, 1284, 1460, 1495, 1524, 1544, 1592, 1604, 1652, 1695, 1739, 1748, 1796, 1880, 1887, 1896, 1928, 1940, 1956, 2136, 2247, 2360, 2404, 2407, 2483, 2487, 2532, 2552, 2596, 2603, 2712, 2724, 2743, 2948, 2983, 2987, 3007, 3016, 3076, 3099, 3103, 3124, 3131, 3155, 3219, 3288, 3320, 3367, 3395, 3496, 3512, 3515, 3567, 3655, 3668, 3684, 3748, 3755, 3908, 3979, 4011, 4015, 4024, 4036, 4148, 4264, 4355, 4371, 4395, 4403, 4408, 4539, 4548, 4660, 4728, 4731, 4756, 4763, 4855, 4891, 5019, 5028, 5044, 5080, 5092, 5268, 5331, 5332, 5352, 5368, 5512, 5560, 5592, 5731, 5944, 5955, 5956, 5988, 6051, 6088, 6136, 6139, 6168, 6280, 6339, 6467, 6504, 6648, 6712, 6755, 6808, 6856, 7012, 7032, 7044, 7060, 7096, 7131, 7144, 7163, 7171, 7192, 7240, 7428, 7432, 7467, 7572, 7611, 7624, 7635, 7651, 7667, 7720, 7851, 7876, 7924, 7939, 8067, 8251, 8292, 8296, 8355, 8404, 8472, 8491, 8632, 8692, 8755, 8808, 8920, 8995, 9051, 9124, 9147, 9160, 9195, 9331, 9339, 9363, 9443, 9571, 9592, 9688, 9691, 9732, 9755, 9795, 9892, 9976, 9979, 10027, 10083, 10155, 10171, 10291, 10299, 10308, 10507, 10515, 10552, 10564, 10819, 10888, 11272, 11320, 11355, 11379, 11395, 11427, 11428, 11539, 11659, 11755, 11860, 11883, 11947, 11955, 12019, 12139, 12280, 12315, 12328, 12331, 12355, 12363, 12467, 12468, 12472, 12499, 12532, 12587, 12603, 12712, 12883, 12931, 12955, 12963, 13155, 13243, 13528, 13555, 13588, 13651, 13803, 13960, 14307, 14331, 14467, 14491, 14659, 14755, 14788, 15235, 15268, 15355, 15603, 15688, 15691, 15763, 15883, 15892, 15955, 16147, 16228, 16395, 16408, 16435, 16483, 16507, 16612, 16648, 16683, 16707, 16915, 16923, 17067, 17187, 17368, 17563, 17643, 17763, 17907, 18067, 18163, 18195, 18232, 18355, 18363, 19083, 19443, 19492, 19555, 19923, 20083, 20203, 20587, 20683, 20755, 20883, 21091, 21235, 21268, 21307, 21387, 21508, 21595, 21723, 21763, 21883, 22387, 22467, 22555, 22603, 22723, 23443, 23947, 24283, 24355, 24747, 24963, 25123, 25363, 26635, 26755, 26827, 26923, 27003, 27955, 27987, 28483, 28555, 29107, 29203, 30283, 30787, 31003, 31483, 31747, 31987, 32923, 33163, 34435, 35683, 35995, 36283, 37627, 37843, 37867, 38347, 39187, 39403, 40243, 40363, 40555, 40723, 43747, 47083, 48283, 51643, 54763, 58507 |
| 21 |
85 |
A046018 |
431, 503, 743, 863, 1931, 2503, 2579, 2767, 2819, 3011, 3371, 4283, 4523, 4691, 5011, 5647, 5851, 5867, 6323, 6691, 7907, 8059, 8123, 8171, 8243, 8387, 8627, 8747, 9091, 9187, 9811, 9859, 10067, 10771, 11731, 12107, 12547, 13171, 13291, 13339, 13723, 14419, 14563, 15427, 16339, 16987, 17107, 17707, 17971, 18427, 18979, 19483, 19531, 19819, 20947, 21379, 22027, 22483, 22963, 23227, 23827, 25603, 26683, 27427, 28387, 28723, 28867, 31963, 32803, 34147, 34963, 35323, 36067, 36187, 39043, 40483, 44683, 46027, 49603, 51283, 52627, 55603, 58963, 59467, 61483 |
| 22 |
139 |
A171724 |
591, 623, 767, 871, 879, 1076, 1111, 1167, 1304, 1556, 1591, 1639, 1903, 2215, 2216, 2263, 2435, 2623, 2648, 2815, 2863, 2935, 3032, 3151, 3316, 3563, 3587, 3827, 4084, 4115, 4163, 4328, 4456, 4504, 4667, 4811, 5383, 5416, 5603, 5716, 5739, 5972, 6019, 6127, 6243, 6616, 6772, 6819, 7179, 7235, 7403, 7763, 7768, 7899, 8023, 8143, 8371, 8659, 8728, 8851, 8907, 8915, 9267, 9304, 9496, 10435, 10579, 10708, 10851, 11035, 11283, 11363, 11668, 12091, 12115, 12403, 12867, 13672, 14019, 14059, 14179, 14548, 14587, 14635, 15208, 15563, 15832, 16243, 16251, 16283, 16291, 16459, 17147, 17587, 17779, 17947, 18115, 18267, 18835, 18987, 19243, 19315, 19672, 20308, 20392, 22579, 22587, 22987, 24243, 24427, 25387, 25507, 25843, 25963, 26323, 26548, 27619, 28267, 29227, 29635, 29827, 30235, 30867, 31315, 33643, 33667, 34003, 34387, 35347, 41083, 43723, 44923, 46363, 47587, 47923, 49723, 53827, 77683, 85507 |
| 23 |
68 |
A046020 |
647, 1039, 1103, 1279, 1447, 1471, 1811, 1979, 2411, 2671, 3491, 3539, 3847, 3923, 4211, 4783, 5387, 5507, 5531, 6563, 6659, 6703, 7043, 9587, 9931, 10867, 10883, 12203, 12739, 13099, 13187, 15307, 15451, 16267, 17203, 17851, 18379, 20323, 20443, 20899, 21019, 21163, 22171, 22531, 24043, 25147, 25579, 25939, 26251, 26947, 27283, 28843, 30187, 31147, 31267, 32467, 34843, 35107, 37003, 40627, 40867, 41203, 42667, 43003, 45427, 45523, 47947, 90787 |
| 24 |
511 |
A048925 |
695, 759, 1191, 1316, 1351, 1407, 1615, 1704, 1736, 1743, 1988, 2168, 2184, 2219, 2372, 2408, 2479, 2660, 2696, 2820, 2824, 2852, 2856, 2915, 2964, 3059, 3064, 3127, 3128, 3444, 3540, 3560, 3604, 3620, 3720, 3864, 3876, 3891, 3899, 3912, 3940, 4063, 4292, 4308, 4503, 4564, 4580, 4595, 4632, 4692, 4715, 4744, 4808, 4872, 4920, 4936, 5016, 5124, 5172, 5219, 5235, 5236, 5252, 5284, 5320, 5348, 5379, 5432, 5448, 5555, 5588, 5620, 5691, 5699, 5747, 5748, 5768, 5828, 5928, 5963, 5979, 6004, 6008, 6024, 6072, 6083, 6132, 6180, 6216, 6251, 6295, 6340, 6411, 6531, 6555, 6699, 6888, 6904, 6916, 7048, 7108, 7188, 7320, 7332, 7348, 7419, 7512, 7531, 7563, 7620, 7764, 7779, 7928, 7960, 7972, 8088, 8115, 8148, 8211, 8260, 8328, 8344, 8392, 8499, 8603, 8628, 8740, 8760, 8763, 8772, 8979, 9028, 9048, 9083, 9112, 9220, 9259, 9268, 9347, 9352, 9379, 9384, 9395, 9451, 9480, 9492, 9652, 9672, 9715, 9723, 9823, 9915, 9928, 9940, 10011, 10059, 10068, 10120, 10180, 10187, 10212, 10248, 10283, 10355, 10360, 10372, 10392, 10452, 10488, 10516, 10612, 10632, 10699, 10740, 10756, 10788, 10792, 10840, 10852, 10923, 11019, 11032, 11139, 11176, 11208, 11211, 11235, 11267, 11307, 11603, 11620, 11627, 11656, 11667, 11748, 11752, 11811, 11812, 11908, 11928, 12072, 12083, 12243, 12292, 12376, 12408, 12435, 12507, 12552, 12628, 12760, 12808, 12820, 12891, 13035, 13060, 13080, 13252, 13348, 13395, 13427, 13444, 13512, 13531, 13539, 13540, 13587, 13611, 13668, 13699, 13732, 13780, 13912, 14035, 14043, 14212, 14235, 14260, 14392, 14523, 14532, 14536, 14539, 14555, 14595, 14611, 14632, 14835, 14907, 14952, 14968, 14980, 15019, 15112, 15267, 15339, 15411, 15460, 15483, 15528, 15555, 15595, 15640, 15652, 15747, 15748, 15828, 15843, 15931, 15940, 15988, 16107, 16132, 16315, 16360, 16468, 16563, 16795, 16827, 16872, 16888, 16907, 16948, 17032, 17043, 17059, 17092, 17283, 17560, 17572, 17620, 17668, 17752, 17812, 17843, 18040, 18052, 18088, 18132, 18148, 18340, 18507, 18568, 18579, 18595, 18627, 18628, 18667, 18763, 18795, 18811, 18867, 18868, 18915, 19203, 19528, 19579, 19587, 19627, 19768, 19803, 19912, 19915, 20260, 20307, 20355, 20427, 20491, 20659, 20692, 20728, 20803, 20932, 20955, 20980, 20995, 21112, 21172, 21352, 21443, 21448, 21603, 21747, 21963, 21988, 22072, 22107, 22180, 22323, 22339, 22803, 22852, 22867, 22939, 23032, 23035, 23107, 23115, 23188, 23235, 23307, 23368, 23752, 23907, 23995, 24115, 24123, 24292, 24315, 24388, 24595, 24627, 24628, 24643, 24915, 24952, 24955, 25048, 25195, 25347, 25467, 25683, 25707, 25732, 25755, 25795, 25915, 25923, 25972, 25987, 26035, 26187, 26395, 26427, 26467, 26643, 26728, 26995, 27115, 27163, 27267, 27435, 27448, 27523, 27643, 27652, 27907, 28243, 28315, 28347, 28372, 28459, 28747, 28891, 29128, 29283, 29323, 29395, 29563, 29659, 29668, 29755, 29923, 30088, 30163, 30363, 30387, 30523, 30667, 30739, 30907, 30955, 30979, 31252, 31348, 31579, 31683, 31795, 31915, 32008, 32043, 32155, 32547, 32635, 32883, 33067, 33187, 33883, 34203, 34363, 34827, 34923, 36003, 36043, 36547, 36723, 36763, 36883, 37227, 37555, 37563, 38227, 38443, 38467, 39603, 39643, 39787, 40147, 40195, 40747, 41035, 41563, 42067, 42163, 42267, 42387, 42427, 42835, 43483, 44947, 45115, 45787, 46195, 46243, 46267, 47203, 47443, 47707, 48547, 49107, 49267, 49387, 49987, 50395, 52123, 52915, 54307, 55867, 56947, 57523, 60523, 60883, 61147, 62155, 62203, 63043, 64267, 79363, 84043, 84547, 111763 |
| 25 |
95 |
A056987 |
479, 599, 1367, 2887, 3851, 4787, 5023, 5503, 5843, 7187, 7283, 7307, 7411, 8011, 8179, 9227, 9923, 10099, 11059, 11131, 11243, 11867, 12211, 12379, 12451, 12979, 14011, 14923, 15619, 17483, 18211, 19267, 19699, 19891, 20347, 21107, 21323, 21499, 21523, 21739, 21787, 21859, 24091, 24571, 25747, 26371, 27067, 27091, 28123, 28603, 28627, 28771, 29443, 30307, 30403, 30427, 30643, 32203, 32443, 32563, 32587, 33091, 34123, 34171, 34651, 34939, 36307, 37363, 37747, 37963, 38803, 39163, 44563, 45763, 48787, 49123, 50227, 51907, 54667, 55147, 57283, 57667, 57787, 59707, 61027, 62563, 63067, 64747, 66763, 68443, 69763, 80347, 85243, 89083, 93307 |


