

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Modulo Multiplication Group
المؤلف:
Eggar, M. H.
المصدر:
"A Curious Property of the Integer 24." Math. Gaz. 84
الجزء والصفحة:
...
12-1-2020
1274
Modulo Multiplication Group
A modulo multiplication group is a finite group 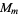 of residue classes prime to
of residue classes prime to  under multiplication mod
under multiplication mod 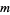 .
. 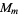 is Abelian of group order
is Abelian of group order 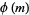 , where
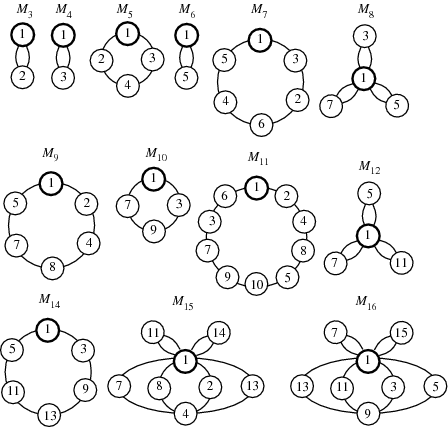
, where 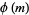 is the totient function.
is the totient function.
A modulo multiplication group can be visualized by constructing its cycle graph. Cycle graphs are illustrated above for some low-order modulo multiplication groups. Such graphs are constructed by drawing labeled nodes, one for each element  of the residue class, and connecting cycles obtained by iterating
of the residue class, and connecting cycles obtained by iterating  . Each edge of such a graph is bidirected, but they are commonly drawn using undirected edges with double edges used to indicate cycles of length two (Shanks 1993, pp. 85 and 87-92).
. Each edge of such a graph is bidirected, but they are commonly drawn using undirected edges with double edges used to indicate cycles of length two (Shanks 1993, pp. 85 and 87-92).
The following table gives the modulo multiplication groups of small orders, together with their isomorphisms with respect to cyclic groups 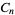 .
.
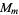 |
group | 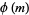 |
elements |
 |
 |
2 | 1 |
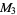 |
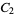 |
2 | 1, 2 |
 |
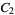 |
2 | 1, 3 |
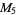 |
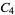 |
4 | 1, 2, 3, 4 |
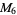 |
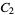 |
2 | 1, 5 |
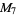 |
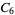 |
6 | 1, 2, 3, 4, 5, 6 |
 |
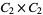 |
4 | 1, 3, 5, 7 |
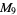 |
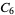 |
6 | 1, 2, 4, 5, 7, 8 |
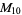 |
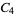 |
4 | 1, 3, 7, 9 |
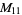 |
 |
10 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 |
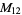 |
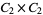 |
4 | 1, 5, 7, 11 |
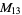 |
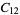 |
12 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12 |
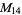 |
 |
6 | 1, 3, 5, 9, 11, 13 |
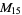 |
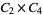 |
8 | 1, 2, 4, 7, 8, 11, 13, 14 |
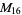 |
 |
8 | 1, 3, 5, 7, 9, 11, 13, 15 |
 |
 |
16 | 1, 2, 3, ..., 16 |
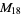 |
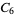 |
6 | 1, 5, 7, 11, 13, 17 |
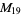 |
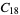 |
18 | 1, 2, 3, ..., 18 |
 |
 |
8 | 1, 3, 7, 9, 11, 13, 17, 19 |
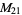 |
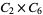 |
12 | 1, 2, 4, 5, 8, 10, 11, 13, 16, 17, 19, 20 |
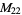 |
 |
10 | 1, 3, 5, 7, 9, 13, 15, 17, 19, 21 |
 |
 |
22 | 1, 2, 3, ..., 22 |
 |
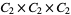 |
8 | 1, 5, 7, 11, 13, 17, 19, 23 |
 is a cyclic group (which occurs exactly when
is a cyclic group (which occurs exactly when  has a primitive root) iff
has a primitive root) iff 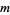 is of one of the forms
is of one of the forms 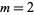 , 4,
, 4, 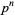 , or
, or 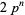 , where
, where  is an odd prime and
is an odd prime and 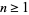 (Shanks 1993, p. 92). The first few of these are
(Shanks 1993, p. 92). The first few of these are 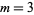 , 4, 5, 6, 7, 9, 10, 11, 13, 14, 17, 18, 19, ... (OEIS A033948; Shanks 1993, p. 84).
, 4, 5, 6, 7, 9, 10, 11, 13, 14, 17, 18, 19, ... (OEIS A033948; Shanks 1993, p. 84).
The only ordered 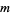 for which the elements of
for which the elements of 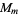 are all self-conjugate are the divisors of 24: 1, 2, 3, 4, 6, 8, 12, 24 (OEIS A018253; Eggar 2000). These correspond to the groups
are all self-conjugate are the divisors of 24: 1, 2, 3, 4, 6, 8, 12, 24 (OEIS A018253; Eggar 2000). These correspond to the groups  ,
,  ,
, 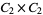 , and
, and 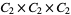 . This also means that no modulo multiplication group is isomorphic to a direct product of more than three copies of
. This also means that no modulo multiplication group is isomorphic to a direct product of more than three copies of 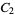 .
.
Isomorphic modulo multiplication groups can be determined using a particular type of factorization of the totient function  using the property that
using the property that
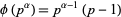 |
(1) |
as described by Shanks (1993, pp. 92-93). To perform this factorization, begin by analogy with computation of the totient function by factoring 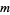 in the standard form
in the standard form
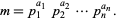 |
(2) |
Now for each power of an odd prime, write
 |
(3) |
and factor the leading term
 |
(4) |
as
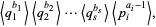 |
(5) |
where 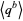 denotes the explicit expansion of
denotes the explicit expansion of 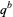 (i.e.,
(i.e.,  ), and the last term is omitted if
), and the last term is omitted if 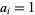 (since in that case,
(since in that case, 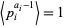 ).
).
If 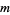 contains a power of 2 so that
contains a power of 2 so that  , then write
, then write
|
(6) |
Now combine terms from the odd and even primes, write them as a product and combine any unambiguous products of terms. The resulting expression is denoted 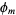 and the group
and the group 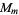 is isomorphic to a direct product of cyclic groups of orders given by
is isomorphic to a direct product of cyclic groups of orders given by 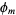 .
.
For example, consider the modulo multiplication group of order 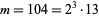 . The only odd prime factor is 13, so factoring gives
. The only odd prime factor is 13, so factoring gives 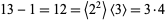 . 104 contains a factor of
. 104 contains a factor of 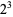 , so the rule for even prime factors gives
, so the rule for even prime factors gives 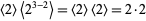 . Combining these two gives
. Combining these two gives 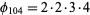 .
.
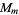 and
and  are isomorphic iff
are isomorphic iff  and
and 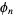 are identical. More specifically, the abstract group corresponding to a given
are identical. More specifically, the abstract group corresponding to a given  can be determined explicitly in terms of a group direct product of cyclic groups of the so-called characteristic factors, whose product is denoted
can be determined explicitly in terms of a group direct product of cyclic groups of the so-called characteristic factors, whose product is denoted 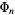 . This representation is obtained from
. This representation is obtained from 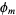 as the set of products of largest powers of each factor of
as the set of products of largest powers of each factor of 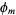 . For example, for
. For example, for 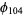 , the largest power of 2 is
, the largest power of 2 is 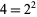 and the largest power of 3 is
and the largest power of 3 is 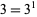 , so the first characteristic factor is
, so the first characteristic factor is 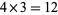 , leaving
, leaving 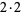 (i.e., only powers of two). The largest power remaining is
(i.e., only powers of two). The largest power remaining is 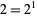 , so the second characteristic factor is 2, leaving 2, which is the third and last characteristic factor. Therefore,
, so the second characteristic factor is 2, leaving 2, which is the third and last characteristic factor. Therefore, 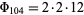 , and the group
, and the group  is isomorphic to
is isomorphic to 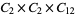 .
.
The following table summarizes the isomorphic modulo multiplication groups 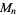 for the first few
for the first few  and identifies the corresponding abstract group. No
and identifies the corresponding abstract group. No 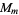 is isomorphic to the cyclic group
is isomorphic to the cyclic group 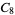 , quaternion group
, quaternion group 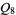 , or the dihedral group
, or the dihedral group 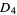 . However, every finite Abelian group is isomorphic to a subgroup of
. However, every finite Abelian group is isomorphic to a subgroup of 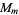 for infinitely many different values of
for infinitely many different values of 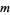 (Shanks 1993, p. 96). Cycle graphs corresponding to
(Shanks 1993, p. 96). Cycle graphs corresponding to 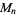 for small
for small  are illustrated above, and more complicated cycle graphs are illustrated by Shanks (1993, pp. 87-92).
are illustrated above, and more complicated cycle graphs are illustrated by Shanks (1993, pp. 87-92).
The following table gives the orders of modulo multiplication groups 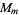 that are isomorphic to direct products of cyclic groups for
that are isomorphic to direct products of cyclic groups for 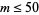 .
.
| group | isomorphic 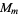 |
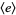 |
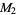 |
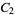 |
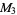 , , 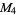 , , 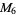 |
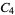 |
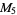 , , 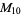 |
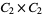 |
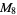 , , 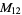 |
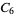 |
 , , 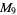 , , 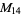 , , 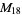 |
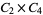 |
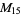 , ,  , , 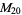 , , 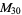 |
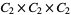 |
 |
 |
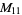 , , 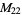 |
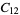 |
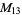 , , 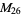 |
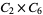 |
 , , 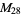 , , 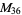 , ,  |
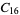 |
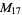 , , 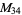 |
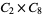 |
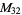 |
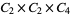 |
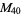 , , 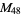 |
 |
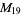 , , 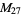 , , 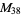 |
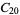 |
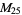 , , 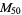 |
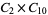 |
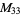 , , 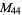 |
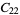 |
 , , 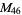 |
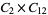 |
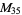 , , 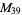 , , 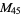 |
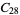 |
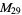 |
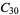 |
 |
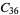 |
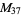 |
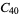 |
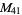 |
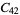 |
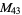 , , 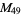 |
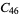 |
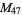 |
The number of characteristic factors 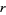 of
of 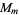 for
for 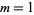 , 2, ... are 1, 1, 1, 1, 1, 1, 1, 2, 1, 1, 1, 2, ... (OEIS A046072).
, 2, ... are 1, 1, 1, 1, 1, 1, 1, 2, 1, 1, 1, 2, ... (OEIS A046072).
The number of quadratic residues in  for
for 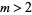 are given by
are given by 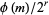 (Shanks 1993, p. 95). The first few for
(Shanks 1993, p. 95). The first few for 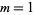 , 2, ... are 0, 1, 1, 1, 2, 1, 3, 1, 3, 2, 5, 1, 6, ... (OEIS A046073).
, 2, ... are 0, 1, 1, 1, 2, 1, 3, 1, 3, 2, 5, 1, 6, ... (OEIS A046073).
In the table below, 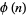 is the totient function (OEIS A000010) factored into characteristic factors,
is the totient function (OEIS A000010) factored into characteristic factors, 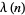 is the Carmichael function (OEIS A011773), and
is the Carmichael function (OEIS A011773), and 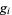 are the smallest generators of the group
are the smallest generators of the group 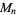 (of which there is a number equal to the number of characteristic factors).
(of which there is a number equal to the number of characteristic factors).
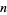 |
 |
 |
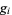 |
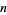 |
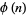 |
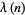 |
 |
| 3 | 2 | 2 | 2 | 27 | 18 | 18 | 2 |
| 4 | 2 | 2 | 3 | 28 | 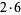 |
6 | 13, 3 |
| 5 | 4 | 4 | 2 | 29 | 28 | 28 | 2 |
| 6 | 2 | 2 | 5 | 30 | 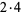 |
4 | 11, 7 |
| 7 | 6 | 6 | 3 | 31 | 30 | 30 | 3 |
| 8 | 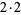 |
2 | 7, 3 | 32 |  |
8 | 31, 3 |
| 9 | 6 | 6 | 2 | 33 | 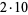 |
10 | 10, 2 |
| 10 | 4 | 4 | 3 | 34 | 16 | 16 | 3 |
| 11 | 10 | 10 | 2 | 35 | 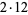 |
12 | 6, 2 |
| 12 | 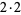 |
2 | 5, 7 | 36 | 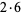 |
6 | 19,5 |
| 13 | 12 | 12 | 2 | 37 | 36 | 36 | 2 |
| 14 | 6 | 6 | 3 | 38 | 18 | 18 | 3 |
| 15 | 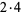 |
4 | 14, 2 | 39 | 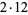 |
12 | 38, 2 |
| 16 |  |
4 | 15, 3 | 40 |  |
4 | 39, 11, 3 |
| 17 | 16 | 16 | 3 | 41 | 40 | 40 | 6 |
| 18 | 6 | 6 | 5 | 42 | 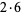 |
6 | 13, 5 |
| 19 | 18 | 18 | 2 | 43 | 42 | 42 | 3 |
| 20 | 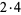 |
4 | 19, 3 | 44 | 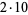 |
10 | 43, 3 |
| 21 | 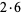 |
6 | 20, 2 | 45 | 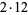 |
12 | 44, 2 |
| 22 | 10 | 10 | 7 | 46 | 22 | 22 | 5 |
| 23 | 22 | 22 | 5 | 47 | 46 | 46 | 5 |
| 24 | 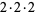 |
2 | 5, 7, 13 | 48 | 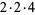 |
4 | 47, 7, 5 |
| 25 | 20 | 20 | 2 | 49 | 42 | 42 | 3 |
| 26 | 12 | 12 | 7 | 50 | 20 | 20 | 3 |
REFERENCES:
Eggar, M. H. "A Curious Property of the Integer 24." Math. Gaz. 84, 96-97, March 2000.
Riesel, H. "The Structure of the Group 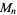 ." Prime Numbers and Computer Methods for Factorization, 2nd ed. Boston, MA: Birkhäuser, pp. 270-272, 1994.
." Prime Numbers and Computer Methods for Factorization, 2nd ed. Boston, MA: Birkhäuser, pp. 270-272, 1994.
Shanks, D. Solved and Unsolved Problems in Number Theory, 4th ed. New York: Chelsea, pp. 61-62 and 92, 1993.
Sloane, N. J. A. Sequences A000010/M0299, A011773, A018253, A033948, A046072, and A046073 in "The On-Line Encyclopedia of Integer Sequences."
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















