Stieltjes Constants
Expanding the Riemann zeta function about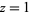 
gives
 |
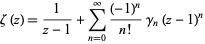(1)
|
(Havil 2003, p. 118), where the constants
![gamma_n=lim_(m->infty)[sum_(k=1)^m((lnk)^n)/k-((lnm)^(n+1))/(n+1)]](http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation2.gif) |
(2)
|
are known as Stieltjes constants.
Another sum that can be used to define the constants is
 |
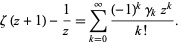(3)
|
These constants are returned by the Wolfram Language function StieltjesGamma[n].
A generalization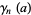 
takes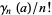 
as the coefficient of 
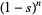is the Laurent series of the Hurwitz zeta function 
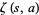about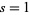 
. These generalized Stieltjes constants are implemented in the Wolfram Language as StieltjesGamma[n, a].
The case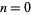 
gives the usual Euler-Mascheroni constant
 |
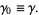(4)
|
A limit formula for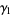 
is given by
![gamma_1=-lim_(y->infty)y<span style=]() {y+I[zeta(1+i/y)]}, " src="http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation5.gif" style="height:37px; width:188px" /> |
(5)
|
where ![I[z]](http://mathworld.wolfram.com/images/equations/StieltjesConstants/Inline9.gif)
is the imaginary part and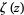 
is the Riemann zeta function.
An alternative definition is given by absorbing the coefficient of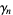 
into the constant,
 |
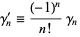(6)
|
(e.g., Hardy 1912, Kluyver 1927).
The Stieltjes constants are also given by
![gamma_n=lim_(z->1)[(-1)^nzeta^((n))(z)-(n!)/((z-1)^(n+1))].](http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation7.gif) |
(7)
|
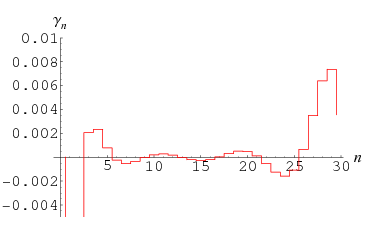
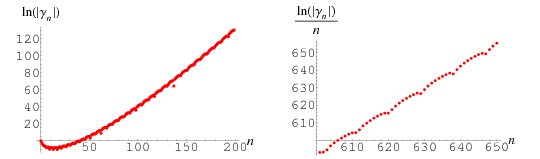
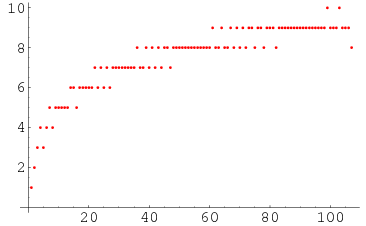
Plots of the values of the Stieltjes constants as a function of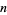 
are illustrated above (Kreminski). The first few numerical values are given in the following table.
 |
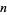OEIS |
 |
| 0 |
A001620 |
0.5772156649 |
| 1 |
A082633 |
 |
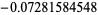| 2 |
A086279 |
 |
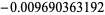| 3 |
A086280 |
0.002053834420 |
| 4 |
A086281 |
0.002325370065 |
| 5 |
A086282 |
0.0007933238173 |
Briggs (1955-1956) proved that there infinitely many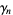 
of each sign. The signs of 
for 
, 1, ... are 1, 
, 
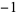, 1, 1, 1, 
, 
, 
, 
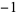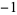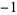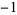, ... (OEIS A114523), and the run lengths of consecutive signs are 1, 2, 3, 4, 3, 4, 5, 4, 5, 5, 5, ... (OEIS A114524). A plot of run lengths is shown above.
Berndt (1972) gave upper bounds of
![|gamma_n|<<span style=]() {(4(n-1)!)/(pi^n) for n even; (2(n-1)!)/(pi^n) for n odd. " src="http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation8.gif" style="height:82px; width:176px" /> |
(8)
|
However, these bounds are extremely weak. A stronger bound is given by
 |
(9)
|
for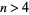 
(Matsuoka 1985).
Vacca (1910) proved that the Euler-Mascheroni constant may be expressed as
 |
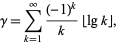(10)
|
where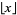 
is the floor function and the lg function 
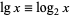is the logarithm to base 2. Hardy (1912) derived the formula
 |
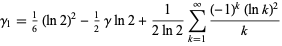(11)
|
from Vacca's expression.
Kluyver (1927) gave similar series for 
valid for all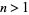 
,
 |
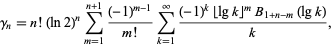(12)
|
where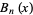 
is a Bernoulli polynomial. However, this series converges extremely slowly, requiring more than 
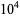terms to get two digits of 
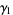and many more for higher order 
.
can also be expressed as a single sum using
 |
(13)
|
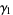also appears in the asymptotic expansion of the sum
 |
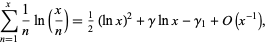(14)
|
where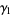 
was called 
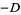and given incorrectly by Ellision and Mendès-France (1975) (and the error was subsequently reproduced by Le Lionnais 1983, p. 47). The exact form of (14) is given by
 |
(15)
|
where 
is a harmonic number and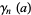 
is a generalized Stieltjes constant.
A set of constants related to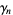 
is
![delta_n=lim_(m->infty)[sum_(k=1)^m(lnk)^n-int_1^m(lnx)^ndx-1/2(lnm)^n]](http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation16.gif) |
(16)
|
(Sitaramachandrarao 1986, Lehmer 1988).
The Stieltjes constants also satisfy the beautiful sum
![sum_(k=0)^infty(gamma_(k+n))/(k!)=(-1)^n[n!+zeta^((n))(0)]](http://mathworld.wolfram.com/images/equations/StieltjesConstants/NumberedEquation17.gif) |
(17)
|
(O. Marichev, pers. comm., 2008).
REFERENCES:
Berndt, B. C. "On the Hurwitz Zeta-Function." Rocky Mountain J. Math. 2, 151-157, 1972.
Bohman, J. and Fröberg, C.-E. "The Stieltjes Function--Definitions and Properties." Math. Comput. 51, 281-289, 1988.
Briggs, W. E. "Some Constants Associated with the Riemann Zeta-Function." Mich. Math. J. 3, 117-121, 1955-1956.
Coffey, M. W. "New Results on the Stieltjes Constants: Asymptotic and Exact Evaluation." J. Math. Anal. Appl. 317, 603-612, 2006.
Coffey, M. W. "New Summation Relations for the Stieltjes Constants." Proc. Roy. Soc. A 462, 2563-2573, 2006.
Ellison, W. J. and Mendès-France, M. Les nombres premiers. Paris: Hermann, 1975.
Finch, S. R. "Stieltjes Constants." §2.21 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 166-171, 2003.
Hardy, G. H. "Note on Dr. Vacca's Series for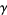 
." Quart. J. Pure Appl. Math. 43, 215-216, 1912.
Hardy, G. H. and Wright, E. M. "The Behavior of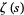 
when 
." §17.3 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 246-247, 1979.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, 2003.
Kluyver, J. C. "On Certain Series of Mr. Hardy." Quart. J. Pure Appl. Math. 50, 185-192, 1927.
Knopfmacher, J. "Generalised Euler Constants." Proc. Edinburgh Math. Soc. 21, 25-32, 1978.
Kreminski, R. "Newton-Cotes Integration for Approximating Stieltjes (Generalized Euler) Constants." Math. Comput. 72, 1379-1397, 2003.
Kreminski, R. "This Site Will Archive Some Stieltjes-Related Computational Work..." http://www.tamu-commerce.edu/math/FACULTY/KREMIN/stieltjesrelated/.
Kreminski, R. "This Page Displays Work in Progress by Rick Kreminski." http://www.tamu-commerce.edu/math/FACULTY/KREMIN/stieltjes/stieltjestestpage.html.
Kreminski, R. "Gammas 1 to 12 to 6900 Digits." http://www.tamu-commerce.edu/math/FACULTY/KREMIN/stieltjesrelated/gammas1to12/.
Lammel, E. "Ein Beweis dass die Riemannsche Zetafunktion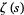 
is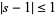 
keine Nullstelle besitzt." Univ. Nac. Tucmán Rev. Ser. A 16, 209-217, 1966.
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 47, 1983.
Lehmer, D. H. "The Sum of Like Powers of the Zeros of the Riemann Zeta Function." Math. Comput. 50, 265-273, 1988.
Liang, J. J. Y. and Todd, J. "The Stieltjes Constants." J. Res. Nat. Bur. Standards--Math. Sci. 76B, 161-178, 1972.
Matsuoka, Y. "Generalized Euler Constants Associated with the Riemann Zeta Function." In Number Theory and Combinatorics. Japan 1984 (Tokyo, Okayama and Kyoto, 1984). Singapore: World Scientific, pp. 279-295, 1985.
Plouffe, S. "Stieltjes Constants from 0 to 78, to 256 Digits Each." http://pi.lacim.uqam.ca/piDATA/stieltjesgamma.txt.
Sitaramachandrarao, R. "Maclaurin Coefficients of the Riemann Zeta Function." Abstracts Amer. Math. Soc. 7, 280, 1986.
Sloane, N. J. A. Sequences A001620/M3755, A082633, A086279, A086280, A086281, A086282, A114523, and A114524 in "The On-Line Encyclopedia of Integer Sequences."
Vacca, G. "A New Series for the Eulerian Constant." Quart. J. Pure Appl. Math. 41, 363-368, 1910.
الاكثر قراءة في نظرية الاعداد
اخر الاخبار
اخبار العتبة العباسية المقدسة
