

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Prime Gaps
المؤلف:
American Institute of Mathematics.
المصدر:
"Small Gaps between Consecutive Primes: Recent Work of D. Goldston and C. Yildirim." https://www.aimath.org/goldston_tech/.
الجزء والصفحة:
...
8-9-2020
1814
Prime Gaps
A prime gap of length 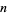 is a run of
is a run of  consecutive composite numbers between two successive primes. Therefore, the difference between two successive primes
consecutive composite numbers between two successive primes. Therefore, the difference between two successive primes 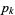 and
and 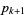 bounding a prime gap of length
bounding a prime gap of length 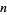 is
is 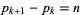 , where
, where 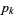 is the
is the  th prime number. Since the prime difference function
th prime number. Since the prime difference function
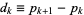 |
(1) |
is always even (except for 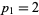 ), all primes gaps
), all primes gaps 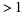 are also even. The notation
are also even. The notation 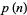 is commonly used to denote the smallest prime
is commonly used to denote the smallest prime 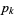 corresponding to the start of a prime gap of length
corresponding to the start of a prime gap of length 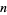 , i.e., such that
, i.e., such that 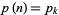 is prime,
is prime, 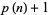 ,
, 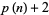 , ...,
, ...,  are all composite, and
are all composite, and  is prime (with the additional constraint that no smaller number satisfying these properties exists).
is prime (with the additional constraint that no smaller number satisfying these properties exists).
The maximal prime gap 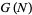 is the length of the largest prime gap that begins with a prime
is the length of the largest prime gap that begins with a prime 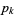 less than some maximum value
less than some maximum value  . For
. For 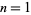 , 2, ...,
, 2, ..., 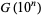 is given by 4, 8, 20, 36, 72, 114, 154, 220, 282, 354, 464, 540, 674, 804, 906, 1132, ... (OEIS A053303).
is given by 4, 8, 20, 36, 72, 114, 154, 220, 282, 354, 464, 540, 674, 804, 906, 1132, ... (OEIS A053303).
Arbitrarily large prime gaps exist. For example, for any  , the numbers
, the numbers  ,
,  , ...,
, ..., 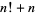 are all composite (Havil 2003, p. 170). However, no general method more sophisticated than an exhaustive search is known for the determination of first occurrences and maximal prime gaps (Nicely 1999).
are all composite (Havil 2003, p. 170). However, no general method more sophisticated than an exhaustive search is known for the determination of first occurrences and maximal prime gaps (Nicely 1999).
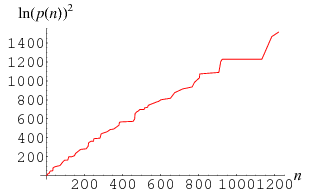
Cramér (1937) and Shanks (1964) conjectured that
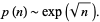 |
(2) |
Wolf conjectures a slightly different form
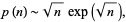 |
(3) |
which agrees better with numerical evidence.
Wolf conjectures that the maximal gap  between two consecutive primes less than
between two consecutive primes less than 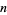 appears approximately at
appears approximately at
![G(n)∼n/(pi(n))[2lnpi(n)-lnn+ln(2C_2)]=g(n),](https://mathworld.wolfram.com/images/equations/PrimeGaps/NumberedEquation4.gif) |
(4) |
where 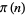 is the prime counting function and
is the prime counting function and 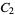 is the twin primes constant. Setting
is the twin primes constant. Setting 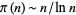 reduces to Cramer's conjecture for large
reduces to Cramer's conjecture for large  ,
,
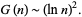 |
(5) |
It is known that there is a prime gap of length 803 following 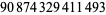 , and a prime gap of length
, and a prime gap of length 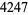 following
following 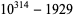 (Baugh and O'Hara 1992). H. Dubner (2001) discovered a prime gap of length
(Baugh and O'Hara 1992). H. Dubner (2001) discovered a prime gap of length 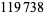 between two 3396-digit probable primes. On Jan. 15, 2004, J. K. Andersen and H. Rosenthal found a prime gap of length
between two 3396-digit probable primes. On Jan. 15, 2004, J. K. Andersen and H. Rosenthal found a prime gap of length 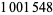 between two probabilistic primes of
between two probabilistic primes of 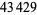 digits each. In January-May 2004, Hans Rosenthal and Jens Kruse Andersen found a prime gap of length
digits each. In January-May 2004, Hans Rosenthal and Jens Kruse Andersen found a prime gap of length 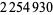 between two probabilistic primes with
between two probabilistic primes with 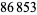 digits each (Anderson 2004).
digits each (Anderson 2004).
The merit of a prime gap compares the size of a gap to the local average gap, and is given by 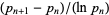 . In 1999, the number 1693182318746371 was found, with merit
. In 1999, the number 1693182318746371 was found, with merit 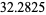 . This remained the record merit until 804212830686677669 was found in 2005, with a gap of 1442 and a merit of
. This remained the record merit until 804212830686677669 was found in 2005, with a gap of 1442 and a merit of  . Andersen maintains a list of the top 20 known merits. The prime gaps of increasing merit are 2, 3, 7, 113, 1129, 1327, 19609, ... (OEIS A111870).
. Andersen maintains a list of the top 20 known merits. The prime gaps of increasing merit are 2, 3, 7, 113, 1129, 1327, 19609, ... (OEIS A111870).
Young and Potler (1989) determined the first occurrences of prime gaps up to 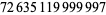 , with all first occurrences found between 1 and 673. Nicely (1999) has extended the list of maximal prime gaps. The following table gives the values of
, with all first occurrences found between 1 and 673. Nicely (1999) has extended the list of maximal prime gaps. The following table gives the values of 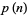 for small
for small 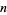 , omitting degenerate runs which are part of a run with greater
, omitting degenerate runs which are part of a run with greater 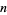 (OEIS A005250 and A002386).
(OEIS A005250 and A002386).
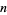 |
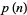 |
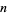 |
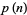 |
| 1 | 2 | 354 | 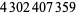 |
| 2 | 3 | 382 | 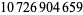 |
| 4 | 7 | 384 | 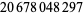 |
| 6 | 23 | 394 | 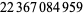 |
| 8 | 89 | 456 | 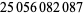 |
| 14 | 113 | 464 | 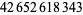 |
| 18 | 523 | 468 | 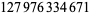 |
| 20 | 887 | 474 | 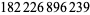 |
| 22 | 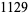 |
486 |  |
| 34 | 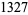 |
490 |  |
| 36 | 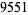 |
500 |  |
| 44 |  |
514 | 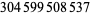 |
| 52 | 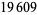 |
516 | 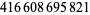 |
| 72 | 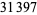 |
532 | 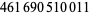 |
| 86 | 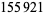 |
534 | 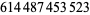 |
| 96 | 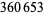 |
540 | 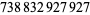 |
| 112 | 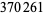 |
582 | 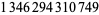 |
| 114 |  |
588 | 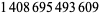 |
| 118 | 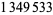 |
602 | 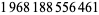 |
| 132 |  |
652 | 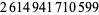 |
| 148 |  |
674 | 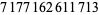 |
| 154 | 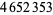 |
716 |  |
| 180 | 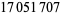 |
766 | 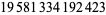 |
| 210 | 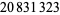 |
778 |  |
| 220 |  |
804 | 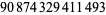 |
| 222 | 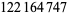 |
806 | 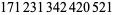 |
| 234 |  |
906 | 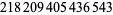 |
| 248 | 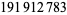 |
916 | 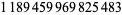 |
| 250 | 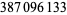 |
924 | 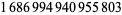 |
| 282 | 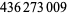 |
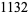 |
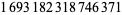 |
| 288 | 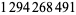 |
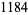 |
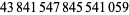 |
| 292 | 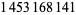 |
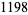 |
 |
| 320 |  |
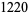 |
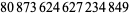 |
| 336 | 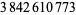 |
Define
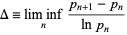 |
(6) |
as the infimum limit of the ratio of the 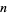 th prime difference to the natural logarithm of the
th prime difference to the natural logarithm of the 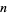 th prime number. If there are an infinite number of twin primes, then
th prime number. If there are an infinite number of twin primes, then 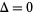 . This follows since it must then be true that
. This follows since it must then be true that 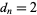 infinitely often, say at
infinitely often, say at 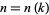 for
for  , 2, ..., so a necessary condition for the twin prime conjecture to hold is that
, 2, ..., so a necessary condition for the twin prime conjecture to hold is that
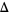 |
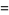 |
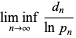 |
(7) |
 |
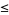 |
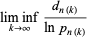 |
(8) |
 |
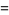 |
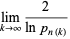 |
(9) |
 |
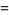 |
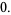 |
(10) |
However, this condition is not sufficient, since the same proof works if 2 is replaced by any constant.
Hardy and Littlewood showed in 1926 that, subject to the truth of the generalized Riemann hypothesis, 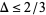 . This was subsequently improved by Rankin (again assuming the generalized Riemann hypothesis) to
. This was subsequently improved by Rankin (again assuming the generalized Riemann hypothesis) to 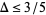 . In 1940, Erdős used sieve theory to show for the first time with no assumptions that
. In 1940, Erdős used sieve theory to show for the first time with no assumptions that 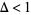 . This was subsequently improved to 15/16 (Ricci),
. This was subsequently improved to 15/16 (Ricci), 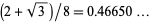 (Bombieri and Davenport 1966), and
(Bombieri and Davenport 1966), and  (Pil'Tai 1972), as quoted in Le Lionnais (1983, p. 26). Huxley (1973, 1977) obtained
(Pil'Tai 1972), as quoted in Le Lionnais (1983, p. 26). Huxley (1973, 1977) obtained 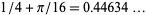 , which was improved by Maier in 1986 to
, which was improved by Maier in 1986 to 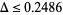 , which was the best result known until 2003 (American Institute of Mathematics).
, which was the best result known until 2003 (American Institute of Mathematics).
At a March 2003 meeting on elementary and analytic number theory in Oberwolfach, Germany, Goldston and Yildirim presented an attempted proof that 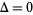 . While the original proof turned out to be flawed (Mackenzie 2003ab), the result has now been established by a new proof (American Institute of Mathematics 2005, Cipra 2005, Devlin 2005, Goldston et al. 2005ab).
. While the original proof turned out to be flawed (Mackenzie 2003ab), the result has now been established by a new proof (American Institute of Mathematics 2005, Cipra 2005, Devlin 2005, Goldston et al. 2005ab).
REFERENCES:
American Institute of Mathematics. "Small Gaps between Consecutive Primes: Recent Work of D. Goldston and C. Yildirim." https://www.aimath.org/goldston_tech/.
American Institute of Mathematics. "Breakthrough in Prime Number Theory." May 24, 2005. https://aimath.org/.
Andersen, J. K. "First Known Prime Megagap." https://hjem.get2net.dk/jka/math/primegaps/megagap.htm.
Andersen, J. K. "Largest Known Prime Gap." https://hjem.get2net.dk/jka/math/primegaps/megagap2.htm.
Andersen, J. K. "A Prime Gap of 1001548." 15 Jan 2004. https://listserv.nodak.edu/scripts/wa.exe?A2=ind0401&L=nmbrthry&F=&S=&P=397.
Andersen, J. K. "A Prime Gap of 2254930." 2 Jun 2004. https://listserv.nodak.edu/cgi-bin/wa.exe?A2=ind0406&L=nmbrthry&P=601.
Andersen, J. K. "Top-20 Prime Gaps." https://hjem.get2net.dk/jka/math/primegaps/gaps20.htm.
Baugh, D. and O'Hara, F. "Large Prime Gaps." J. Recr. Math. 24, 186-187, 1992.
Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 133-134, 1994.
Bombieri, E. and Davenport, H. "Small Differences between Prime Numbers." Proc. Roy. Soc. A 293, 1-18, 1966.
Brent, R. P. "The First Occurrence of Large Gaps between Successive Primes." Math. Comput. 27, 959-963, 1973.
Brent, R. P. "The Distribution of Small Gaps between Successive Primes." Math. Comput. 28, 315-324, 1974.
Brent, R. P. "The First Occurrence of Certain Large Prime Gaps." Math. Comput. 35, 1435-1436, 1980.
Caldwell, C. "The Gaps Between Primes." https://primes.utm.edu/notes/gaps.html.
Cipra, B. "Proof Promises Progress in Prime Progressions." Science 304, 1095, 2004.
Cipra, B. "Third Time Proves Charm for Prime-Gap Theorem." Science 308, 1238, 2005.
Cramér, H. "On the Order of Magnitude of the Difference between Consecutive Prime Numbers." Acta Arith. 2, 23-46, 1937.
Cutter, P. A. "Finding Prime Pairs with Particular Gaps." Math. Comput. 70, 1737-1744, 2001.
Devlin, K. "Major Advance on the Twin Primes Conjecture." May 24, 2005. https://www.maa.org/news/052505twinprimes.html.
Dubner, H. "New Large Prime Gap Record." 13 Dec 2001. https://listserv.nodak.edu/scripts/wa.exe?A2=ind0112&L=nmbrthry&P=1093.
Fouvry, É. "Autour du théorème de Bombieri-Vinogradov." Acta. Math. 152, 219-244, 1984.
Fouvry, É. and Grupp, F. "On the Switching Principle in Sieve Theory." J. reine angew. Math. 370, 101-126, 1986.
Fouvry, É. and Iwaniec, H. "Primes in Arithmetic Progression." Acta Arith. 42, 197-218, 1983.
Goldston, D. A.; Graham, S. W.; Pintz, J.; and Yildirim, C. Y. "Small Gaps between Primes or Almost Primes." Jun. 3, 2005a. https://www.arxiv.org/abs/math.NT/0506067/.
Goldston, D. A.; Motohashi, Y.; Pintz, J.; and Yildirim, C. Y. "Small Gaps between Primes Exist." May 14, 2005b. https://www.arxiv.org/abs/math.NT/0505300/.
Guy, R. K. "Gaps between Primes. Twin Primes" and "Increasing and Decreasing Gaps." §A8 and A11 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 19-23 and 26-27, 1994.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, 2003.
Huxley, M. N. "Small Differences between Consecutive Primes." Mathematica 20, 229-232, 1973.
Huxley, M. N. "Small Differences between Consecutive Primes. II." Mathematica 24, 142-152, 1977.
Lander, L. J. and Parkin, T. R. "On First Appearance of Prime Differences." Math. Comput. 21, 483-488, 1967.
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, 1983.
Mackenzie, D. "Prime Proof Helps Mathematicians Mind the Gaps." Science 300, 32, 2003a.
Mackenzie, D. "Prime-Number Proof's Leap Falls Short." Science 300, 1066, 2003b.
Montgomery, H. "Small Gaps Between Primes." 13 Mar 2003. https://listserv.nodak.edu/scripts/wa.exe?A2=ind0303&L=nmbrthry&P=1323.
Nicely, T. R. "First Occurrence Prime Gaps." https://www.trnicely.net/gaps/gaplist.html.
Nicely, T. R. "New Maximal Prime Gaps and First Occurrences." Math. Comput. 68, 1311-1315, 1999. https://www.trnicely.net/gaps/gaps.html.
Nicely, T. R. and Nyman, B. "First Occurrence of a Prime Gap of 1000 or Greater." https://www.trnicely.net/gaps/gaps2.html.
Nyman, B. and Nicely, T. R. "New Prime Gaps Between 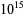 and
and 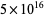 ." J. Int. Seq. 6, 1-6, 2003.
." J. Int. Seq. 6, 1-6, 2003.
Rivera, C. "Problems & Puzzles: Puzzle 011-Distinct, Increasing & Decreasing Gaps." https://www.primepuzzles.net/puzzles/puzz_011.htm.
Shanks, D. "On Maximal Gaps between Successive Primes." Math. Comput. 18, 646-651, 1964.
Sloane, N. J. A. Sequences A002386/M0858, A008996, A008950, A008995, A008996, A030296, A053303, and A111870 in "The On-Line Encyclopedia of Integer Sequences."
Soundararajan, K. "Small Gaps Between Prime Numbers: The Work of Goldston-Pintz-Yildirim." Bull. Amer. Math. Soc. 44, 1-18, 2007.
Wolf, M. "First Occurrence of a Given Gap between Consecutive Primes." https://www.ift.uni.wroc.pl/~mwolf/.
Wolf, M. "Some Conjectures on the Gaps Between Consecutive Primes." https://www.ift.uni.wroc.pl/~mwolf/.
Young, J. and Potler, A. "First Occurrence Prime Gaps." Math. Comput. 52, 221-224, 1989.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















