

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Prime Formulas
المؤلف:
Conway, J. H. and Guy, R. K
المصدر:
The Book of Numbers. New York: Springer-Verlag
الجزء والصفحة:
...
19-9-2020
3584
Prime Formulas
There exist a variety of formulas for either producing the 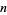 th prime as a function of
th prime as a function of  or taking on only prime values. However, all such formulas require either extremely accurate knowledge of some unknown constant, or effectively require knowledge of the primes ahead of time in order to use the formula (Dudley 1969; Ribenboim 1996, p. 186). There also exist simple prime-generating polynomials that generate only primes for the first (possibly large) number of integer values.
or taking on only prime values. However, all such formulas require either extremely accurate knowledge of some unknown constant, or effectively require knowledge of the primes ahead of time in order to use the formula (Dudley 1969; Ribenboim 1996, p. 186). There also exist simple prime-generating polynomials that generate only primes for the first (possibly large) number of integer values.
There are also many beautiful formulas involving prime sums and prime products that can be done in closed form.
Considering examples of formulas that produce only prime numbers (although not necessarily the complete set of prime numbers  ), there exists a constant
), there exists a constant 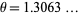 (OEIS A051021) known as Mills' constant such that
(OEIS A051021) known as Mills' constant such that
 |
(1) |
where 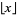 is the floor function, is prime for all
is the floor function, is prime for all 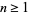 (Ribenboim 1996, p. 186). The first few values of
(Ribenboim 1996, p. 186). The first few values of 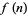 are 2, 11, 1361, 2521008887, ... (OEIS A051254). It is not known if
are 2, 11, 1361, 2521008887, ... (OEIS A051254). It is not known if 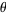 is irrational. There also exists a constant
is irrational. There also exists a constant  (OEIS A086238) such that
(OEIS A086238) such that
 |
(2) |
(Wright 1951; Ribenboim 1996, p. 186) is prime for every 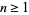 . The first few values of
. The first few values of 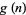 are 3, 13, 16381, ... (OEIS A016104). In the case of both
are 3, 13, 16381, ... (OEIS A016104). In the case of both 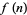 and
and 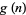 , the numbers at
, the numbers at  grow so rapidly that an extremely precise value of
grow so rapidly that an extremely precise value of 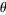 or
or 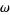 is needed in order to obtain the correct value, and values for
is needed in order to obtain the correct value, and values for 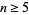 are effectively incomputable.
are effectively incomputable.
Explicit formulas exist for the 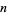 th prime both as a function of
th prime both as a function of 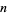 and in terms of the primes 2, ...,
and in terms of the primes 2, ...,  (Hardy and Wright 1979, pp. 5-6, 344-345, and 414; Guy 1994, pp. 36-41), a number of which are given below. However, it should again be emphasized that these formulas are extremely inefficient, and in many (if not all) cases, simply performing an efficient sieving would yield the primes much more quickly and efficiently.
(Hardy and Wright 1979, pp. 5-6, 344-345, and 414; Guy 1994, pp. 36-41), a number of which are given below. However, it should again be emphasized that these formulas are extremely inefficient, and in many (if not all) cases, simply performing an efficient sieving would yield the primes much more quickly and efficiently.
Let
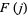 |
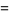 |
![|_cos^2[pi((j-1)!+1)/j]_|](https://mathworld.wolfram.com/images/equations/PrimeFormulas/Inline23.gif) |
(3) |
 |
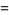 |
(4) |
for  an integer, where
an integer, where 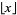 is again the floor function. This formula is a consequence of Wilson's theorem and conceals the prime numbers
is again the floor function. This formula is a consequence of Wilson's theorem and conceals the prime numbers 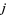 as those for which
as those for which 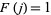 , i.e., the values of
, i.e., the values of 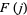 are 1, 1, 1, 0, 1, 0, 1, 0, 0, 0, 1, ... (OEIS A080339). Then
are 1, 1, 1, 0, 1, 0, 1, 0, 0, 0, 1, ... (OEIS A080339). Then
 |
(5) |
and
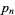 |
 |
 |
(6) |
 |
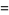 |
 |
(7) |
where 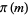 is the prime counting function (Willans 1964; Havil 2003, pp. 168-169).
is the prime counting function (Willans 1964; Havil 2003, pp. 168-169).
Gandhi gave the formula in which 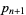 is the unique integer such that
is the unique integer such that
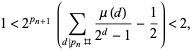 |
(8) |
where  is the primorial function (Gandhi 1971, Eynden 1972, Golomb 1974) and
is the primorial function (Gandhi 1971, Eynden 1972, Golomb 1974) and 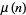 is the Möbius function. It is also true that
is the Möbius function. It is also true that
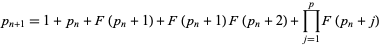 |
(9) |
(Ribenboim 1996, pp. 180-182). Note that the number of terms in the summation to obtain the 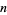 th prime is
th prime is  , so these formulas turn out not to be practical in the study of primes.
, so these formulas turn out not to be practical in the study of primes.
Hardy and Wright (1979, p. 414) give the formula
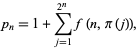 |
(10) |
for 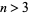 , where
, where
|
(11) |
and an "elementary" formula for the prime counting function is given by
![pi(n)=-1+sum_(j=3)^n[(j-2)!-j|_((j-2)!)/j_|]](https://mathworld.wolfram.com/images/equations/PrimeFormulas/NumberedEquation8.gif) |
(12) |
(correcting a sign error), where 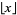 is the floor function.
is the floor function.
A double sum for the 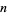 th prime
th prime 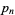 is
is
![p_n=1+sum_(k=1)^(2(|_nlnn_|+1))[1-|_(sum_(j=2)^(k)1+|_s(j)_|)/n_|],](https://mathworld.wolfram.com/images/equations/PrimeFormulas/NumberedEquation9.gif) |
(13) |
where
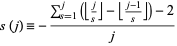 |
(14) |
(Ruiz 2000).
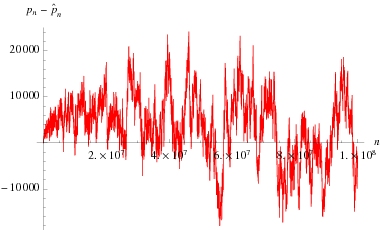
An asymptotic formula for 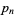 is given by
is given by
 |
(15) |
(Cipolla 1902). This asymptotic expansion is the inverse of the logarithmic integral 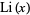 obtained by series reversion, where the inversion of
obtained by series reversion, where the inversion of 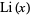 gives
gives 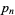 because the prime number theorem says that
because the prime number theorem says that 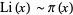 , where
, where 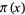 is the prime counting function and the inverse of
is the prime counting function and the inverse of 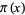 is
is 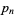 in the sense that
in the sense that 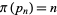 . However, the formula oscillates a great deal as illustrated above, where
. However, the formula oscillates a great deal as illustrated above, where 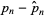 is the difference between the actual
is the difference between the actual  th prime and that given by the Cipolla formula. Interestingly, truncating at
th prime and that given by the Cipolla formula. Interestingly, truncating at  gives the refined form of Rosser's theorem, which is a strict inequality on
gives the refined form of Rosser's theorem, which is a strict inequality on 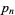 . Salvy (1994) deals with more general cases.
. Salvy (1994) deals with more general cases.

B. M. Bredihin proved that
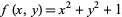 |
(16) |
takes prime values for infinitely many integral pairs 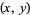 (Honsberger 1976, p. 30). For example,
(Honsberger 1976, p. 30). For example, 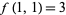 ,
, 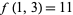 ,
, 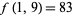 , and so on. The primes of this form are 3, 11, 19, 41, 53, 59, 73, 83, 101, 107, 131, 137, 149, 163, ... (OEIS A079544; Mitrinović and Sándor 1995, p. 11). The values of
, and so on. The primes of this form are 3, 11, 19, 41, 53, 59, 73, 83, 101, 107, 131, 137, 149, 163, ... (OEIS A079544; Mitrinović and Sándor 1995, p. 11). The values of  and
and 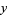 for which
for which 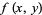 is prime are plotted above, showing some interesting patterns.
is prime are plotted above, showing some interesting patterns.
It is in general not difficult to artificially construct formulas that always generate prime numbers. For example, consider the formula
![f(x,y)=1/2(y-1)[|B^2(x,y)-1|-(B^2(x,y)-1)]+2,](https://mathworld.wolfram.com/images/equations/PrimeFormulas/NumberedEquation13.gif) |
(17) |
where
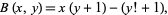 |
(18) |
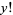 is the factorial, and
is the factorial, and  and
and 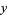 are positive integers (Honsberger 1976, p. 33). This will always have
are positive integers (Honsberger 1976, p. 33). This will always have 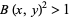 and hence yield the value 2 unless
and hence yield the value 2 unless ![x=[(p-1)!+1]/p](https://mathworld.wolfram.com/images/equations/PrimeFormulas/Inline72.gif) and
and 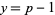 , in which case it simplifies to
, in which case it simplifies to
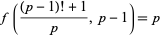 |
(19) |
The formula therefore generates odd primes exactly once each (Honsberger 1976, p. 33) at the values for which the Wilson quotient ![x=[(p-1)!+1]/p](https://mathworld.wolfram.com/images/equations/PrimeFormulas/Inline74.gif) is an integer, i.e., 1, 1, 5, 103, 329891, 36846277, 1230752346353, ... (OEIS A007619).
is an integer, i.e., 1, 1, 5, 103, 329891, 36846277, 1230752346353, ... (OEIS A007619).
The FRACTRAN game (Guy 1983, Conway and Guy 1996, p. 147) provides an unexpected means of generating the prime numbers based on 14 fractions, but it is actually just a concealed version of a sieve.
REFERENCES:
Cipolla, M. ""La determinazione assintotica dell' numero primo." Napoli Rend. 3, 132-166, 1902.
numero primo." Napoli Rend. 3, 132-166, 1902.
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 130 and 147, 1996.
Dudley, U. "History of Formula for Primes." Amer. Math. Monthly 76, 23-28, 1969.
Dusart, P. "The  Prime is Greater than
Prime is Greater than  for
for  ." Math. Comput. 68, 411-415, 1999.
." Math. Comput. 68, 411-415, 1999.
Eynden, C. V. "A Proof of Gandhi's Formula for the 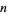 th Prime." Amer. Math. Monthly 79, 625, 1972.
th Prime." Amer. Math. Monthly 79, 625, 1972.
Finch, S. R. "Hardy-Littlewood Constants." §2.1 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 84-94, 2003.
Gandhi, J. M. "Formulae for the  th Prime." Proc. Washington State University Conferences on Number Theory. pp. 96-107, 1971.
th Prime." Proc. Washington State University Conferences on Number Theory. pp. 96-107, 1971.
Gardner, M. "Patterns and Primes." Ch. 9 in The Sixth Book of Mathematical Games from Scientific American. Chicago, IL: University of Chicago Press, pp. 79-90, 1984.
Golomb, S. W. "A Direct Interpretation of Gandhi's Formula." Amer. Math. Monthly 81, 752-754, 1974.
Guy, R. K. "Conway's Prime Producing Machine." Math. Mag. 56, 26-33, 1983.
Guy, R. K. "Prime Numbers," "Formulas for Primes," and "Products Taken over Primes." Ch. A, §A17, and §B48 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 3-43, 36-41, and 102-103, 1994.
Hardy, G. H. and Wright, E. M. "Prime Numbers" and "The Sequence of Primes." §1.2 and 1.4 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 1-4, 5-6, 344-345, and 414, 1979.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, 2003.
Honsberger, R. Mathematical Gems II. Washington, DC: Math. Assoc. Amer., 1976.
Mills, W. H. "A Prime-Representing Function." Bull. Amer. Math. Soc. 53, 604, 1947.
Mitrinović, D. S. and Sándor, J. Handbook of Number Theory. Dordrecht, Netherlands: Kluwer, 1995.
Ribenboim, P. The New Book of Prime Number Records. New York: Springer-Verlag, 1996.
Ruiz, S. M. "The General Term of the Prime Number Sequence and the Smarandache Prime Function." Smarandache Notions J. 11, 59-61, 2000.
Salvy, B. "Fast Computation of Some Asymptotic Functional Inverses." J. Symb. Comput. 17, 227-236, 1994.
Sloane, N. J. A. Sequences A007619/M4023, A016104, A051021, A051254, A079544, A080339, and A086238 in "The On-Line Encyclopedia of Integer Sequences."
Willans, C. P. "A Formula for the 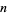 th Prime Number." Math. Gaz. 48, 413-415, 1964.
th Prime Number." Math. Gaz. 48, 413-415, 1964.
Wright, E. M. "A Prime-Representing Function." Amer. Math. Monthly 58, 616-618, 1951.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















