

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Representations and Crossings
المؤلف:
W.D. Wallis
المصدر:
Mathematics in the Real World
الجزء والصفحة:
118-119
12-2-2016
2342
Consider these two diagrams.
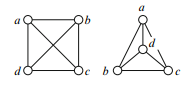
They represent the same graph, the complete graph K4 on four vertices. But as diagrams they are quite different: in the left-hand version, two edges cross; in theright-hand diagram there are no crossings. We shall refer to the two diagrams as different representations of K4. The crossing number of a representation is the number of different pairs of edges that cross. A graph that can be drawn without any crossings is called planar and a drawing of a graph with no crossings is called a planar representation. For example, K4 is planar and the right-hand drawing is a planar representation of K4.
Sample Problem 1.1 Show that the crossing number of the complete graph K5on five vertices is 1.
Solution. We can think of K5 as being K4 with one more vertex added. If we start with the representation shown in the left-hand of the diagram, we certainly get at least one crossing, and it is not hard to avoid further crossings. So the crossing number of K5 is either 0 or 1. To obtain a planar representation of K5 we would have to add a new vertex, e say, to the right-hand diagram. But if the new vertex is inside the old diagram, at least one new crossing will be introduced— for example, if it is inside the triangle bcd, edge ae must cross one of the original edges—and if e is outside the original diagram, de must cross another edge. The following diagrams illustrate these two cases.
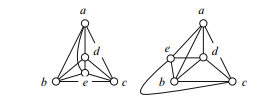
So K5 has crossing number 1.
It is easy to see that any tree has crossing number 0. Similarly, any cycle can be drawn without crossings.
There are many applications of crossing numbers. An early use was in the design of railway yards; it is inconvenient to have the different lines crossing, and sometimes it is preferable to have longer track rather than extra intersections. An obvious extension of this idea is freeway design. At a complex intersection, fewer crossings will mean fewer expensive flyover bridges. More recently, small crossing numbers have proven important in the design of VLSI chips; if two parts of a circuit are not to be connected electrically, but they cross, a costly insulation process is necessary.
 الاكثر قراءة في نظرية البيان
الاكثر قراءة في نظرية البيان
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















