
DECOMPOSITION AND SPECIAL GRAPHS
 المؤلف:
Douglas B. West
المؤلف:
Douglas B. West
 المصدر:
Introduction to Graph Theory Second Edition
المصدر:
Introduction to Graph Theory Second Edition
 الجزء والصفحة:
8-10
الجزء والصفحة:
8-10
 2-8-2016
2-8-2016
 2092
2092
The example 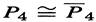 suggests a family of graph problems.
suggests a family of graph problems.
1.1. Definition.
A graph is self-complementary if it is isomorphic to its complement. A decomposition of a graph is a list of subgraphs such that
each edge appears in exactly one subgraph in the list.
An n-vertex graph H is self-complementary if and only if Kn has a decomposition consisting of two copies of H.
1.2 Example.
We can decompose K5 into two 5-cycles, and thus the 5-cycle is self-complementary. Any n-vertex graph and its complement decompose Kn.
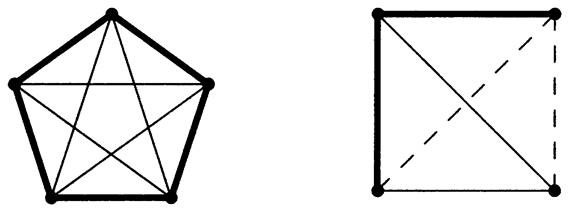
Also Kl,n-l and Kn-l. decompose Kn, even though one of these subgraphs omits a vertex. On the right below we show a decomposition of K4
using three copies consider graph decompositions. .
1.3 Example.
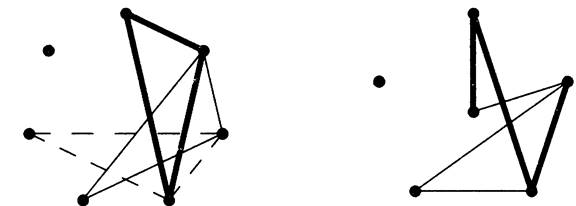
The question of which complete graphs decompose into copies of K3 is a fundamental question in the theory of combinatorial designs.
On the left below we suggest such a decomposition for K7. Rotating the triangle through seven positions uses each edge exactly once.
On the right we suggest a decomposition of K6 into copies of P4. Placing one vertex in the center groups the edges into three types: the outer 5-cycle, the inner (crossing) 5-cycle on those vertices, and the edges involving the central vertex. Each 4-vertex path in the decomposition uses one edge of each type; we rotate the picture to get the next path.
We referred to a copy of K3 as a triangle. Short names for graphs that arise frequently in structural discussions can be convenient.
1.4. Definition.
The Petersen graph is the simple graph whose vertices are the 2-element subsets of a 5-element set and whose edges are the pairs
of disjoint 2-element subsets.
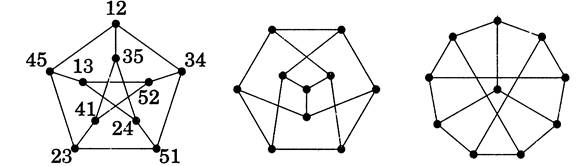
We have drawn the Petersen graph in three ways above. It is a useful example so often that an entire book was devoted to it (Holton-Sheell an [I993]).
Its properties follow from the statement of its adjacency relation that we have used as the definition.
1.5. Proposition.
If two vertices are nonadjacent in the Petersen graph, then they have exactly one common neighbor.
proof.
Non adjacent vertices are 2-sets sharing one element; their union S has size 3. A vertex adjacent to both IS a 2-set disjoint from both. Since the 2-sets
are chosen from {1, 2, 3,4, 5}, there is exactly one 2-set disjoint from S.
1.6 Definition.
The girth of a graph with a cycle is the length of its shortest cycle. A graph with no cycle has infinite girth.
1.7 Definition.
An automorphism of G is an isomorphism from G to G. A graph G is vertex-transitive if for every pair u, v ϵ V (G) there is an automorphism that maps u to v.
The automorphisms of G are the permutations of V (G) that can be applied to both the rows and the columns of A(G) without changing A(G).
Introduction to Graph Theory Second Edition, Douglas B. West , Indian Reprint, 2002,page(8.10)
 الاكثر قراءة في نظرية البيان
الاكثر قراءة في نظرية البيان
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


