
Space-time intervals
 المؤلف:
Richard Feynman, Robert Leighton and Matthew Sands
المؤلف:
Richard Feynman, Robert Leighton and Matthew Sands
 المصدر:
The Feynman Lectures on Physics
المصدر:
The Feynman Lectures on Physics
 الجزء والصفحة:
Volume I, Chapter 17
الجزء والصفحة:
Volume I, Chapter 17
 2024-02-27
2024-02-27
 2135
2135
Although the geometry of space-time is not Euclidean in the ordinary sense, there is a geometry which is very similar, but peculiar in certain respects. If this idea of geometry is right, there ought to be some functions of coordinates and time which are independent of the coordinate system. For example, under ordinary rotations, if we take two points, one at the origin, for simplicity, and the other one somewhere else, both systems would have the same origin, and the distance from here to the other point is the same in both. That is one property that is independent of the particular way of measuring it. The square of the distance is x2+y2+z2. Now what about space-time? It is not hard to demonstrate that we have here, also, something which stays the same, namely, the combination c2t2−x2−y2−z2 is the same before and after the transformation:

This quantity is therefore something which, like the distance, is “real” in some sense; it is called the interval between the two space-time points, one of which is, in this case, at the origin. (Actually, of course, it is the interval squared, just as x2+y2+z2 is the distance squared.) We give it a different name because it is in a different geometry, but the interesting thing is only that some signs are reversed and there is a c in it.
Let us get rid of the c; that is an absurdity if we are going to have a wonderful space with x’s and y’s that can be interchanged. One of the confusions that could be caused by someone with no experience would be to measure widths, say, by the angle subtended at the eye, and measure depth in a different way, like the strain on the muscles needed to focus them, so that the depths would be measured in feet and the widths in meters. Then one would get an enormously complicated mess of equations in making transformations such as (17.2), and would not be able to see the clarity and simplicity of the thing for a very simple technical reason, that the same thing is being measured in two different units. Now in Eqs. (17.1) and (17.3) nature is telling us that time and space are equivalent; time becomes space; they should be measured in the same units. What distance is a “second”? It is easy to figure out from (17.3) what it is. It is 3×108 meters, the distance that light would go in one second. In other words, if we were to measure all distances and times in the same units, seconds, then our unit of distance would be 3×108 meters, and the equations would be simpler. Or another way that we could make the units equal is to measure time in meters. What is a meter of time? A meter of time is the time it takes for light to go one meter, and is therefore 1/3×10−8 sec, or 3.3 billionths of a second! We would like, in other words, to put all our equations in a system of units in which c=1. If time and space are measured in the same units, as suggested, then the equations are obviously much simplified. They are
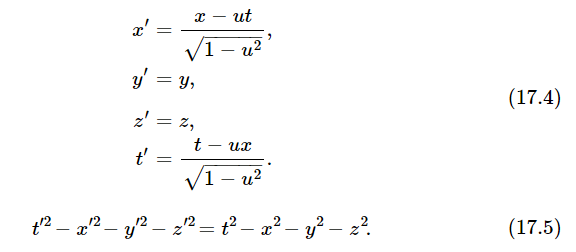
If we are ever unsure or “frightened” that after we have this system with c=1 we shall never be able to get our equations right again, the answer is quite the opposite. It is much easier to remember them without the c’s in them, and it is always easy to put the c’s back, by looking after the dimensions. For instance, in  we know that we cannot subtract a velocity squared, which has units, from the pure number 1, so we know that we must divide u2 by c2 in order to make that unitless, and that is the way it goes.
we know that we cannot subtract a velocity squared, which has units, from the pure number 1, so we know that we must divide u2 by c2 in order to make that unitless, and that is the way it goes.
The difference between space-time and ordinary space, and the character of an interval as related to the distance, is very interesting. According to formula (17.5), if we consider a point which in a given coordinate system had zero time, and only space, then the interval squared would be negative and we would have an imaginary interval, the square root of a negative number. Intervals can be either real or imaginary in the theory. The square of an interval may be either positive or negative, unlike distance, which has a positive square. When an interval is imaginary, we say that the two points have a space-like interval between them (instead of imaginary), because the interval is more like space than like time. On the other hand, if two objects are at the same place in a given coordinate system, but differ only in time, then the square of the time is positive and the distances are zero and the interval squared is positive; this is called a time-like interval. In our diagram of space-time, therefore, we would have a representation something like this: at 45∘ there are two lines (actually, in four dimensions these will be “cones,” called light cones) and points on these lines are all at zero interval from the origin. Where light goes from a given point is always separated from it by a zero interval, as we see from Eq. (17.5). Incidentally, we have just proved that if light travels with speed c in one system, it travels with speed c in another, for if the interval is the same in both systems, i.e., zero in one and zero in the other, then to state that the propagation speed of light is invariant is the same as saying that the interval is zero.
 الاكثر قراءة في النظرية النسبية العامة
الاكثر قراءة في النظرية النسبية العامة
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


