

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Exponential Functions
المؤلف:
المرجع الالكتروني للمعلوماتيه
المصدر:
www.almerja.com
الجزء والصفحة:
...
4-3-2017
2509
What is an exponential function?
An exponential function is a mathematical expression in which a variable represents the exponent of an expression.
What does an exponential function look like?
Here's a very simple exponential function:
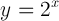
That equation is read as "y equals 2 to the x power."
Exponent refresher:
Let's remember how exponents work. Suppose we have the equation below:

That equation tells us to multiply x by itself to get y. It's the equivalent of:

If we want to find y when x=3, we can pretty quickly find that y=3*3=9. But, this is actually what's known as a "power function". In fact, it's just a polynomial, and not an exponential function at all.
Take a closer look at x2 . This means x squared or x to the second power. What does it mean? There are two parts to this exponential term:
1) An exponent, which is the number 2.
2) A base, which is the variable x.
With exponential functions, the variable will actually be the exponent, with a constant as the base.
Exponential Functions
Here's what exponential functions look like:
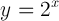
The equation is y equals 2 raised to the x power. This sort of equation represents what we call "exponential growth" or "exponential decay." Other examples of exponential functions include:

The general exponential function looks like this:  , where the base b is any positive constant. The base b could be 1, but remember that 1 to any power is just 1, so it's a particularly boring exponential function!
, where the base b is any positive constant. The base b could be 1, but remember that 1 to any power is just 1, so it's a particularly boring exponential function!
Let's try some examples:
Example 1
Solve for x: 
This one is actually pretty simple, so let's just think it through:
The problem says we have to multiply x number of two's together to get four. Well, everyone knows that 2*2=4, so the answer is two:

Ok, great, that was an easy example. But you can see where this could get really, hard, right? Look at this:
Example 2
Solve for y when x=5.

That means we need to plug-in x=5 and see what we get:
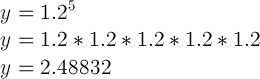
Fortunately all we had to do with this problem was multiply 1.2 times itself a few times to get the answer.
What about a word problem example?
We can use a formula for exponential growth to model the population of a bacteria. Let's say the bacteria population is defined by 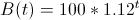 where B is the total population and t represents time in hours. While that may look complicated, it really tells us that the bacteria grows by 12 percent every hour. Every time another hour goes by, t goes up by 1, so we have to multiply the population times 1.12 again. The 100 simply sets the initial population at time t=0.
where B is the total population and t represents time in hours. While that may look complicated, it really tells us that the bacteria grows by 12 percent every hour. Every time another hour goes by, t goes up by 1, so we have to multiply the population times 1.12 again. The 100 simply sets the initial population at time t=0.
So, how much bacteria remains after 4 hours?
What do we know? We have the formula  and the fact that t=4.
and the fact that t=4.
Replace t with 4 hours in the formula above and simplify.
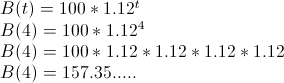
 الاكثر قراءة في مواضيع عامة في الجبر
الاكثر قراءة في مواضيع عامة في الجبر
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















