

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Chaitin,s Constant
المؤلف:
Borwein, J. and Bailey, D
المصدر:
Mathematics by Experiment: Plausible Reasoning in the 21st Century. Wellesley, MA: A K Peters
الجزء والصفحة:
...
20-2-2020
2698
Chaitin's Constant
A Chaitin's constant, also called a Chaitin omega number, introduced by Chaitin (1975), is the halting probability of a universal prefix-free (self-delimiting) Turing machine. Every Chaitin constant is simultaneously computably enumerable (the limit of a computable, increasing, converging sequence of rationals), and algorithmically random (its binary expansion is an algorithmic random sequence), hence uncomputable (Chaitin 1975).
A Chaitin's constant can therefore be defined as
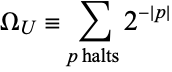 |
(1) |
which gives the probability that for any set of instructions, a particular prefix-free universal Turing machine will halt, where 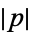 is the size in bits of program
is the size in bits of program 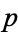 .
.
The value of a Chaitin constant is highly machine-dependent. In some cases, it can even be proved that not a single bit can be computed (Solovay 2000).
Chaitin constants 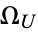 are perhaps the most obvious specific example of uncomputable numbers. They are also known to be transcendental.
are perhaps the most obvious specific example of uncomputable numbers. They are also known to be transcendental.
Calude et al. (2002) computed the first 64 bits of Chaitin's constant 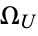 for a certain universal Turing machine as
for a certain universal Turing machine as
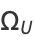 |
 |
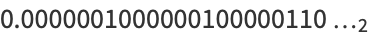 |
(2) |
 |
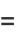 |
 |
(3) |
(OEIS A079365 and A100264).

Calude and Dinneen (2007) subsequently computed the first 43 and 40 bits of another prefix-free Turing machine which is universal when used with data in base 16 and 2, respectively, as
 |
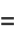 |
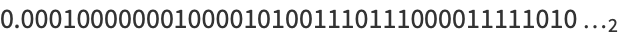 |
(4) |
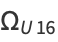 |
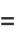 |
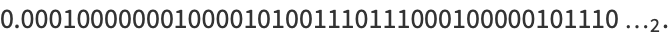 |
(5) |
The binary result is engraved on the medallion presented to Gregory Chaitin for his 60th birthday by Stephen Wolfram, illustrated above.
REFERENCES:
Borwein, J. and Bailey, D. Mathematics by Experiment: Plausible Reasoning in the 21st Century. Wellesley, MA: A K Peters, p. 145, 2003.
Calude, C. S.; Dinneen, M. J.; and Shu, C.-K. "Computing a Glimpse of Randomness." Exper. Math. 11, 361-370, 2002.
Calude, C. S. and Dinneen, M. J. "Exact Approximations of Omega Numbers." Int. J. Bifur. Chaos 17, 1937-1954, 2007.
Chaitin, G. J. "A Theory of Program Size Formally Identical to Information Theory." J. Assoc. Comput. Mach. 22, 329-340, 1975.
Chaitin, G. J. "How Much Information Can There be in a Real Number?" Int. J. Bifur. Chaos 17, 1933-1935, 2007.
Chaitin, G. Meta Math!:The Quest for Omega. New York: Pantheon Books, 2005.
Finch, S. R. "Chaitin's Constant." §1.11 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 81-83, 2003.
Gardner, M. "The Random Number  Bids Fair to Hold the Mysteries of the Universe." Sci. Amer. 241, 20-34, Nov. 1979.
Bids Fair to Hold the Mysteries of the Universe." Sci. Amer. 241, 20-34, Nov. 1979.
Gardner, M. "Chaitin's Omega." Ch. 21 in Fractal Music, Hypercards, and More Mathematical Recreations from Scientific American Magazine. New York: W. H. Freeman, pp. 307-319, 1992.
Kobayashi, K. "Sigma(N)O-Complete Properties of Programs and Lartin-Lof Randomness." Information Proc. Let. 46, 37-42, 1993.
Sloane, N. J. A. Sequences A079365 and A100264 in "The On-Line Encyclopedia of Integer Sequences."
Solovay, R. M. "A Version of  for Which ZFC Cannot Predict a Single Bit." In Finite Versus Infinite. Contributions to an Eternal Dilemma (Ed. C. Calude and G. Păun). London: Springer-Verlag, pp. 323-334, 2000.
for Which ZFC Cannot Predict a Single Bit." In Finite Versus Infinite. Contributions to an Eternal Dilemma (Ed. C. Calude and G. Păun). London: Springer-Verlag, pp. 323-334, 2000.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















