


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 13-12-2020
Date: 10-9-2020
Date: 1-8-2020
|
A zerofree number 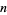 is called right truncatable if
is called right truncatable if  and all numbers obtained by successively removing the rightmost digits are prime. There are exactly 83 right truncatable primes in base 10. The first few are 2, 3, 5, 7, 23, 29, 31, 37, 53, 59, 71, 73, 79, 233, 239, 293, 311, 313, 317, 373, 379, 593, 599, ... (OEIS A024770), the largest being the 8-digit number
and all numbers obtained by successively removing the rightmost digits are prime. There are exactly 83 right truncatable primes in base 10. The first few are 2, 3, 5, 7, 23, 29, 31, 37, 53, 59, 71, 73, 79, 233, 239, 293, 311, 313, 317, 373, 379, 593, 599, ... (OEIS A024770), the largest being the 8-digit number 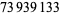 (Angell and Godwin 1977). The numbers of
(Angell and Godwin 1977). The numbers of 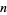 -digit right prime strings for
-digit right prime strings for 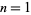 , 2, ..., 8 are 4, 9, 14, 16, 15, 12, 8, and 5 (OEIS A050986; Rivera puzzle 70).
, 2, ..., 8 are 4, 9, 14, 16, 15, 12, 8, and 5 (OEIS A050986; Rivera puzzle 70).
Similarly, call a number  left truncatable if
left truncatable if  and all numbers obtained by successively removing the leftmost digit are prime. There are exactly 4260 left truncatable primes in base 10 when the digit zero is not allowed. The first few are 2, 3, 5, 7, 13, 17, 23, 37, 43, 47, 53, 67, 73, 83, 97, 113, 137, 167, 173, ... (OEIS A024785), with the largest being the 24-digit number
and all numbers obtained by successively removing the leftmost digit are prime. There are exactly 4260 left truncatable primes in base 10 when the digit zero is not allowed. The first few are 2, 3, 5, 7, 13, 17, 23, 37, 43, 47, 53, 67, 73, 83, 97, 113, 137, 167, 173, ... (OEIS A024785), with the largest being the 24-digit number 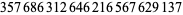 (Angell and Godwin 1977). The numbers of
(Angell and Godwin 1977). The numbers of 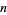 -digit left truncatable primes for
-digit left truncatable primes for 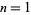 , 2, ... 24 are 4, 11, 39, 99, 192, 326, 429, 521, 545, 517, 448, 354, 276, 212, 117, 72, 42, 24, 13, 6, 5, 4, 3, and 1 (OEIS A050987; Rivera puzzle 70).
, 2, ... 24 are 4, 11, 39, 99, 192, 326, 429, 521, 545, 517, 448, 354, 276, 212, 117, 72, 42, 24, 13, 6, 5, 4, 3, and 1 (OEIS A050987; Rivera puzzle 70).
If zeros are permitted, the sequence of left truncatable primes is infinite, and the first few terms are 2, 3, 5, 7, 13, 17, 23, 37, 43, 47, 53, 67, 73, 83, 97, 103, 107, 113, 137, 167, 173, 197, 223, 283, 307, ... (OEIS A033664).
J. Shallit has shown that in base 10, there is a finite, minimal list of primes that do not have any other primes as substrings (where digits do not need to be consecutive). This result is a special case of a much more general theorem, whose proof is unfortunately nonconstructive.
Call an 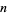 -digit prime
-digit prime 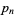 (with
(with 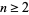 ) is a restricted left truncatable prime if
) is a restricted left truncatable prime if
1. If the leftmost digit of 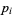 is deleted, a prime number
is deleted, a prime number 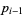 is obtained for
is obtained for 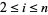 , and
, and
2. No prime with 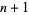 digits can have its leftmost digit removed to produce
digits can have its leftmost digit removed to produce 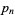 .
.
Kahan and Weintraub (1998) dub such primes "Henry VIII primes." Restricted left truncatable primes 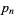 are therefore a subset of left truncatable primes for which there are no left truncatable primes of length
are therefore a subset of left truncatable primes for which there are no left truncatable primes of length  having the same
having the same 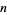 last digits as
last digits as 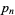 . There are a total of 1440 such primes, and the first few are 773, 3373, 3947, 4643, 5113, 6397, 6967, 7937, ... (OEIS A055521), the largest being 357686312646216567629137 (Angell and Godwin 1977, Kahan and Weintraub 1998).
. There are a total of 1440 such primes, and the first few are 773, 3373, 3947, 4643, 5113, 6397, 6967, 7937, ... (OEIS A055521), the largest being 357686312646216567629137 (Angell and Godwin 1977, Kahan and Weintraub 1998).
Truncatable primes are also called Russian doll primes.
REFERENCES:
Angell, I. O. and Godwin, H. J. "On Truncatable Primes." Math. Comput. 31, 265-267, 1977.
De Geest, P. "List of the 4260 Left-Truncatable Primes (without the Zero Digit)." https://www.worldofnumbers.com/truncat.htm.
Kahan, S. and Weintraub, S. "Left Truncatable Primes." J. Recr. Math. 29, 254-264, 1998.
Rivera, C. "Problems & Puzzles: Puzzle 002-Prime Strings." https://www.primepuzzles.net/puzzles/puzz_002.htm.
Rivera, C. "Problems & Puzzles: Puzzle 070-Primes Double Tree (A Puzzle Suggested by Paul Leyland)." https://www.primepuzzles.net/puzzles/puzz_070.htm.
Schroeppel, R. Item 33 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, p. 14, Feb. 1972. https://www.inwap.com/pdp10/hbaker/hakmem/number.html#item33.
Sloane, N. J. A. Sequences A024770, A024785, A032437, A033664, A050986, A050987, and A055521 in "The On-Line Encyclopedia of Integer Sequences."



|
|
|
|
دراسة تكشف "مفاجأة" غير سارة تتعلق ببدائل السكر
|
|
|
|
|
|
|
أدوات لا تتركها أبدًا في سيارتك خلال الصيف!
|
|
|
|
|
|
|
العتبة العباسية المقدسة تؤكد الحاجة لفنّ الخطابة في مواجهة تأثيرات الخطابات الإعلامية المعاصرة
|
|
|