
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
Mass and Energy
المؤلف:
Roger J Blin-Stoyle, FRS
المصدر:
Physics of Particles, Matter and the Universe
الجزء والصفحة:
p 111
24-5-2016
2814
Mass and Energy
The two postulates of relativity and the discussion of some of their implications leads on to a remarkable relationship between energy and mass. Saw that when a force is applied to an object its motion in the direction of the force accelerates and it gains an amount of kinetic energy equal to the work done by the force; energy is conserved. Continuing to exert the force, the speed of the object steadily increases and classically there is no limit to the speed that can be attained. However, this is not the case with relativity theory; the maximum attainable speed is c, the speed of light. As the speed approaches c it becomes harder to accelerate the object. Yet, if energy is conserved, the work being done by the force must in some way be increasing the energy of the object. This energy is dependent on only two quantities the object’s mass and its speed. If the latter cannot readily increase in response to the force it follows that the former must somehow be increasing and the nearer the speed becomes to c the larger will be this effect. We thus come to the remarkable conclusion that the mass of an object increases as its speed increases, becoming very large indeed (heading for infinity) as this speed approaches the speed of light. Clearly the lowest value this mass can have is when the object is at rest and this value is referred to as the object’s rest muss (denoted by mo). The increase in the mass of an object due to its motion is proportional to its rest mass and it therefore follows that the only circumstance in which there is no increase in mass is if the rest mass is itself zero. This is the situation with a photon and that is why, to state the tautologically obvious, it does travel with the speed of light. Other elementary particles that are also believed to have zero rest mass and which also, therefore, always travel at the speed of light. Further, since energy (provided by the work done by the force) is being transformed into mass, it follows that there must be an intimate relationship between these two physical quantities. It turns out that this relationship is extremely simple and is embodied in probably the best known of all physical formulae, namely
energy = (mass) × (speed of light)2
or, more succinctly,
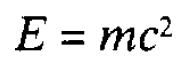
where E represents energy and m mass. In principle, then, energy can be converted into mass and mass into energy. This being so, means that it is no longer sensible in the relativistic context to talk about the ‘conservation of energy’ since clearly energy and mass are equivalent. Rather we have to employ a more embracing law the conservation of mass energy. All this being said does not imply that our considerations in earlier chapters about motion, energy and the behaviour of matter in the everyday world are invalidated. To be sure they are not exactly right but, since the speeds involved are exceedingly small compared with the speed of light, any corrections to our conclusions are absolutely negligible. Reverting back to the moving object we have been discussing, its momentum ( p ) is simply given by the classical expression mass × velocity except that, instead of using what we have now called rest mass (m0) for the mass of the object, the relativistic mass (m) must be used. In these terms, a very simple relationship for the total energy (E) of an object, including its rest-mass energy, can then be obtained. It is
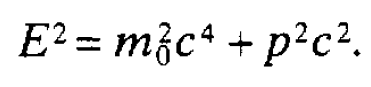
Thus, for a particle at rest (p = 0) the energy contained within its mass is

At the other extreme, for an object of zero rest mass (m0 = 0), such as a photon, the relation between energy and momentum is simply
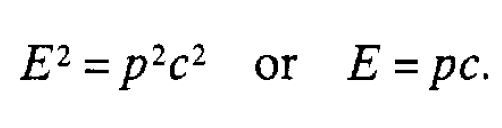
Since for a photon E = hv where U is its frequency and h is Planck’s constant, we have

Then, using the relation between frequency, wavelength and wave speed the expression relating wavelength, momentum and h used is obtained, namely

Since the speed of light is so large, the mass energy relationship does imply that if a small amount of mass could be converted into energy the amount released would be extremely large indeed. To put this into proportion, 1g of matter completely converted into energy could run a one-bar electric fire 24h a day for a year in around 3000 homes. Unfortunately, it is not possible to completely annihilate a piece of matter and convert all of its mass into energy. This can only be done with relatively small quantities. Even so, when this is arranged the energy release can still be very large witness nuclear explosions brought about by fission or fusion.
 الاكثر قراءة في الميكانيك
الاكثر قراءة في الميكانيك
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















