Force Law for SHM

Now consider Newton's law for a Spring where the force is given by F = -kx (Hooke's law), where k is called the spring constant. Substituting into

   F = ma

  -kx = ma

but we found that  giving

or

which is the angular frequency for an oscillating spring. The period is obtained from  or

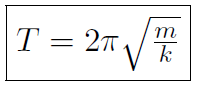
Notice an amazing thing. The period does not depend on the amplitude of oscillation xm! When a spring is oscillating, the oscillations tend to die down in amplitude xm but the period of oscillation remains the same! This is crucial to the operation of clocks. I can ''wind" my spring clock by just pulling on it a bit and still the period is the same.

Navigation and Clocks

NNN - FIX For a pendulum, this independence of the period on the amplitude was first noticed by Galileo and led to the development of clocks which was very important for navigation. The reason was that it enabled one to determine longitude on Earth. (Latitude was easy to determine just by measuring the height of the Sun in the sky at noon.) By dragging knotted ropes behind a ship it was easy to measure the speed of a ship. If one knew how long one had been travelling (i.e. measure the time of travel, say with a pendulum or spring clock) then one knew the distance from the port from which one had set sail. Knowing longitude and latitude gives one's position on the Earth. Thus the invention of accurate clocks (based on the independence of period and amplitude) enabled accurate estimates of longitude and thus revolutionized navigation.

Example F = ma is really a differential equation, that is an equation involving derivatives. For the spring, it becomes  where  . Thus the differential equation is

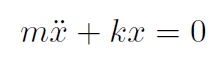
In mathematics there are special techniques for solving differential equations, which you will learn about in a special differential equations course. Using these special techniques one can prove that x = x_m cos t is a solution to the above differential equation. (Just like the solution to the algebraic equation x² - 5 = 4 is x = ±3. We verify this solution by sustituting, (±3)2 -5 = 9-5 = 4). Many students will not have yet learned how to solve differential equations, but we can verify that the solution given is correct. Verify that x = x_m cos t is a solution to the differential equation

Solution

Substitute into

giving

or

Thus if

then x = x_m cos t is a solution.

Example When a mass is suspended from the end of a massless spring, the spring stretches by a distance x. If the spring and mass are then put into oscillation, what is the period ?

Solution We saw that the period is given by . We don't know m or k ! We can get k from Hooke's law F = -kx. The weight W = mg stretches the spring, thus mg = kx or . Thus

and fortunately m cancels out giving

مواضيع ذات صلة

أنظمة لأكثر من جسيم واحد

كمية التحرك الزاوية والقوة المركزية

الحركة الدورانية

امثلة عن الاتزان الاستاتيكي للأجسام الممتدة

الاتزان الاستاتيكي للأجسام الممتدة

تعريف العزم

تذبذبات صغيرة لبندول

اعتبارات الطاقة في الحركة التوافقية البسيطة

الحل الهندسي للمعادلة التفاضلية للحركة التوافقية البسيطة، دائرة المرجع

الحل باستخدام حساب التفاضل والتكامل

قانون هوك والمعادلة التفاضلية للحركة التوافقية البسيطة

القدرة ووحدات الشغل