
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
Divergence
المؤلف:
Richard Fitzpatrick
المصدر:
Classical Electromagnetism
الجزء والصفحة:
p 33
16-7-2017
2885
Divergence
Let us start with a vector field A. Consider  A . dS over some closed surface S, where dS denotes an outward pointing surface element. This surface integral is usually called H the flux of A out of S. If A is the velocity of some fluid, then
A . dS over some closed surface S, where dS denotes an outward pointing surface element. This surface integral is usually called H the flux of A out of S. If A is the velocity of some fluid, then  A . dS is the rate of flow of material out of S. If A is constant in space then it is easily demonstrated that the net flux out of S is zero:
A . dS is the rate of flow of material out of S. If A is constant in space then it is easily demonstrated that the net flux out of S is zero:
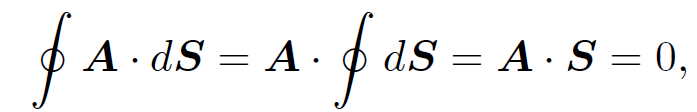 (1.1)
(1.1)
since the vector area S of a closed surface is zero. Suppose, now, that A is not uniform in space. Consider a very small rectangular volume over which A hardly varies. The contribution to  A . dS from the two faces normal to the x-axis is
A . dS from the two faces normal to the x-axis is
 (1.2)
(1.2)
where dV = dx dy dz is the volume element. There are analogous contributions
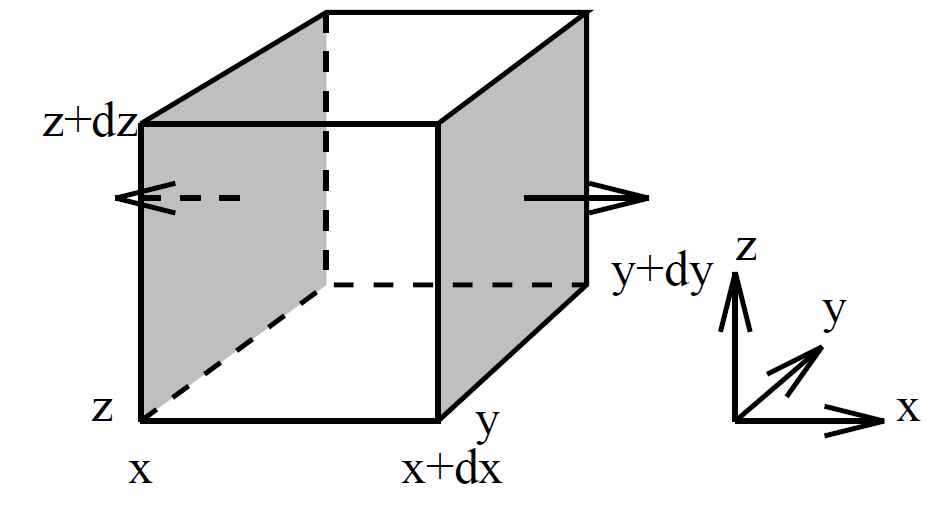
from the sides normal to the y and z-axes, so the total of all the contributions is
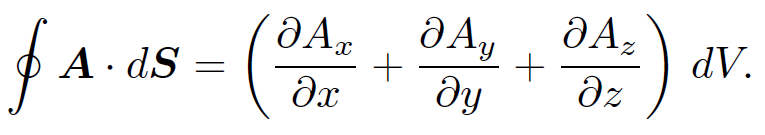 (1.3)
(1.3)
The divergence of a vector field is defined
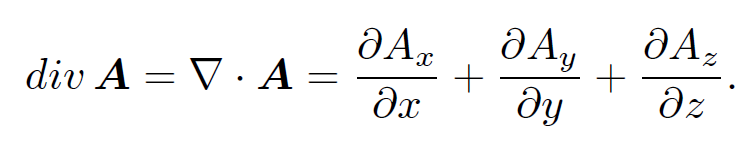 (1.4)
(1.4)
Divergence is a good scalar (i.e., it is coordinate independent), since it is the dot product of the vector operator ∇ with A. The formal definition of divA is
 (1.5)
(1.5)
This definition is independent of the shape of the infinitesimal volume element. One of the most important results in vector field theory is the so-called divergence theorem or Gauss' theorem. This states that for any volume V surrounded by a closed surface S,
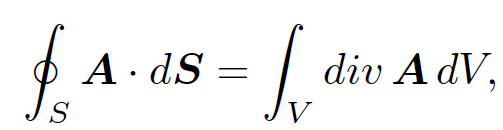 (1.6)
(1.6)
where dS is an outward pointing volume element. The proof is very straightforward.
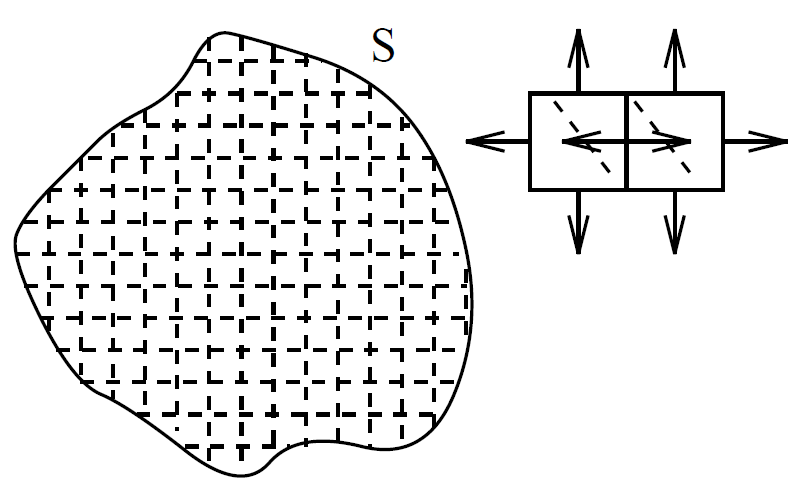
We divide up the volume into lots of very small cubes and sum ∫A . dS over all of the surfaces. The contributions from the interior surfaces cancel out, leaving just the contribution from the outer surface. We can use Eq. (1.3) for each cube individually. This tells us that the summation is equivalent to ∫div A dV over the whole volume. Thus, the integral of A . dS over the outer surface is equal to the integral of divA over the whole volume, which proves the divergence theorem. Now, for a vector field with div A = 0,
 (1.7)
(1.7)
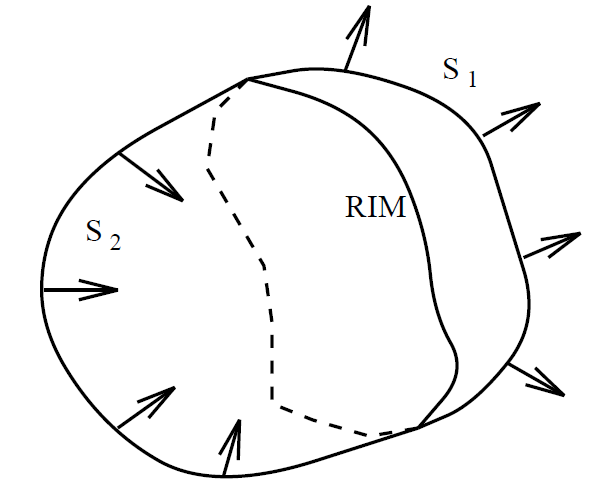
for any closed surface S. So, for two surfaces on the same rim,
 (1.8)
(1.8)
Thus, if div A = 0 then the surface integral depends on the rim but not the nature of the surface which spans it. On the other hand, if div A ≠ 0 then the integral depends on both the rim and the surface.
Consider an incompressible fluid whose velocity field is v. It is clear that 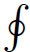 v . dS = 0 for any closed surface, since what flows into the surface must flow out again. Thus, according to the divergence theorem, ∫div v dV = 0 for any volume. The only way in which this is possible is if div v is everywhere zero. Thus, the velocity components of an incompressible fluid satisfy the following differential relation:
v . dS = 0 for any closed surface, since what flows into the surface must flow out again. Thus, according to the divergence theorem, ∫div v dV = 0 for any volume. The only way in which this is possible is if div v is everywhere zero. Thus, the velocity components of an incompressible fluid satisfy the following differential relation:
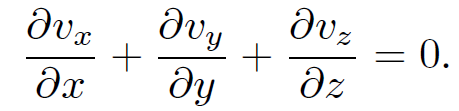 (1.9)
(1.9)
Consider, now, a compressible fluid of density ρ and velocity v. The surface integral  is the net rate of mass flow out of the closed surface S. This must be equal to the rate of decrease of mass inside the volume V enclosed by S, which is written -( ∂/∂t)(∫V ρ dV ). Thus,
is the net rate of mass flow out of the closed surface S. This must be equal to the rate of decrease of mass inside the volume V enclosed by S, which is written -( ∂/∂t)(∫V ρ dV ). Thus,
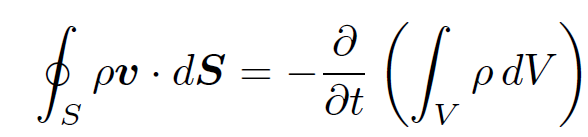 (1.10)
(1.10)
for any volume. It follows from the divergence theorem that
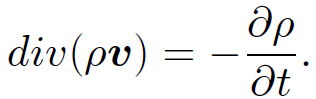 (1.11)
(1.11)
This is called the equation of continuity of the fluid, since it ensures that fluid is neither created nor destroyed as it flows from place to place. If ρ is constant then the equation of continuity reduces to the previous incompressible result div v = 0. It is sometimes helpful to represent a vector field A by ''lines of force" or ''field lines". The direction of a line of force at any point is the same as the direction of A. The density of lines (i.e., the number of lines crossing a unit surface perpendicular to A) is equal to |A|. In the diagram, |A| is larger at point

1 than at point 2. The number of lines crossing a surface element dS is A . dS. So, the net number of lines leaving a closed surface is
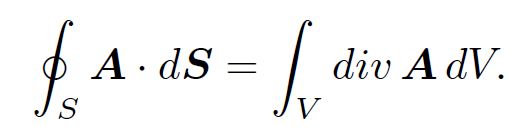 (1.12)
(1.12)
If divA = 0 then there is no net flux of lines out of any surface, which mean that the lines of force must form closed loops. Such a field is called a solenoidal vector field.
 الاكثر قراءة في الفيزياء العامة
الاكثر قراءة في الفيزياء العامة
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















