Repeated Wave Forms

Before we apply the Fourier transform capability of MacScope to the analysis of experimental data, there is one more feature of the analysis we need to discuss. What we are doing with the program is reconstructing a selected section of a waveform from harmonic sine waves. Anything we build from harmonic sine waves exactly repeats at the period of the first harmonic. Thus our reconstructed wave will always be a repeating wave, beginning again at the same height as the beginning of the previous cycle.
You can most easily see what we mean if you select a non repeating section of a wave. In Figure (1) we have gone back to a sine wave, but selected one and a half cycles. In the FFT window you see a slew of harmonics. To see why these extra harmonics are present, we have in Figure (2) selected the first 9 of them and in the upper window see what they add up to. It is immediately clear what has gone wrong. The selected harmonics are trying to reconstruct a repeating version of our 1.5 cycle of the sine wave. The extra spurious harmonics are there to force the reconstructed wave to start and stop at the same height as required by a repeating wave. If you are analyzing a repeating wave form and select a section that repeats, then your harmonic reconstruction will be accurate, with no spurious harmonics. If

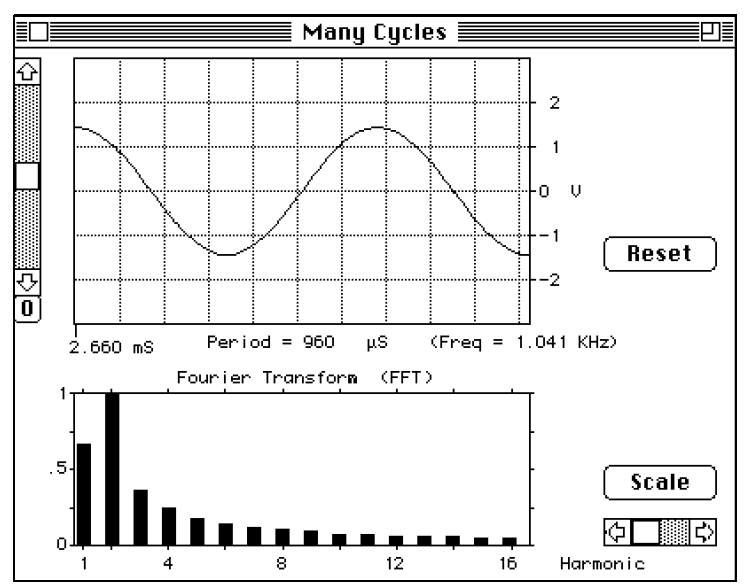
Figure 1: When we select one and a half cycles of a sine wave, we get a whole bunch of spurious harmonics.

your data is not repeating then you have to deal one way or another with this problem. One technique often used by engineers is to select a long section of data and smoothly force the ends of the data to zero so that the selected data can be treated as repeating data. Hopefully, forcing the ends of the data to zero does not destroy the information you are interested in. You can often accomplish the same thing by throwing away higher harmonics, assuming that the lower harmonics contain the interesting features of the data. You can see that neither of these techniques would work well for our one and a half cycles of a sine wave selected in Figure (2). In this text, our use of Fourier analysis will essentially be limited to the analysis of repeating waveforms. As long as we select a section that repeats, we do not have to worry about the spurious harmonics.

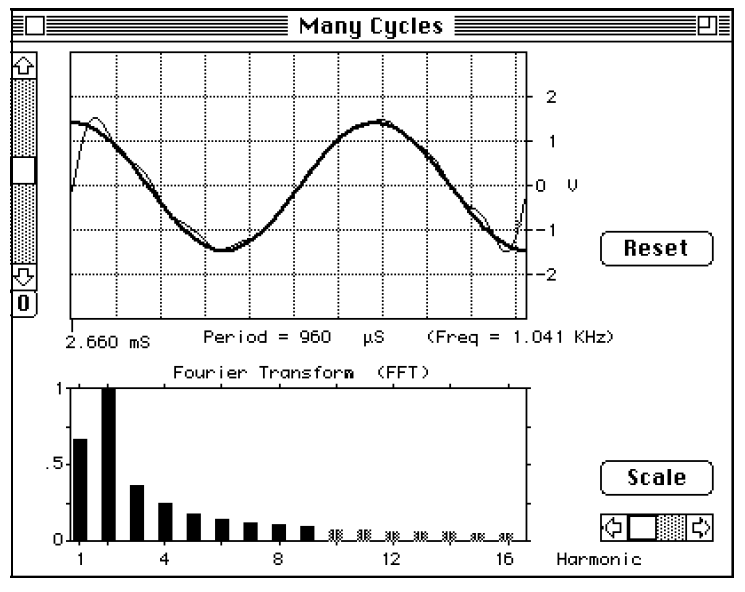
Figure 2: The spurious harmonics result from the fact that the reconstructed wave must be repeating—must start and stop at the same height.

مواضيع ذات صلة

أصول عدم الانعكاس

الانعكاس يولد الانعكاس

الاحتمالية في الميكانيكا الإحصائية

القابلية للانعكاس وعدم القابلية للانعكاس

نظرة في تعددية الفيزياء

الاختزال وعدم القابلية للانعكاس

الواقعية العلمية وتغيير النظريات

نقص الإثبات ومذهب الذرائعية ومذهب الواقعية

مشكلة التمييز: متى يكون المنهج علميا؟

المنهج العلمي: من الاستقراء إلى التكذيب وما بعده

مناهج العلوم وثمارها

!Parity is not conserved